A steady current is flowing in a circular coil of radius R, made up of a thin conducting wire. The magnetic field at the centre of the loop is . Now, a circular loop of radius is made from the same wire without changing its length, by unfounding and refolding the loop, and the same current is passed through it. If new magnetic field at the centre of the coil is , then the ratio is
none of these
The correct Answer is:A
To solve the problem, we need to find the ratio of the magnetic fields BL and BC produced by two different circular coils made from the same wire. Let's break down the solution step by step.
Step 1: Determine the magnetic field BL at the center of the original coil
The magnetic field at the center of a circular coil of radius R carrying a current I is given by the formula:
BL=μ0I2R
where μ0 is the permeability of free space.
Step 2: Determine the length of the wire used in the original coil
The circumference of the original coil is:
L=2πR
This length L is the length of the wire used to make the coil.
Step 3: Determine the radius of the new coil
The new coil is made from the same wire but has a radius of Rn.
Step 4: Calculate the number of turns in the new coil
The circumference of the new coil is:
L=2π(Rn)=2πRn
The number of turns n that can be made with the same length of wire is:
Number of turns=Length of wireCircumference of new coil=2πR2πRn=n
Step 5: Determine the magnetic field BC at the center of the new coil
The magnetic field at the center of a circular coil with n turns and radius Rn is given by:
BC=n⋅μ0I2(Rn)=n⋅μ0In2R=n2μ0I2R
Step 6: Calculate the ratio BLBC
Now we can find the ratio of the two magnetic fields:
BLBC=μ0I2Rn2μ0I2R=1n2
Final Result
Thus, the ratio BLBC is:
BLBC=1n2
The magnetic field at the center of a circular coil of radius R carrying a current I is given by the formula:
BL=μ0I2R
where μ0 is the permeability of free space.
The circumference of the original coil is:
L=2πR
This length L is the length of the wire used to make the coil.
The new coil is made from the same wire but has a radius of Rn.
The circumference of the new coil is:
L=2π(Rn)=2πRn
The number of turns n that can be made with the same length of wire is:
Number of turns=Length of wireCircumference of new coil=2πR2πRn=n
The magnetic field at the center of a circular coil with n turns and radius Rn is given by:
BC=n⋅μ0I2(Rn)=n⋅μ0In2R=n2μ0I2R
Now we can find the ratio of the two magnetic fields:
BLBC=μ0I2Rn2μ0I2R=1n2
Thus, the ratio BLBC is:
BLBC=1n2
Topper's Solved these Questions
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Exercise (multiple Currect )
5 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Exercise (assertion-reasioning )
2 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Exercise (subjective )
10 Videos
- RAY OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RAY OPTICSEXERCISE - DPP 1.6
12 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Comprehension Type
14 Videos
Similar Questions
A circular coil of n turns and radius r carries a current I. The magnetic field at the centre is
View SolutionA square loop of side a carris a current I. The magnetic field at the centre of the loop is
View SolutionA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is

View SolutionA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is :

View SolutionA current I flows along a triangle loop having sides of equal length a. The strength of magnetic field at the centre of the loop is :
View SolutionA square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is
View SolutionWhat will be magnetic field at centre of current carrying circular loop of radius R?
View SolutionA rectangular loop of metallic wire is of length a and breadth b and carries a current i. The magnetic field at the centre of the loop is
View SolutionA current I flows along a round circular loop of radius R. Find the line integration of magnetic field along the axis of the loop from center to ∞
View SolutionA current l flows through a closed loop as shown in figure .The magnetic field at the centre O is
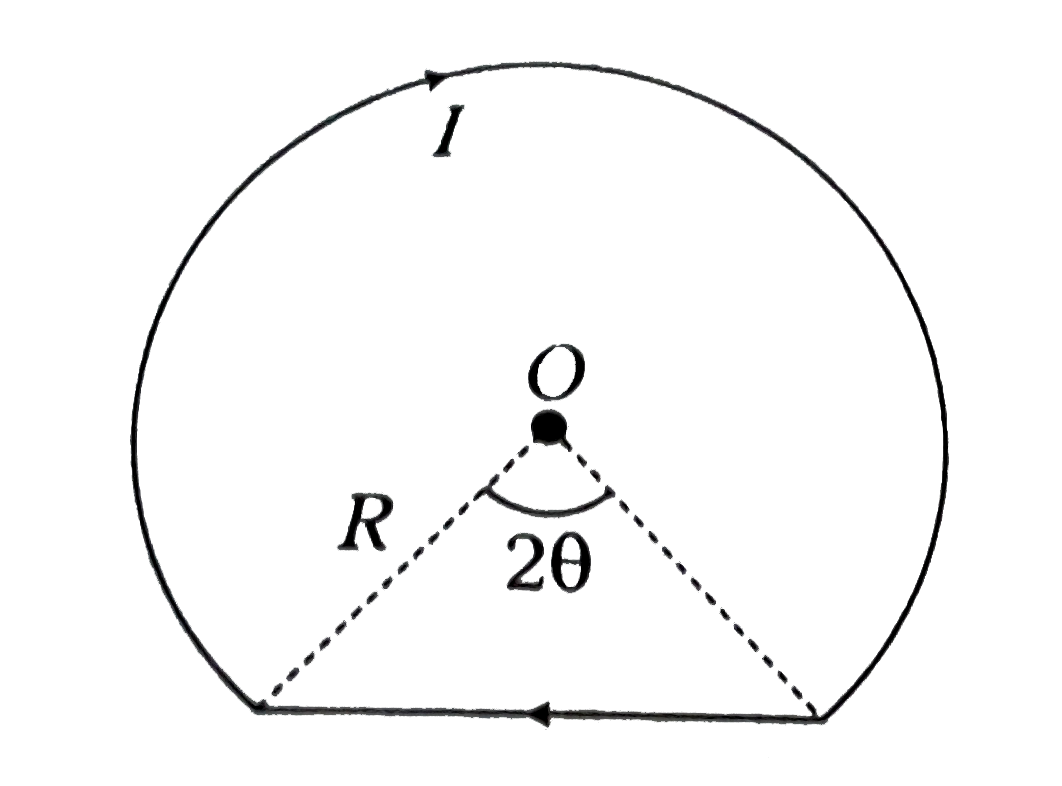
View Solution
