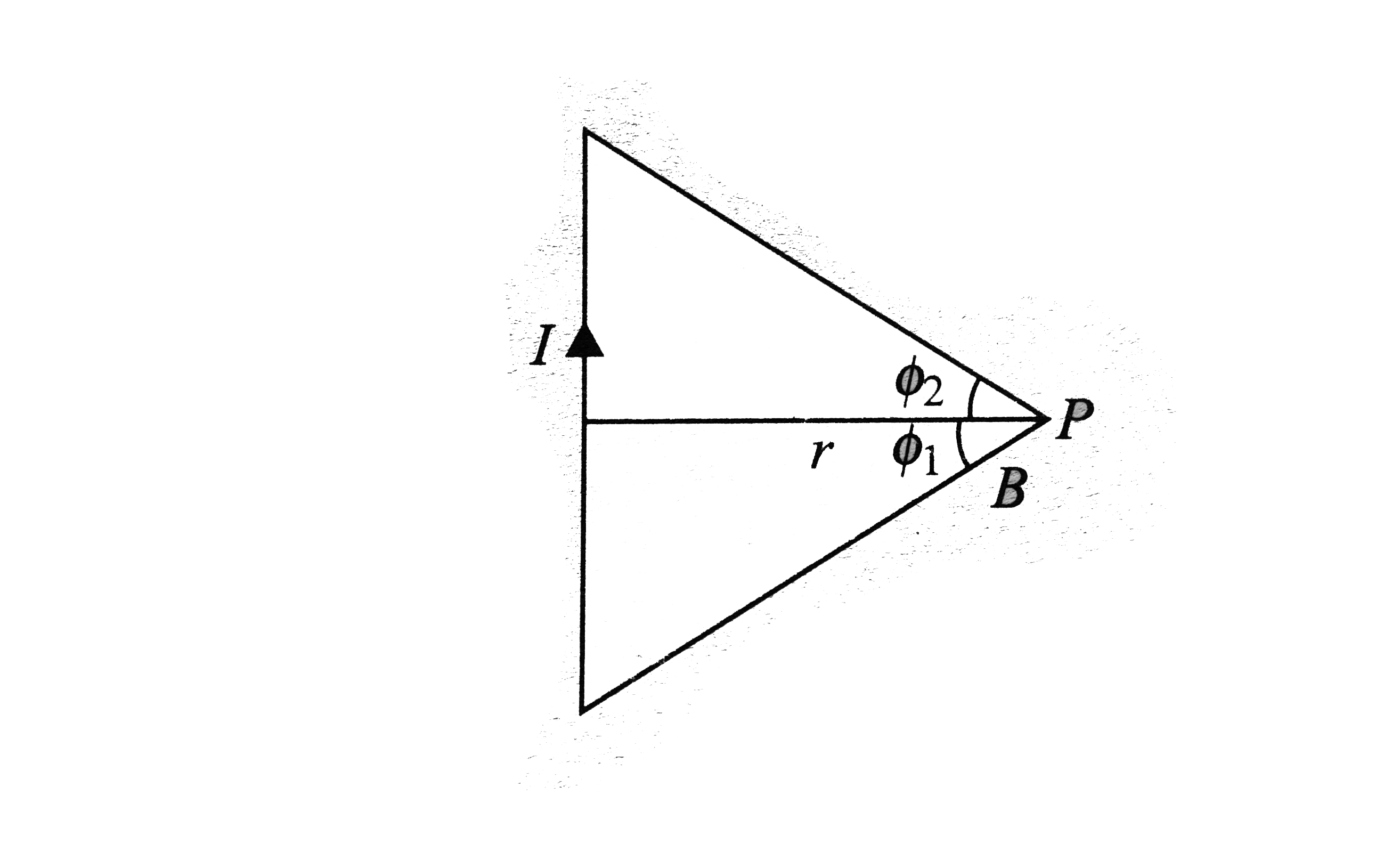
According to Biot-Savarat's law, magentic field due to a straight current carrying wire at a point at a distance r form it is given by
The direction of magnetic field being perpendicular to the plane containing the wire and the point.
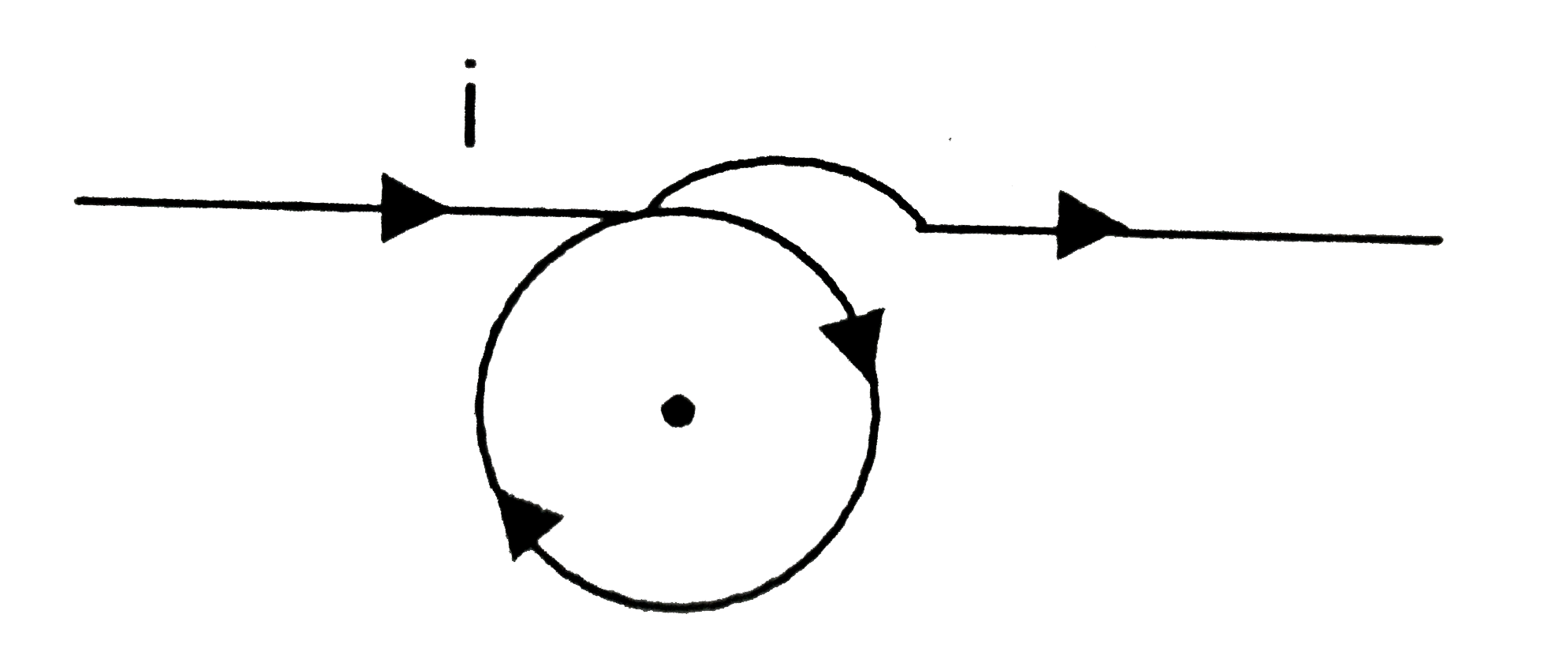
Figure, shows a closed loop AOCBA in which current I is flowing as shown. Given . Find the magnetic field at point B due to this loop.

The correct Answer is:C
(c)
At B, magnetic field will be zero due to CB and AB parts.
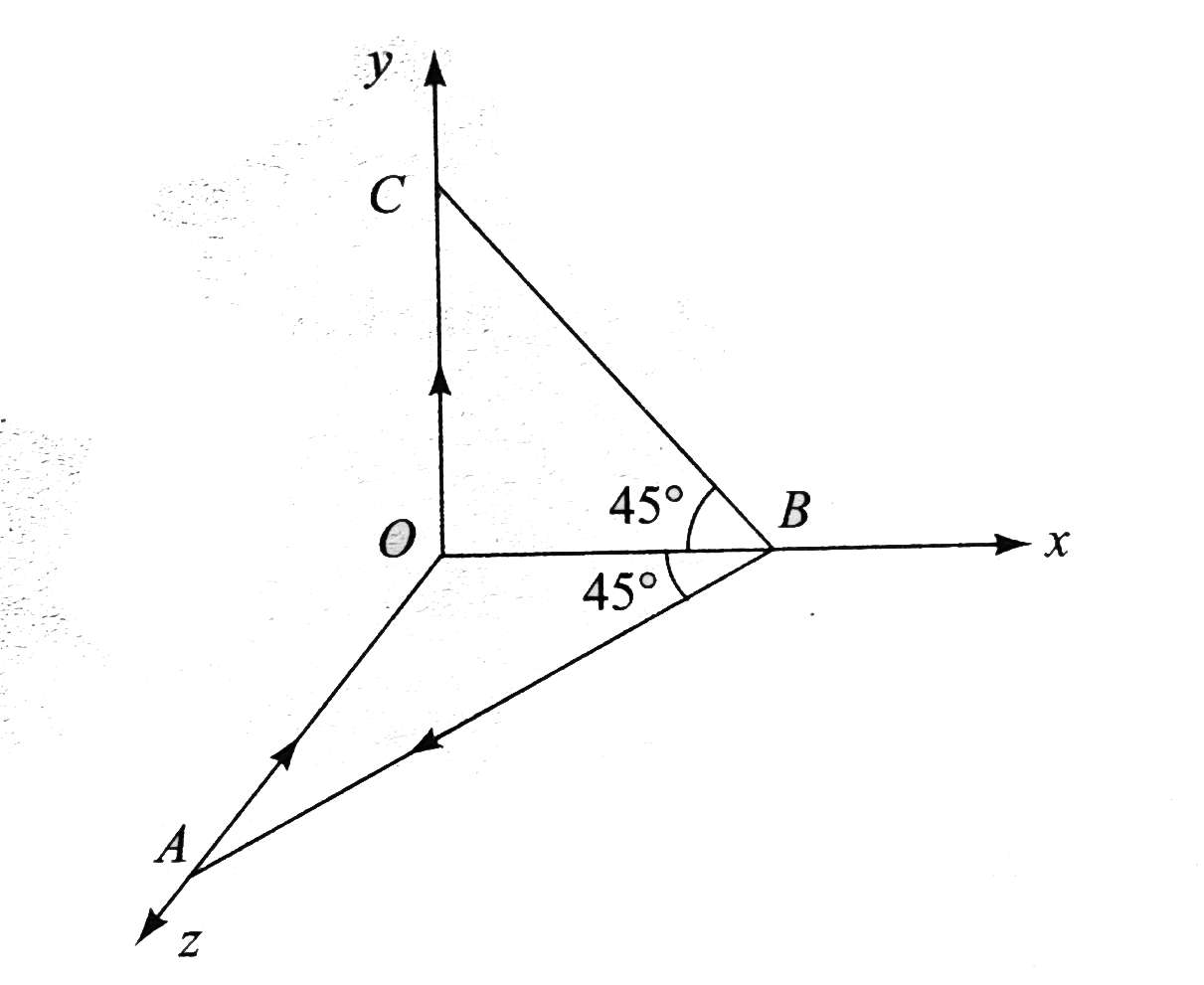
Net magnetic field at B
At O, magnetic field due to AO and OC will be zero.
Due. to BC:
Similarly, due to BA:
Net magnetic field
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Exercise (integer)7videos
SOURCES OF MAGNETIC FIELD
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Archives (fill In The Blanks)1videos
SOURCES OF MAGNETIC FIELD
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Exercise (assertion-reasioning )2videos
SOURCES OF MAGNETIC FIELD
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RAY OPTICSEXERCISE - DPP 1.612videos
RAY OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Comprehension Type14videos
WAVE OPTICS
Similar Questions
According to Biot-Savarat's law, magentic field due to a straight current carrying wire at a point at a distance r form it is given by
B=μ0I4πr(sinϕ1+sinϕ2)
The direction of magnetic field being perpendicular to the plane containing the wire and the point.
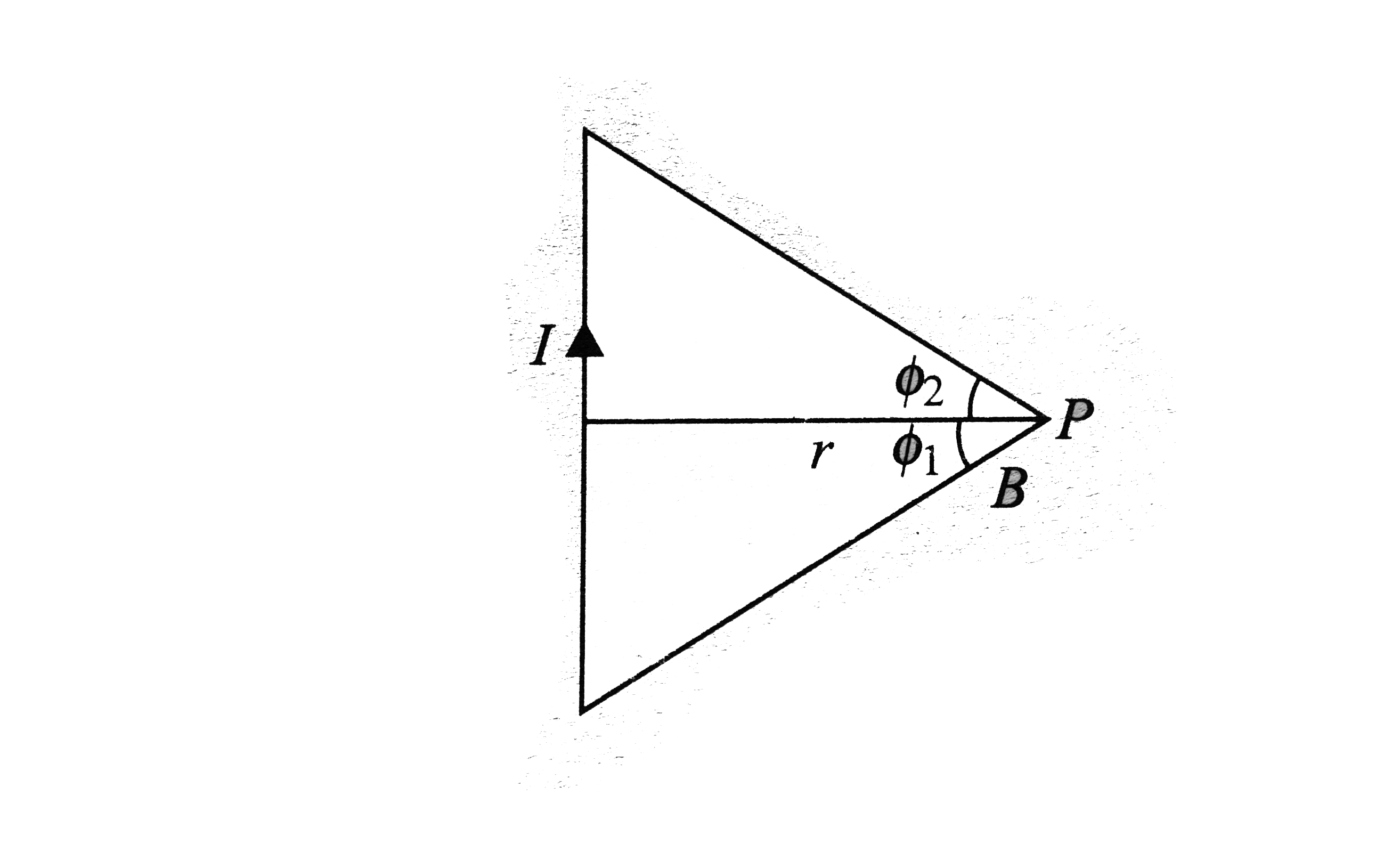
Find magnetic field at point O in Fig.View SolutionThe magnetic field in a straight current carrying conductor wire is:
View SolutionThe magnetic field at the origin due to the current flowing in the wire is
 View Solution
View SolutionThe magnetic field at the origin due to the current flowing in the wire is
 View Solution
View SolutionFind the magnitude and direction of magnetic field at point P due to the current carrying wire as shown in
 View Solution
View SolutionThe resulting magnetic field at the point O due to the current carrying wire shown in the figure:
 View Solution
View SolutionFind the direction of magnetic field at a point P due to two infinite current carrying wires shown in the figure below
 View Solution
View SolutionIn the given figure find out magnetic field at point B (Given : I=2.5A, r=5cm)
 View Solution
View SolutionTwo perpendicular infinite wires are carrying current i as shown in the figure. The magnetic field at point P is :
_2019_CBT2_E01_008_Q01.png) View Solution
View SolutionA long wire having a semi-circular loop of radius r carries a current I, as shown in Fig. Find the magnetic field due to entire wire.
 View Solution
View Solution

