In a series resonant circuit, the ac, voltage across resistance R, inductance L and capacitance are 5V, 10V and 10V, respectively. The ac. voltage applied to the circuit will be
20 V
10 V
5 V
25 V
The correct Answer is:C
To find the AC voltage applied to the series resonant circuit, we can follow these steps:
Step 1: Identify the given values
We have the following voltages across the components:
- Voltage across the resistor (Vr) = 5V
- Voltage across the inductor (Vl) = 10V
- Voltage across the capacitor (Vc) = 10V
Step 2: Understand the relationship in a series resonant circuit
In a series resonant circuit, the voltage across the inductor and the capacitor are equal in magnitude but opposite in phase. Therefore, they can be subtracted when calculating the total voltage applied to the circuit.
Step 3: Apply the formula for total voltage
The total voltage (V) applied to the circuit can be calculated using the formula:
V=√Vr2+(Vl−Vc)2
Since Vl=Vc, we can simplify the equation:
Vl−Vc=10V−10V=0
Thus, the equation simplifies to:
V=√Vr2+02
V=√Vr2
V=Vr
Step 4: Substitute the value of Vr
Now, substituting the value of Vr:
V=√(5V)2
V=5V
Step 5: Conclusion
The AC voltage applied to the circuit is 5V.
Final Answer
The AC voltage applied to the circuit is 5V.
---
We have the following voltages across the components:
- Voltage across the resistor (Vr) = 5V
- Voltage across the inductor (Vl) = 10V
- Voltage across the capacitor (Vc) = 10V
In a series resonant circuit, the voltage across the inductor and the capacitor are equal in magnitude but opposite in phase. Therefore, they can be subtracted when calculating the total voltage applied to the circuit.
The total voltage (V) applied to the circuit can be calculated using the formula:
V=√Vr2+(Vl−Vc)2
Since Vl=Vc, we can simplify the equation:
Vl−Vc=10V−10V=0
Thus, the equation simplifies to:
V=√Vr2+02
V=√Vr2
V=Vr
Now, substituting the value of Vr:
V=√(5V)2
V=5V
The AC voltage applied to the circuit is 5V.
The AC voltage applied to the circuit is 5V.
Similar Questions
In a series LCR circuit the rms voltage across the inductance , capacitance and resistance are respectively 4 V, 8 V and 5 V . The RMS voltage of the AC source in the circuit is
View SolutionIn a series L-C-R circuit the voltage across resistance , capacitance and inductance is 10 V each. If the capacitance is short circuited, the voltage across the inductance will be
View Solution
Knowledge Check
In a series LCR circuit the voltage across an inductor, capacitor and resistor are 20 V, 20 V and 40 V respectively. The phase difference between the applied voltage and the current in the circuit is
A30∘B45∘C60∘D0∘In a series LCR circuit, the voltage across the resistance, capacitance and inductance is 10 V each. If the capacitance is short circuited, the voltage across the inductance will be
A10VB10√2VC10√2VD20V
In an L-C-R circuit the AC voltage across R, L and C comes out as 10 V , 10 V and 20 V respectively . The voltage across the enter combination will be
View SolutionIn a series LCR circuit, the voltages across an inductor, a capacitor and a resistor are 30 V, 30 V, 60 V respectively. What is the phase difference between the applied voltage and the current in the circuit ?
View SolutionIn a series LCR circuit, the voltages across an inductor, a capacitor and a resistor are 30 V, 30 V, 60 V respectively. What is the phase difference between the applied voltage and the current in the circuit ?
View SolutionIn series LCR circuit voltage drop across resistance is 8V, across inductor is 6V and across capacitor is 12V. Then
View SolutionIn the given circuit the R.M.S values of voltages across the capacitor C, inductor L and resistor R1 are 12V, 10V and 5 V respectively. Then the peak voltage across R2 is
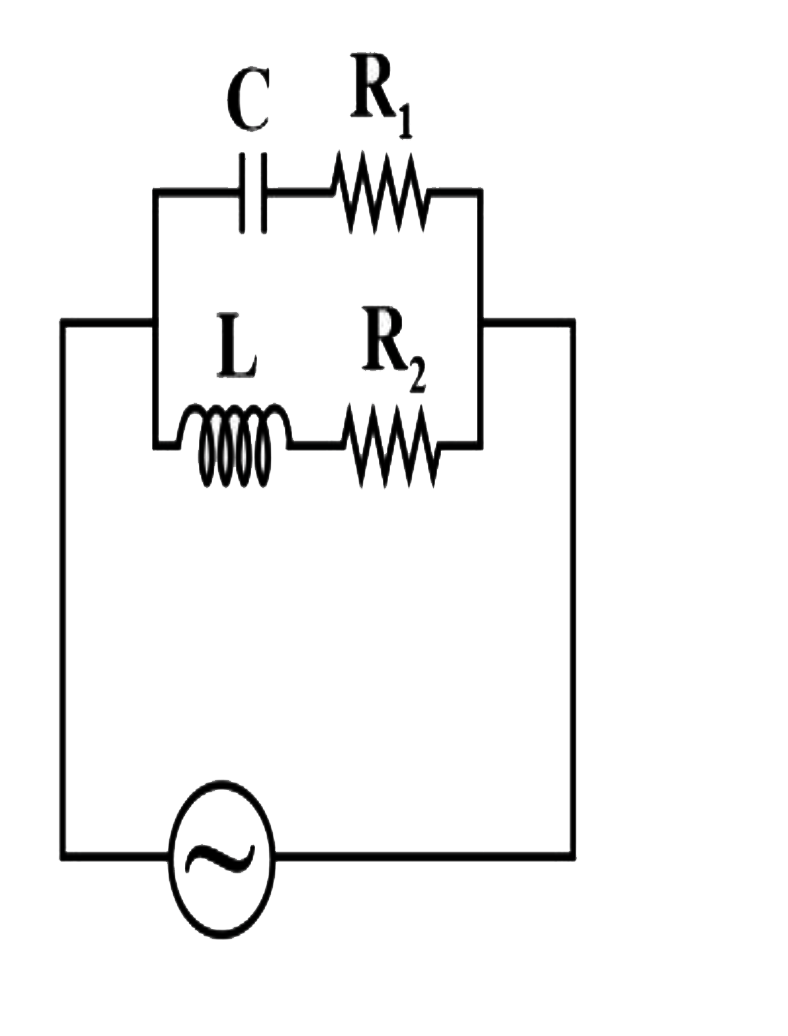
View SolutionThe potential differences across the resistance, capacitance and inductance are 80V,40V and 100V respectively in an L−C−R circuit. The power factor of this circuit is
View Solution
