A small quantity of a liquid which dows not mix with water sinks to the bottom at 20∘C, the densities of the liquid and water being 1021 and 990kg/m3, respectively. To what teperature must the mixture be uniformly heated in order that the liquid forms globules which just float on water ? the cubical expansion of the liquid and water over the temperature ranges is 85×10−5/K and 45×10−5/K respectively.
To solve the problem step by step, we will use the concept of density changes due to thermal expansion.
Step 1: Understand the problem
We have a liquid that sinks in water at 20°C. The densities of the liquid and water are given as 1021 kg/m³ and 990 kg/m³, respectively. We need to find the temperature at which the liquid will just float on water.
Step 2: Write the formula for density change
The density of a substance changes with temperature due to thermal expansion. The new density (d) can be expressed as:
d=d01+βΔT
where:
- d0 is the original density,
- β is the coefficient of cubical expansion,
- ΔT is the change in temperature.
Step 3: Set up the equations for the densities
For the liquid:
dliquid=10211+85×10−5ΔT
For water:
dwater=9901+45×10−5ΔT
Step 4: Set the condition for floating
For the liquid to just float on water, their densities must be equal:
10211+85×10−5ΔT=9901+45×10−5ΔT
Step 5: Cross-multiply to eliminate the fractions
Cross-multiplying gives:
1021(1+45×10−5ΔT)=990(1+85×10−5ΔT)
Step 6: Expand both sides
Expanding both sides:
1021+1021×45×10−5ΔT=990+990×85×10−5ΔT
Step 7: Rearrange the equation
Rearranging gives:
1021−990=990×85×10−5ΔT−1021×45×10−5ΔT
Step 8: Simplify the equation
This simplifies to:
31=(990×85−1021×45)×10−5ΔT
Step 9: Calculate the coefficients
Calculating the coefficients:
- 990×85=84150
- 1021×45=45945
Thus:
31=(84150−45945)×10−5ΔT
31=38205×10−5ΔT
Step 10: Solve for ΔT
Now, solving for ΔT:
ΔT=3138205×10−5
ΔT=0.81(approximately)
Step 11: Find the final temperature
Since ΔT=T2−T1 and T1=20°C:
T2=20+0.81≈20.81°C
Step 12: Conclusion
Thus, the temperature to which the mixture must be uniformly heated in order for the liquid to just float on water is approximately 20.81°C.
---
We have a liquid that sinks in water at 20°C. The densities of the liquid and water are given as 1021 kg/m³ and 990 kg/m³, respectively. We need to find the temperature at which the liquid will just float on water.
The density of a substance changes with temperature due to thermal expansion. The new density (d) can be expressed as:
d=d01+βΔT
where:
- d0 is the original density,
- β is the coefficient of cubical expansion,
- ΔT is the change in temperature.
For the liquid:
dliquid=10211+85×10−5ΔT
dwater=9901+45×10−5ΔT
For the liquid to just float on water, their densities must be equal:
10211+85×10−5ΔT=9901+45×10−5ΔT
Cross-multiplying gives:
1021(1+45×10−5ΔT)=990(1+85×10−5ΔT)
Expanding both sides:
1021+1021×45×10−5ΔT=990+990×85×10−5ΔT
Rearranging gives:
1021−990=990×85×10−5ΔT−1021×45×10−5ΔT
This simplifies to:
31=(990×85−1021×45)×10−5ΔT
Calculating the coefficients:
- 990×85=84150
- 1021×45=45945
31=(84150−45945)×10−5ΔT
31=38205×10−5ΔT
Now, solving for ΔT:
ΔT=3138205×10−5
ΔT=0.81(approximately)
Since ΔT=T2−T1 and T1=20°C:
T2=20+0.81≈20.81°C
Thus, the temperature to which the mixture must be uniformly heated in order for the liquid to just float on water is approximately 20.81°C.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Exercise 1.123videos
CALORIMETRY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Exercise 1.222videos
CALORIMETRY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.620videos
BASIC MATHEMATICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE1videos
CENTRE OF MASS
Similar Questions
A hemispherical bowl just floats without sinking in a liquid of density 1.2×103kg/m3. If outer diameter and the density of the bowl are 1m and 2×104kg/m3 respectively, then the inner diameter of bowl will be
View SolutionA hemispherical bowl just floats without sinking in a liquid of density 1.2×103kg/m3. If outer diameter and the density of the bowl are 1m and 2×104kg/m3 respectively, then the inner diameter of bowl will be
View SolutionIf the depth of a lake is 10 m, and the temperature of surroundings is −10∘C and that of water at the bottom of the lake is 5∘C. The thermal conductivity of the Ice and water are Kf and Kw respectively. The depth of ice will be
View SolutionA 250cm3 glass bottle is completely filled with water at 50∘C. The bottle and water are heated to 60∘C. How much water runs over if:
a. the expansion of the bottle is neglected:
b. the expansion of the bottle is included? Given the coefficient of areal expansion of glass β=1.2×10−5/K and γwater=60×10−5/∘C.View SolutionA tank contains water on top of mercury . A cube of iron, 60mm along each edge, is sitting upright in equilibrium in the liquids. Find how much of it is in each liquid. The densities of iron and mercury are 7.7×103kg/m3 and 13.6×103kg/m3 respectively
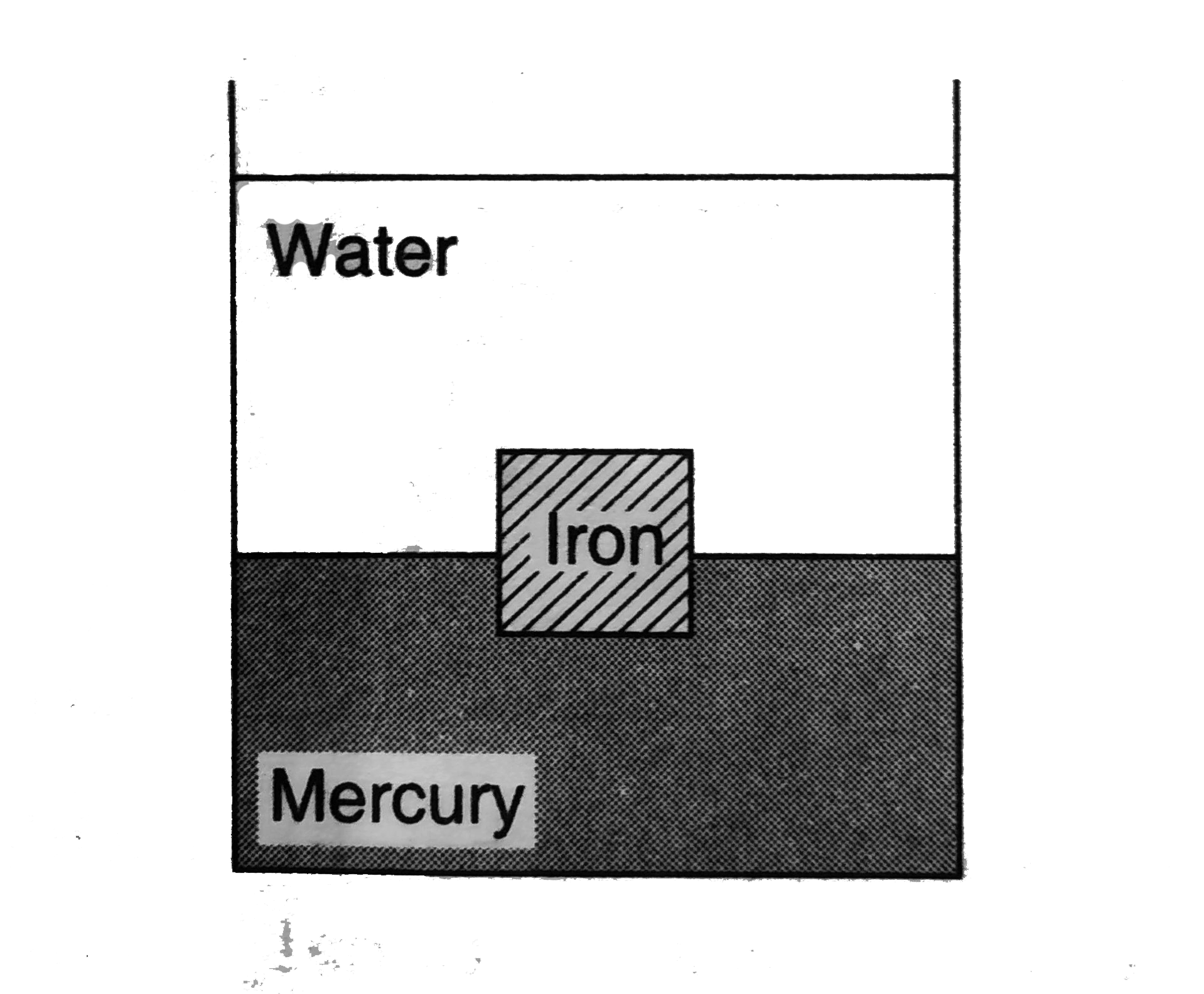 View Solution
View SolutionA tank contains water on top of mercury . A cube of iron, 60mm along each edge, is sitting upright in equilibrium in the liquids. Find how much of it is in each liquid. The densities of iron and mercury are 7.7×103kg/m3 and 13.6×103kg/m3 respectively
 View Solution
View SolutionThree liquids A, B and C having same sepcific heats have masses m,2m and 3m. Their temperaures are 0,20 and 3θ respectively. ltBrgt Q. What is the temperature of mixture, when Aand C all are mixed?
View SolutionThe densities of wood and benzene at 0∘C are 880kgm−3and900kgm−3 respectively. The coefficients of volume expansion are 1.2×10−3C−1 for wood and 1.5×10−3C−1 for benzene. At what temperature will a piece of wood just sink in benzene?
View SolutionThe surface tension and vapour pressure of water at 20∘C is 7.28×10−2Nm−1 and 2.33×103Pa, respectively. What is the radius of the smallest spherical water droplet which can form without evaporating at 20∘C?
View SolutionLiquids A and B form an ideal solution in the entire composition range. At 350K, the vapor pressure of pure A and pure B are 7×103 Pa and 12×103 Pa, respectively. The composition of the vapor in equilibrium with a solution containing 40 mole percent of A at this temperature is :
View Solution

