Two moles a monatomic gas in state A having critical pressure P0 and temperature 3T0 is taken to a state B having pressure 3P0 and temperature T0/3 by the process of equation P2T = constant. Then state B is taken to state C keeping the volume constant and it comes back to initial state A keeping temperature constant.
a. Plot a P and T graph. (P on the y-axis and T on the x-axis).
Find the net work done and heat supplied to the gas during the complete cycle.
Work done in process AB
ΔWAB=2r[T03−3T0](13−1)
W=−8RT0
ΔQAB=du+Δw=−nCvΔT−8RT0
ΔQAB=−16RT0
For process B→C,WBC=0
du=8RT0,ΔQBC=8RT0
and C→A (isothermal process)
ΔWCA=2R(3T0)1nVfVi(VfVi=27)
ΔWCA=18RT01n3
ΔQCA=18RT01n3
Net work done,
ΔW=ΔWAB+ΔWBC+ΔWCA
=−8RT0+0+18RT01n3
ΔW=RT0+(181n3−8)
ΔQnet=ΔQBC+ΔQCA
=−8RT0+18RT01n3
ΔQnet=RT0(181n3+8)

Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Single Correct140videos
KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Multiple Corrects29videos
KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Exercise 2.228videos
KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression2videos
KINETIC THEORY OF GASES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single correct anwer type14videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
Similar Questions
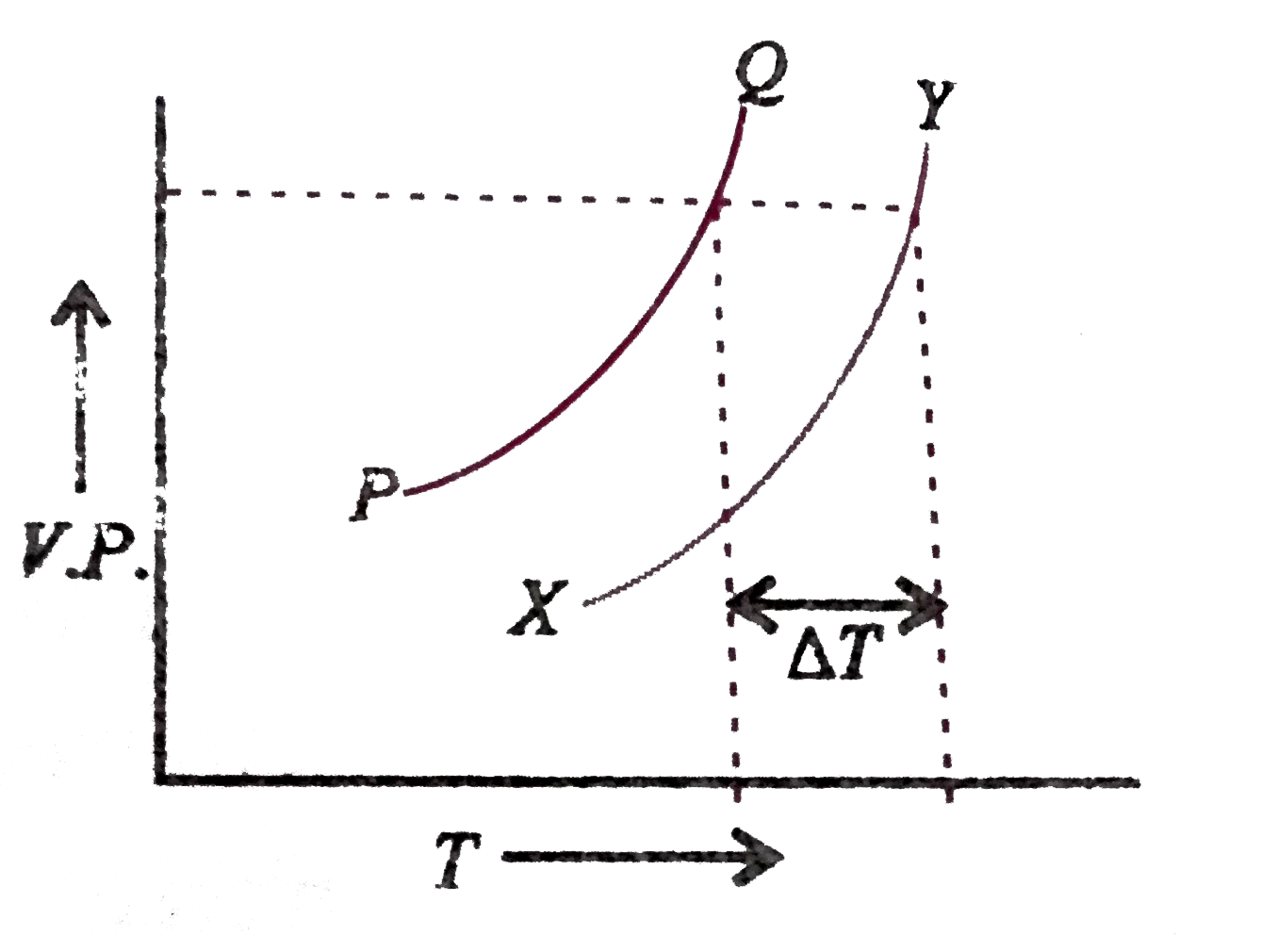
In the graph plotted between vapour pressure (V.P.) and temperature (T),
 View Solution
View SolutionThree moles of an ideal gas at a pressure PA and temperature TA is isothermally expanded to twice its initial volume. It is then compressed at constant pressure to its original , volume. Finally the gas is compressed at constant volume to its original pressure PA
(a) Sketch the P-V and P-T diagrams for the complete process.
(b) Calculate the net work done by the gas, and net heat supplied to the gas during the complete process.View Solution
Knowledge Check
- Question 1 - Select One
The equation of state for 5 g of oxygen at a pressure P and temperature T, when occupying a volume V, will be
APV=(532)RTBPV=5RTCPV=(52)RTDPV=(516)RT - Question 2 - Select One
The equation of state for 5 g of oxygen at a pressure P and temperature T, when occupying a volume V, wll be
APV =(5/32) TRBPV=5RTCPV=(5/2) RTDPV= (5/16) RT
Three moles of an ideal gas (Cp=72R) at pressure, PA and temperature TA is isothermally expanded to twice its initial volume. It is then compressed at constant pressure to its original volume. Finally gas is compressed at constant volume to its original pressure PA.
(a) Sketch P-V and P-T diagrams for the complete process.
(b) Calculate the net work done by the gas, and net heat supplied to the gas during the complete process.View SolutionThe equation of state for 5 g of oxygen at a pressure P and temperature T, when occupying a volume V, will be
View SolutionThe equation of state for 5 g of oxygen at a pressure P and temperature T, when occupying a volume V, will be
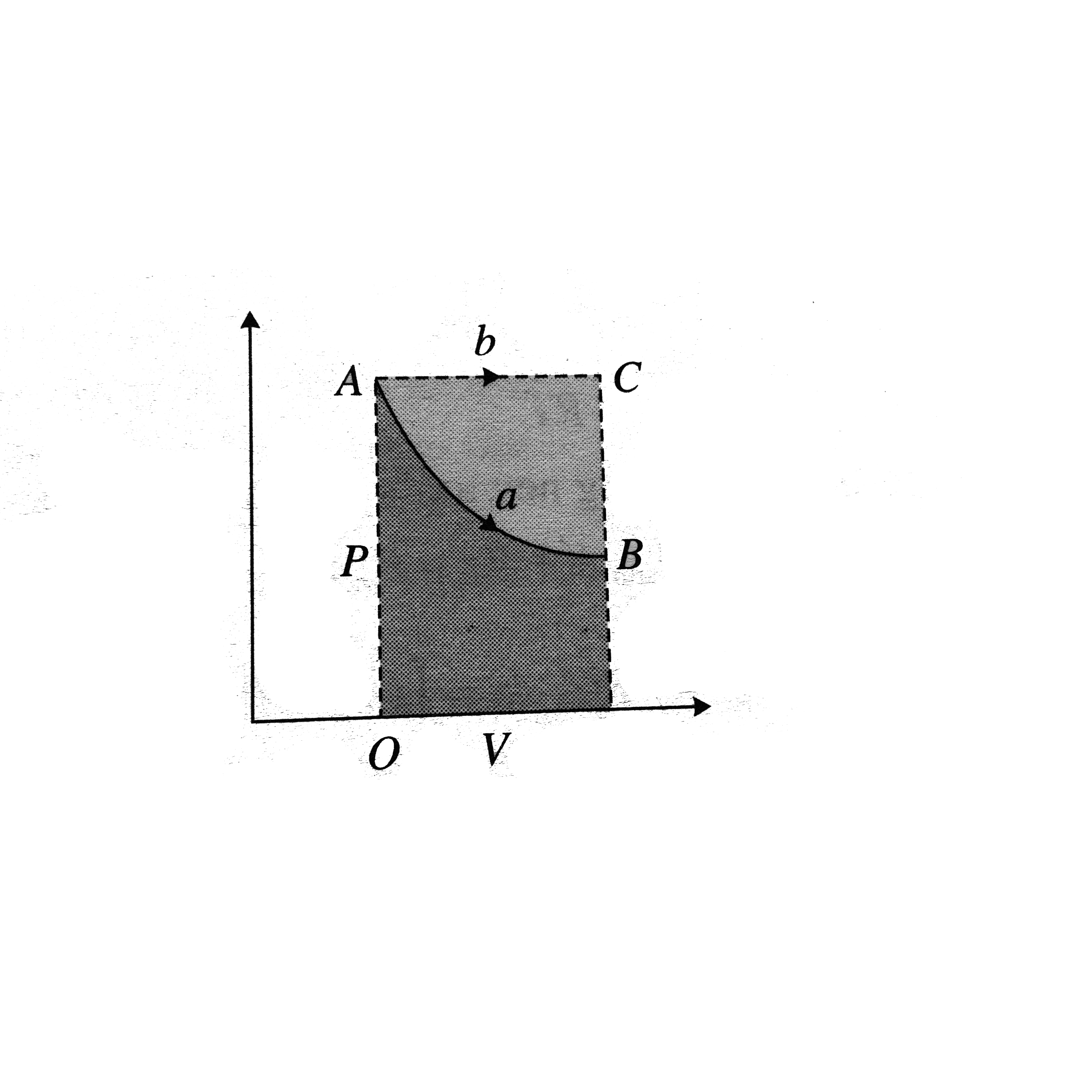
View SolutionA quantity of gas occupies an initial volume V0 at pressure p0 and temperature T0. It expands to a volume V (a) constant temperature and (b) constant pressure. In which case does the gas do more work ?
 View Solution
View SolutionAn ideal gas is found to obey the law V2T=constant. The initial pressure and temperature are P0 and T0. If gas expands such that its pressure becomes P04, then the final temperature of the gas will be
View SolutionFour moles of an ideal gas is initially in a state A having pressure 2×105N/m2 and temperature 200 K . Keeping pressure constant the gas is taken to state B at temperature of 400K. The gas is then taken to a state C in such a way that its temperature increases and volume decreases. Also from B to C, the magnitude of dTdV increases. The volume of gas at state C is eaual to its volume at state A. Now gas is taken is initial state A keeping volume constant. A total of 1000 J heat is rejected from the sample in the cyclic process. Take R=8.3J/K/mol.
The work done by the gas along path B to C isView Solution
