One mole of an ideal gas at pressure P0 and temperature T0 is expanded isothermally to twice its volume and then compressed at constant pressure to (V0/2) and the gas is brought bac to original state by a process in which PαV (pressure is directly proportional to volume). The correct temperature of the process is
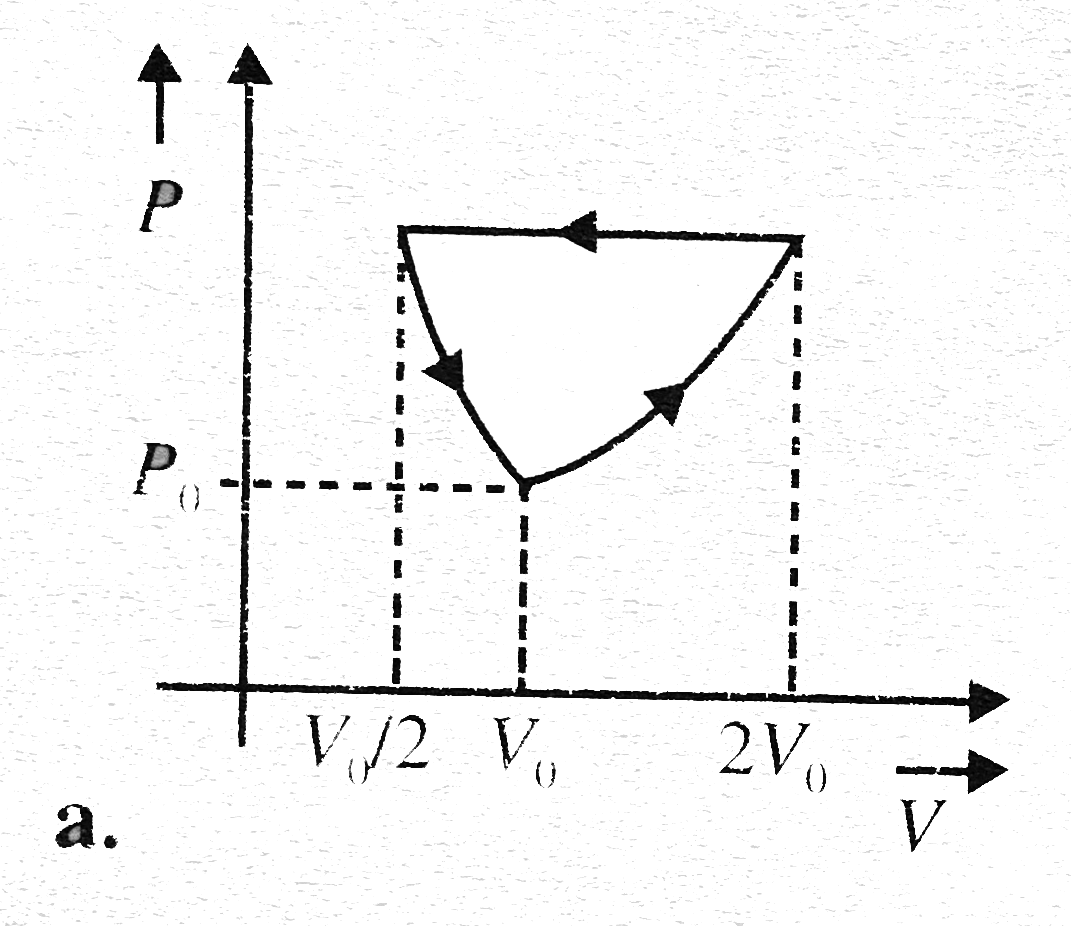



The correct Answer is:C
To solve the problem step by step, we will analyze the three processes that the gas undergoes and determine the final temperature of the gas after it has returned to its original state.
Step 1: Understand the Initial Conditions
We have 1 mole of an ideal gas at:
- Initial Pressure: P0
- Initial Temperature: T0
- Initial Volume: V0
Step 2: Process 1 - Isothermal Expansion
The gas is expanded isothermally to twice its volume:
- Final Volume after expansion: V=2V0
Since the process is isothermal, the temperature remains constant at T0. According to Boyle's Law for isothermal processes:
P0V0=P1(2V0)
From this, we can find the new pressure P1:
P1=P0V02V0=P02
Step 3: Process 2 - Isobaric Compression
Next, the gas is compressed at constant pressure to V0/2:
- Final Volume after compression: V=V02
- Pressure remains constant: P1=P0/2
Using the ideal gas law PV=nRT:
P02⋅V02=nRT1
Where T1 is the temperature after this process. Substituting n=1 (1 mole):
P0V04=RT1
Thus,
T1=P0V04R
Step 4: Process 3 - P is Proportional to V
In the final process, the gas is brought back to the original state with P∝V. This means:
P=kV
Using the ideal gas law again, we can express this as:
PV=nRT
Substituting P=kV:
kV2=nRT
This implies:
T=kV2nR
Step 5: Returning to Original State
To find the temperature at the end of the third process, we need to know the values of k and V. Since the process returns to the original state, we know:
- Final Volume: V=V0
- Final Pressure: P=P0
Substituting back into the equation:
T=kV20nR
Step 6: Relate k to Original Conditions
From the previous processes, we can relate k to the original conditions. Since we know the gas returns to its original state, we can set T equal to T0:
T0=kV20R
Final Calculation
To find the correct temperature of the process, we need to relate it back to the initial temperature:
- After the first process, the temperature remains T0.
- After the second process, the temperature is T1=P0V04R.
- The final temperature after the third process must also relate back to the original state.
Thus, the correct temperature of the process is:
T=T0
Conclusion
The correct temperature of the process is T0.
We have 1 mole of an ideal gas at:
- Initial Pressure: P0
- Initial Temperature: T0
- Initial Volume: V0
The gas is expanded isothermally to twice its volume:
- Final Volume after expansion: V=2V0
P0V0=P1(2V0)
From this, we can find the new pressure P1:
P1=P0V02V0=P02
Next, the gas is compressed at constant pressure to V0/2:
- Final Volume after compression: V=V02
- Pressure remains constant: P1=P0/2
P02⋅V02=nRT1
Where T1 is the temperature after this process. Substituting n=1 (1 mole):
P0V04=RT1
Thus,
T1=P0V04R
In the final process, the gas is brought back to the original state with P∝V. This means:
P=kV
Using the ideal gas law again, we can express this as:
PV=nRT
Substituting P=kV:
kV2=nRT
This implies:
T=kV2nR
To find the temperature at the end of the third process, we need to know the values of k and V. Since the process returns to the original state, we know:
- Final Volume: V=V0
- Final Pressure: P=P0
T=kV20nR
From the previous processes, we can relate k to the original conditions. Since we know the gas returns to its original state, we can set T equal to T0:
T0=kV20R
To find the correct temperature of the process, we need to relate it back to the initial temperature:
- After the first process, the temperature remains T0.
- After the second process, the temperature is T1=P0V04R.
- The final temperature after the third process must also relate back to the original state.
T=T0
The correct temperature of the process is T0.
Topper's Solved these Questions
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Multiple Corrects
Explore 29 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Assertion-Reasoning
Explore 6 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Subjective
Explore 22 Videos
- KINETIC THEORY OF GASESBook:CENGAGE PHYSICS ENGLISHChapter:KINETIC THEORY OF GASESExercise:Compression
Explore 2 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBook:CENGAGE PHYSICS ENGLISHChapter:LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONExercise:Single correct anwer type
Explore 14 Videos
Similar Questions
Three moles of an ideal gas at a pressure PA and temperature TA is isothermally expanded to twice its initial volume. It is then compressed at constant pressure to its original , volume. Finally the gas is compressed at constant volume to its original pressure PA
(a) Sketch the P-V and P-T diagrams for the complete process.
(b) Calculate the net work done by the gas, and net heat supplied to the gas during the complete process.
View SolutionAn ideal gas has pressure P, volume V and temperature T. Select the correct option.
View Solution
Knowledge Check
A mass of ideal gas at pressure P is expanded isothermally to four times the originl volume and then slowly compressed adiabatically to its original volume. Assuming γ to be 1.5 , the new pressure of the gas is
A0.58333333333333BPC0.66666666666667DP/2
A sample of an ideal gas has pressure p0, volume V0 and tempreture T0. It is isothermally expanded to twice its oringinal volume.it is then compressed at constant pressure to have the original volume V0. Finally, the gas is heated at constant volume to get the original tempreture.(a) show the process in a V-T diagram (b) calculate the heat absorbed in the process.
View SolutionThree moles of an ideal gas (Cp=72R) at pressure, PA and temperature TA is isothermally expanded to twice its initial volume. It is then compressed at constant pressure to its original volume. Finally gas is compressed at constant volume to its original pressure PA.
(a) Sketch P-V and P-T diagrams for the complete process.
(b) Calculate the net work done by the gas, and net heat supplied to the gas during the complete process.
View SolutionAn ideal gas of volume V and pressure P expands isothermally to volume 16 V and then compressed adiabatically to volume V . The final pressure of gas is [γ=1.5]
View SolutionThree moles of an ideal gas (Cp=72R) at pressure p0 and temperature T0 is isothermally expanded to twice its initial volume. It is then compressed at a constant pressure to its original volume.
(a) Sketch p-V and p-T diagram for complete process.
(b) Calculate net work done by the gas.
(c) Calculate net heat supplied to the gas during complete process.
(Write your answer in terms of gas constant =R)
View SolutionA monoatomic gas at pressure P1 and volume V1 is compressed adiabatically to 18th of its original volume. What is the final pressure of gas.
View SolutionA quantity of gas occupies an initial volume V0 at pressure p0 and temperature T0. It expands to a volume V (a) constant temperature and (b) constant pressure. In which case does the gas do more work ?
View SolutionA monatomic gas at a pressure P, having a volume V expands isothermally to a volume 2 V and then adiabatically to a volume 16 V. The final pressure of the gas is ( take γ=53)
View Solution