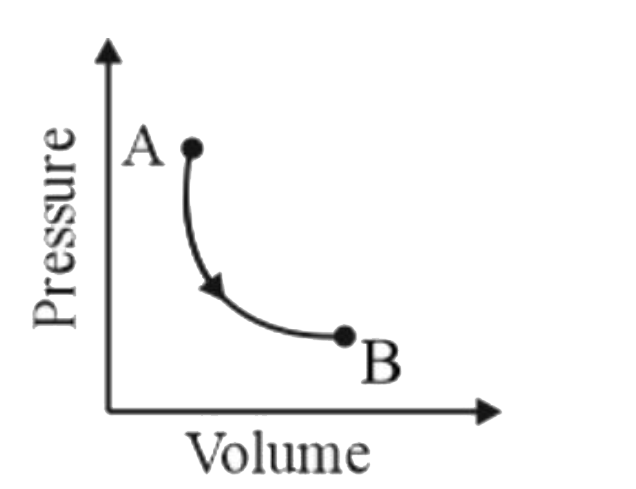
On an isothermal process, there are two POINTs $A$ and $B$ at which pressures and volumes are $(2 P_{0}, V_{0})$ and $(P_{0}, 2 V_{0})$ respectively. If $A$ and $B$ are connected by a straight line, find the pressure at a POINT on this straight line at which temperature is maximum

A

$\frac{4 P_{0}}{3}$

B

$\frac{5}{4} P_{0}$

C

$\frac{3}{2} P_{0}$

D

$\frac{7}{5} P_{0}$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:C

To solve the problem step by step, we will follow the process outlined in the video transcript.
Step 1: Understand the Points A and B
We have two points A and B on an isothermal process:
- Point A: Pressure $P_{A} = 2 P_{0}$ , Volume $V_{A} = V_{0}$
- Point B: Pressure $P_{B} = P_{0}$ , Volume $V_{B} = 2 V_{0}$
Step 2: Equation of the Straight Line AB
The equation of the straight line connecting points A and B can be derived using the two-point form of the line equation. The general form is:
$\frac{y - y_{A}}{x - x_{A}} = \frac{y_{B} - y_{A}}{x_{B} - x_{A}}$
Here, we replace $y$ with pressure $P$ and $x$ with volume $V$ :
$\frac{P - 2 P_{0}}{V - V_{0}} = \frac{P_{0} - 2 P_{0}}{2 V_{0} - V_{0}}$
This simplifies to:
$\frac{P - 2 P_{0}}{V - V_{0}} = \frac{- P_{0}}{V_{0}}$
Step 3: Rearranging the Equation
Cross-multiplying gives:
$P - 2 P_{0} = - \frac{P_{0}}{V_{0}} (V - V_{0})$
Rearranging this leads to:
$P = 2 P_{0} - \frac{P_{0}}{V_{0}} (V - V_{0})$
Step 4: Expressing PV
Multiplying both sides by $V$ :
$P V = V (2 P_{0}) - \frac{P_{0}}{V_{0}} (V^{2} - V V_{0})$
This can be expressed as:
$P V = 2 P_{0} V - \frac{P_{0}}{V_{0}} V^{2} + \frac{P_{0}}{V_{0}} V V_{0}$
Step 5: Relating to Temperature
Using the ideal gas law $P V = n R T$ , we can equate:
$n R T = 2 P_{0} V - \frac{P_{0}}{V_{0}} V^{2} + P_{0} V$
Rearranging gives:
$n R T = 2 P_{0} V - \frac{P_{0}}{V_{0}} V^{2} + P_{0} V$
Step 6: Finding Maximum Temperature
To find the maximum temperature, we differentiate $T$ with respect to $V$ and set the derivative to zero:
$\frac{d T}{d V} = 0$
This leads to:
$2 P_{0} - \frac{P_{0}}{V_{0}} (2 V - V_{0}) = 0$
Step 7: Solving for V
Solving the equation:
$2 P_{0} = \frac{P_{0}}{V_{0}} (2 V - V_{0})$
Cancelling $P_{0}$ and rearranging gives:
$2 = \frac{2 V}{V_{0}} - 1$
Thus,
$3 = \frac{2 V}{V_{0}}$
This implies:
$V = \frac{3}{2} V_{0}$
Step 8: Finding Pressure at Maximum Temperature
Substituting $V = \frac{3}{2} V_{0}$ back into the equation for pressure:
$P = 2 P_{0} - \frac{P_{0}}{V_{0}} (\frac{3}{2} V_{0} - V_{0})$
This simplifies to:
$P = 2 P_{0} - \frac{P_{0}}{V_{0}} (\frac{1}{2} V_{0}) = 2 P_{0} - \frac{1}{2} P_{0} = \frac{3}{2} P_{0}$
Final Answer
The pressure at the point on the straight line where the temperature is maximum is:
$\frac{3}{2} P_{0}$

|

Updated on:18/9/2024

Class 11 PHYSICS KINETIC THEORY OF GASES

Topper's Solved these Questions

Similar Questions

CENGAGE PHYSICS ENGLISH-KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICS-Single Correct

Home
Ask Doubt
Profile