Three particle and one particle decaying takes place in series from an isotope . Finally the isotope obtained will be.
The correct Answer is:C
To solve the problem of determining the isotope obtained after three alpha decays and one beta decay from the isotope 23888Ra, we can follow these steps:
Step 1: Understand the decay process
- An alpha particle (α) consists of 2 protons and 2 neutrons, which means it decreases the atomic mass by 4 and the atomic number by 2.
- A beta particle (β) is an electron emitted from a nucleus, which effectively increases the atomic number by 1 (since a neutron is converted into a proton).
Step 2: Calculate the change in atomic mass (A)
- Starting with A=238 (the mass number of radium).
- Since three alpha particles are emitted, the total decrease in mass due to alpha decay is:
Decrease in mass=3×4=12
- Therefore, the new mass number A′ after the alpha decays will be:
A′=238−12=226
Step 3: Calculate the change in atomic number (Z)
- Starting with Z=88 (the atomic number of radium).
- Each alpha decay decreases the atomic number by 2, so for three alpha particles:
Decrease in atomic number=3×2=6
- After three alpha decays, the atomic number becomes:
Z′=88−6=82
- Now, we have one beta decay, which increases the atomic number by 1:
Z′′=82+1=83
Step 4: Write the final isotope
- After all the decays, the final isotope will have:
- Mass number A=226
- Atomic number Z=83
- The resulting isotope can be represented as:
22683Bi
- Therefore, the final isotope obtained after the decay process is Bismuth (Bi).
Final Answer
The isotope obtained after the decay process is 22683Bi.
---
- An alpha particle (α) consists of 2 protons and 2 neutrons, which means it decreases the atomic mass by 4 and the atomic number by 2.
- A beta particle (β) is an electron emitted from a nucleus, which effectively increases the atomic number by 1 (since a neutron is converted into a proton).
- Starting with A=238 (the mass number of radium).
- Since three alpha particles are emitted, the total decrease in mass due to alpha decay is:
Decrease in mass=3×4=12
- Therefore, the new mass number A′ after the alpha decays will be:
A′=238−12=226
- Starting with Z=88 (the atomic number of radium).
- Each alpha decay decreases the atomic number by 2, so for three alpha particles:
Decrease in atomic number=3×2=6
- After three alpha decays, the atomic number becomes:
Z′=88−6=82
- Now, we have one beta decay, which increases the atomic number by 1:
Z′′=82+1=83
- After all the decays, the final isotope will have:
- Mass number A=226
- Atomic number Z=83
- The resulting isotope can be represented as:
22683Bi
- Therefore, the final isotope obtained after the decay process is Bismuth (Bi).
The isotope obtained after the decay process is 22683Bi.
Topper's Solved these Questions
- NUCLEAR PHYSICSBook:CENGAGE PHYSICS ENGLISHChapter:NUCLEAR PHYSICSExercise:ddp.5.4
Explore 15 Videos
- NUCLEAR PHYSICSBook:CENGAGE PHYSICS ENGLISHChapter:NUCLEAR PHYSICSExercise:ddp.5.5
Explore 14 Videos
- NUCLEAR PHYSICSBook:CENGAGE PHYSICS ENGLISHChapter:NUCLEAR PHYSICSExercise:ddp.5.2
Explore 15 Videos
- MISCELLANEOUS VOLUME 5Book:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS VOLUME 5Exercise:Integer
Explore 12 Videos
- PHOTOELECTRIC EFFECTBook:CENGAGE PHYSICS ENGLISHChapter:PHOTOELECTRIC EFFECTExercise:Integer Type
Explore 4 Videos
Similar Questions
A nucleus .mnX emits one α−particle and two β−particles. The resulting nucleus is
View SolutionA : During radioactive disintegration an α-particle and a β-particle do not emit simultaneously from any nucleus.
R : An α-particle emits from a nucleus when the N/Z ratio is less than the stability range (where N=number of neutrons and Z=number of protons in a nucleus. )
View Solution
Knowledge Check
A radioactive decay can form an isotope of the original nucleus with the emission of particles
Aone α and four βBone α and two βCone α and one βDfour α and one β
IN radioactive series, an inner transition element .92U238 loses one α-particles and one β-particle to produce a very unstable daughter nuclei .91Pa234 with half life 1.14 min. Find out the displacement in group due to these emissions.
View SolutionThe number of αandβ particles emitted in the nucleur disintegration series _90228Th→21283Bi are:
View SolutionA uranium nucleus .92U238 emits and α-particle and a β-particle in succession. The atomic number and mass number of the final nucleus will be
View Solution.92U238 emits 8α− particles and 6β− particles. The n/p ratio in the product nucleus is
View SolutionIf a nucleus .AZx emits one α-particle and one β (negative β) particle in succession, then the daughter nucleus will have which of the following configurations?
View SolutionA uranium nucleus U−238 of atomic number92 emits two α- particles and two β-particles and trasnforms into a thorium nucleus. What is the mass number and atomic number of the thorium nucleus so produced?
View Solution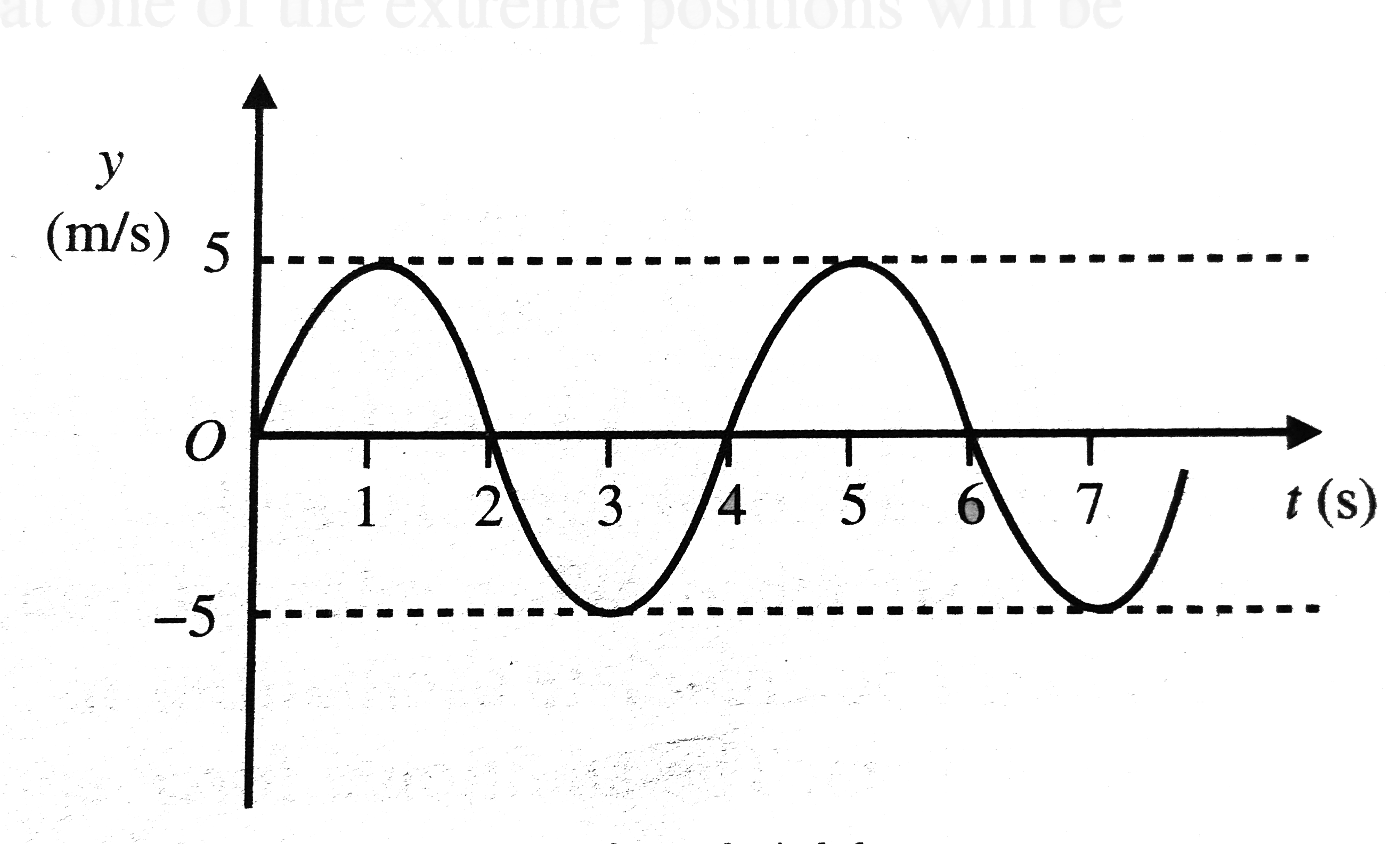
The variation of velocity of a particle executing SHM with time is shown is fig. The velocity of the particle when a phase change of π6 takes place from the instant it is at one of the extreme positions will be
View Solution