Equilibrium mixture of ice and water is held at constant pressure. On heating some ice melts. For the system
(a) Entropy increases
(b) Free energy increases
(c) Free energy decreases
(d) Entropy decreases
To solve the problem regarding the equilibrium mixture of ice and water held at constant pressure, we need to analyze the changes that occur when some ice melts upon heating. Here’s a step-by-step solution:
Step 1: Understand the Initial Condition
We start with an equilibrium mixture of ice (solid) and water (liquid) at a constant pressure. This means that both phases are present and in balance at a specific temperature (0°C for ice and water).
Hint: Remember that at equilibrium, the rates of melting and freezing are equal.
Step 2: Heating the System
When we heat the system, some of the ice begins to melt. This process involves the conversion of solid ice into liquid water.
Hint: Heating adds energy to the system, which can change the state of matter.
Step 3: Analyze the Change in Entropy
As ice melts into water, the degree of randomness or disorder in the system increases. In solid ice, the molecules are tightly packed and have limited movement. In liquid water, the molecules are more spread out and can move freely, leading to a higher entropy.
Hint: Entropy is a measure of disorder; melting increases disorder.
Step 4: Conclusion on Entropy
Since the melting of ice results in an increase in disorder, we conclude that the entropy of the system increases.
Hint: Look for changes in molecular arrangement to determine changes in entropy.
Step 5: Analyze the Change in Gibbs Free Energy
The change in Gibbs free energy (ΔG) is related to the spontaneity of the process. For a spontaneous process, ΔG is negative. Since the melting of ice into water is a spontaneous process at the given conditions, we can conclude that ΔG decreases.
Hint: A negative ΔG indicates that the process can occur without external input.
Step 6: Final Summary
In summary, when ice melts into water at constant pressure:
- The entropy of the system increases.
- The Gibbs free energy of the system decreases.
Final Answer
- Entropy increases (correct).
- Gibbs free energy decreases (correct).
We start with an equilibrium mixture of ice (solid) and water (liquid) at a constant pressure. This means that both phases are present and in balance at a specific temperature (0°C for ice and water).
When we heat the system, some of the ice begins to melt. This process involves the conversion of solid ice into liquid water.
As ice melts into water, the degree of randomness or disorder in the system increases. In solid ice, the molecules are tightly packed and have limited movement. In liquid water, the molecules are more spread out and can move freely, leading to a higher entropy.
Since the melting of ice results in an increase in disorder, we conclude that the entropy of the system increases.
The change in Gibbs free energy (ΔG) is related to the spontaneity of the process. For a spontaneous process, ΔG is negative. Since the melting of ice into water is a spontaneous process at the given conditions, we can conclude that ΔG decreases.
In summary, when ice melts into water at constant pressure:
- The entropy of the system increases.
- The Gibbs free energy of the system decreases.
- Entropy increases (correct).
- Gibbs free energy decreases (correct).
Topper's Solved these Questions
- THERMODYNAMICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - Exercise
1 Videos
- THERMODYNAMICSBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - Ex 6.1
26 Videos
- STOICHIOMETRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - STOICHIOMETRYEXERCISE - Archives Subjective
33 Videos
Similar Questions
Knowledge Check
Melting of ice into water is a
Achemical changeBphysical changeCirreversible changeDperiodic change
Molar heat capacity of water in equilibrium with the ice at constant pressure is :
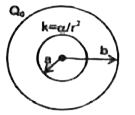
View SolutionAn ice ball of radius a and mass m is placed at 0∘C inside a thick hollow sphere of inner and outer radii a and b whose thermal conductivity varies as k=αr2, where α is a positive constant and r is the distance from centre. The temperature of surrounding is θ0 and latent heat of fusion for ice is Lf. Then , time required to completely melt the ice ball is (Neglect the contraction in volume when ice melts into water and any change in thermal resistance due to melting of ice of the system.)
View SolutionState the effect of increase of pressure on the melting point of ice.
View SolutionWhen1 mole of ice melt at 0∘ C and at constant pressure of 1 atm . 1440 calories of heat are absorbed by the system. The molar volumes of ice and water are 0.0196 and 0.0180 litres respectively. Calculate ΔH and ΔE for the reaction.
View SolutionA metal piece weighing 15g is heated to 100∘C and then immersed in a mixture of ice and water at the thermal equilibrium. The volume of the mixture is found to be reduced by 0.15cm3 with the temperature of mixture remaining constant. Find the specific heat of the metal. Given specific gravity of ice =0.92, specific gravity of water at 0∘C=1.0, latent heat of fusion of ice =80cal−g−1.
View SolutionWhen ice melts at 1∘C :
View SolutionEqual masses of ice (at0∘C) and water are in contact. Find the temperature of water needed to just melt the complete ice.
View Solution
