A particle of mass m can move along x -axis of co-ordinate frame in a force field of stationary sources. Potential energy ' U of system varies wita position x of the particle according to the equation U=k|x|, where k is a positive constant. If the particle is projected from the origin with a kinetic energy E, and its period of bounded motion is Nk√mE2. Find the value of N.
The correct Answer is:8
Topper's Solved these Questions
Similar Questions
A particle of mass m is present in a region where the potential energy of the particle depends on the x-coordinate according to the expression U=ax2−bx, where a and b are positive constant. The particle will perform.
View SolutionA particle of mass 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is

View Solution
Knowledge Check
A particle of mass m is present in a region where the potential energy of the particle depends on the x-coordinate according to the expression U=ax2−bx, where a and b are positive constant. The particle will perform.
Aoscillatory motion but not simple harmonic motion about its mean position for small displacementsBsimple harmonic motion with time period 2π√8a2mb4 about its mean position for small displacementsCneither simple harmonic motion nor oscillatory about its mean position for small displacementsDnone of the aboveA particle of mass m is executing oscillations about the origin on the x-axis, it's potential energy is U(x)=k|x|2, where k is a positive constant, if the amplitude of oscillation is a, then it's time period T is
AProportional o1√aBIndependent of aCProportional to √aDProportional to a3/2A particle of mass 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is
 A2π5sB2√2π5sC√2π5sD4π5s
A2π5sB2√2π5sC√2π5sD4π5s
A particle of mass 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is

View SolutionA particle of mass = 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is nπ5 second. Find value of n.
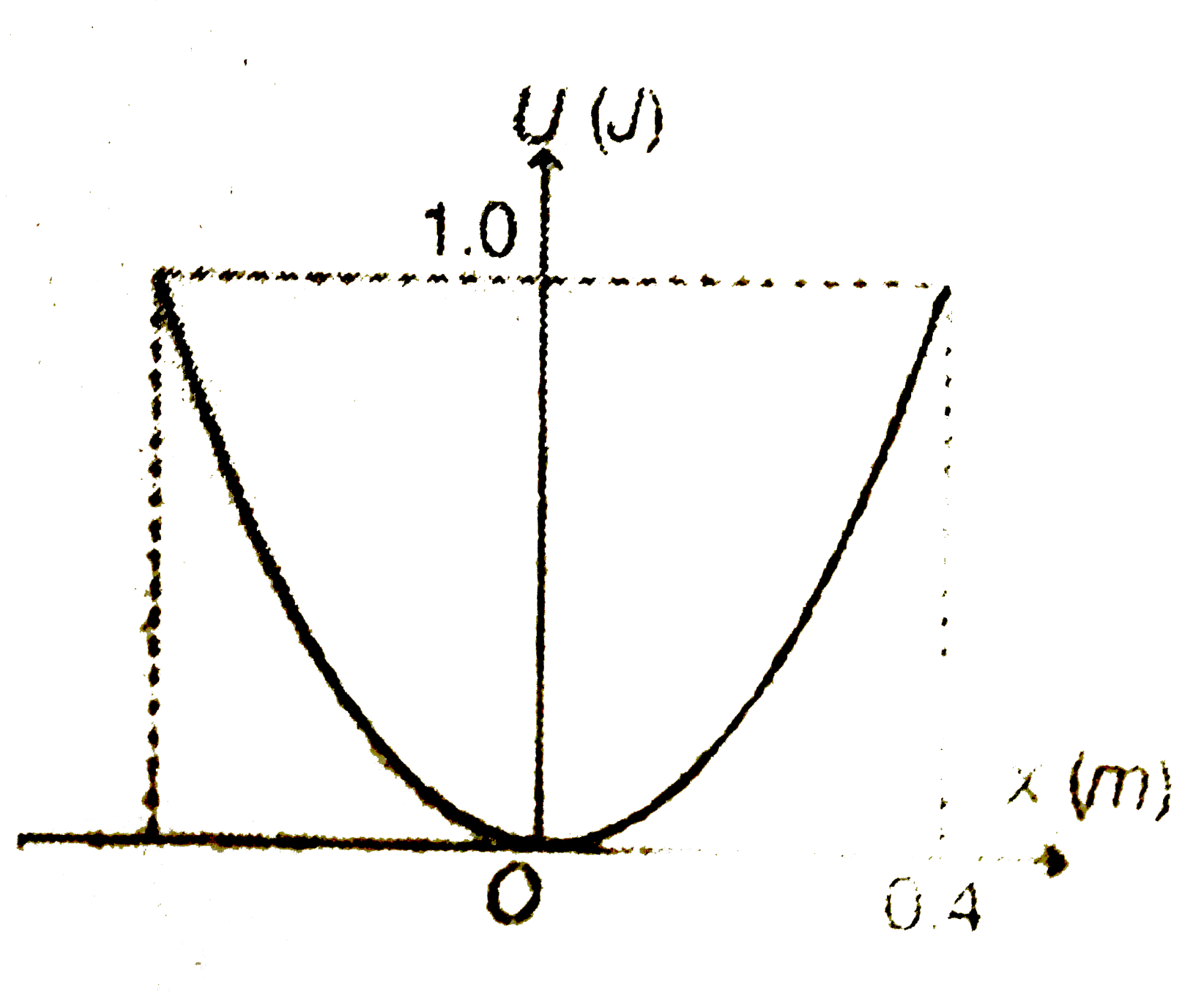
View SolutionA particle of mass = 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is nπ5 second. Find value of n.

View SolutionA particle of mass (m) is executing oscillations about the origin on the (x) axis. Its potential energy is V(x)=k|x|3 where (k) is a positive constant. If the amplitude of oscillation is a, then its time period (T) is.
View SolutionA particle of mass (m) is executing oscillations about the origin on the (x) axis. Its potential energy is V(x)=k|x|3 where (k) is a positive constant. If the amplitude of oscillation is a, then its time period (T) is.
View Solution
