A car travels half of the distance with constant velocity 40 km/h and another half with a constant velocity of 60 km/h along a straight line. The average velocity of the car is
24 km/h
48 km/h
60 km/h
40 km/h
The correct Answer is:B
To find the average velocity of the car that travels half of the distance at a constant velocity of 40 km/h and the other half at 60 km/h, we can follow these steps:
Step 1: Define the total distance
Let the total distance traveled by the car be D. Therefore, half of the distance is D2.
Step 2: Calculate the time taken for the first half of the distance
The car travels the first half of the distance D2 at a velocity of 40 km/h.
Using the formula for time:
t1=distancevelocity=D240=D80 hours
Step 3: Calculate the time taken for the second half of the distance
The car travels the second half of the distance D2 at a velocity of 60 km/h.
Using the same formula for time:
t2=distancevelocity=D260=D120 hours
Step 4: Calculate the total time taken
The total time taken T is the sum of the times for both halves:
T=t1+t2=D80+D120
To add these fractions, we need a common denominator. The least common multiple of 80 and 120 is 240.
T=3D240+2D240=5D240=D48 hours
Step 5: Calculate the average velocity
The average velocity Vavg is defined as the total displacement divided by the total time taken:
Vavg=Total DistanceTotal Time=DT=DD48=48 km/h
Final Answer
The average velocity of the car is 48 km/h.
---
Let the total distance traveled by the car be D. Therefore, half of the distance is D2.
The car travels the first half of the distance D2 at a velocity of 40 km/h.
Using the formula for time:
t1=distancevelocity=D240=D80 hours
The car travels the second half of the distance D2 at a velocity of 60 km/h.
Using the same formula for time:
t2=distancevelocity=D260=D120 hours
The total time taken T is the sum of the times for both halves:
T=t1+t2=D80+D120
To add these fractions, we need a common denominator. The least common multiple of 80 and 120 is 240.
T=3D240+2D240=5D240=D48 hours
The average velocity Vavg is defined as the total displacement divided by the total time taken:
Vavg=Total DistanceTotal Time=DT=DD48=48 km/h
The average velocity of the car is 48 km/h.
Topper's Solved these Questions
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - B)
19 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - C)
7 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Try Yourself
40 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - D)
15 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION D
16 Videos
Similar Questions
A car travels half of the distance with constant velocity 100 km/h and another half with a constant velocity of 60 km/h along a straight line. The average velocity of the car is
View SolutionA car of mass 800 kg is moving with a constant velocity 40 km/h. Find the net force on it.
View SolutionA car of mass 800 kg is moving with a constant velocity 40 km/h . Find the net force on it.
View SolutionA car completes the first half of its journey with a velocity V1 and the remaining half with a velocity V2. Then the average velocity of the car for the whole journey is
View SolutionA car A moves along north with velocity 30 km/h and another car B moves along east with velocity 40 km/h. The relative velocity of A with respect to B is
View SolutionA person travels along a straight road for half the distance with velocity V1 and the remaining half distance with velocity V2 the average velocity is given by
View SolutionA car travels the first half of a distance between two places a speed of 30 km/hr and the second half of the distance a 50 km/hr. The average speed of the car for the whole journey is
View SolutionThe velocity-time graph of a car moving along a straight road is shown in figure. The average velocity of the car is first 25 seconds is
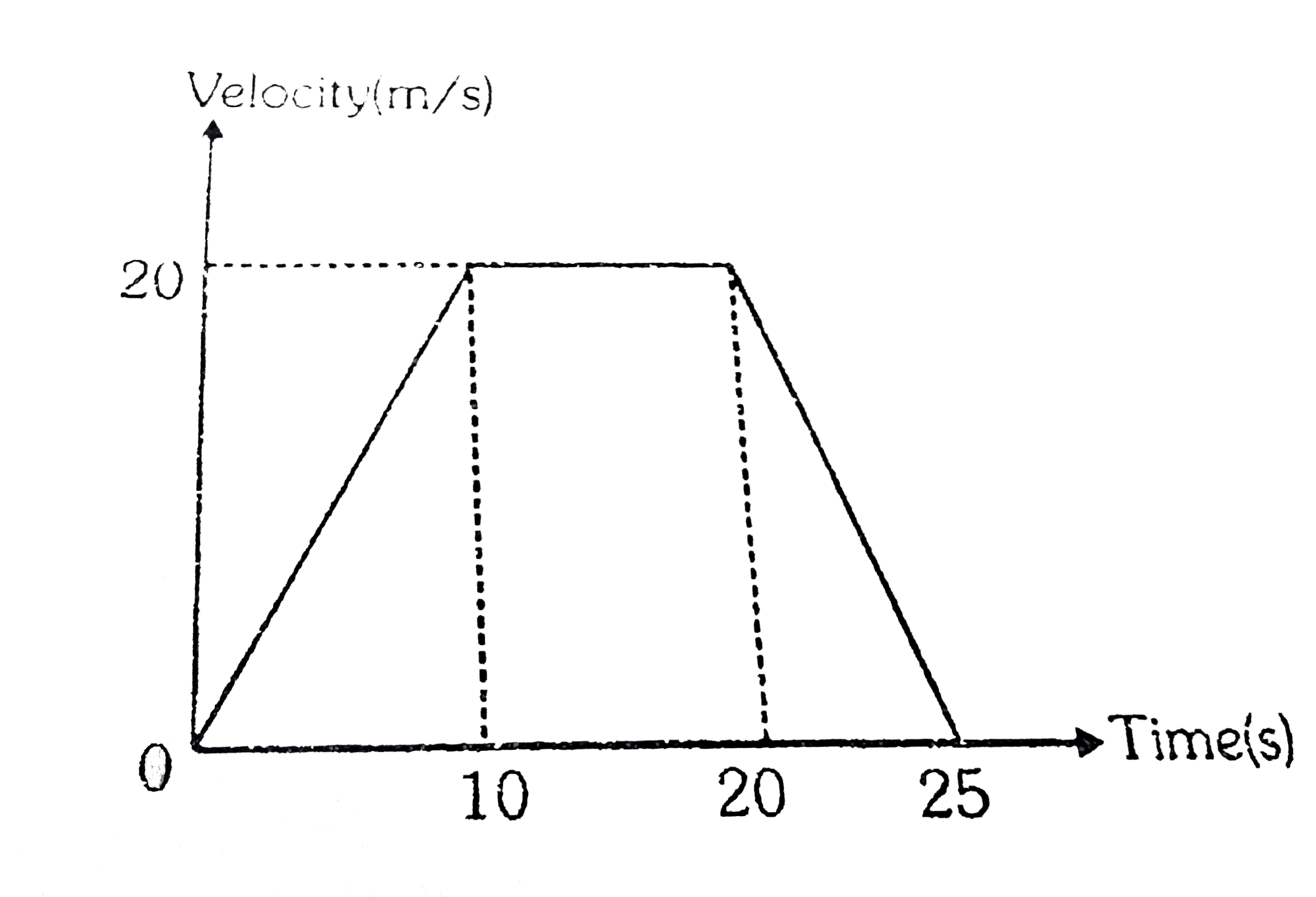
View SolutionThe velocity-time graph of a car moving along a straight road is shown in figure. The average velocity of the car in first 25 seconds is
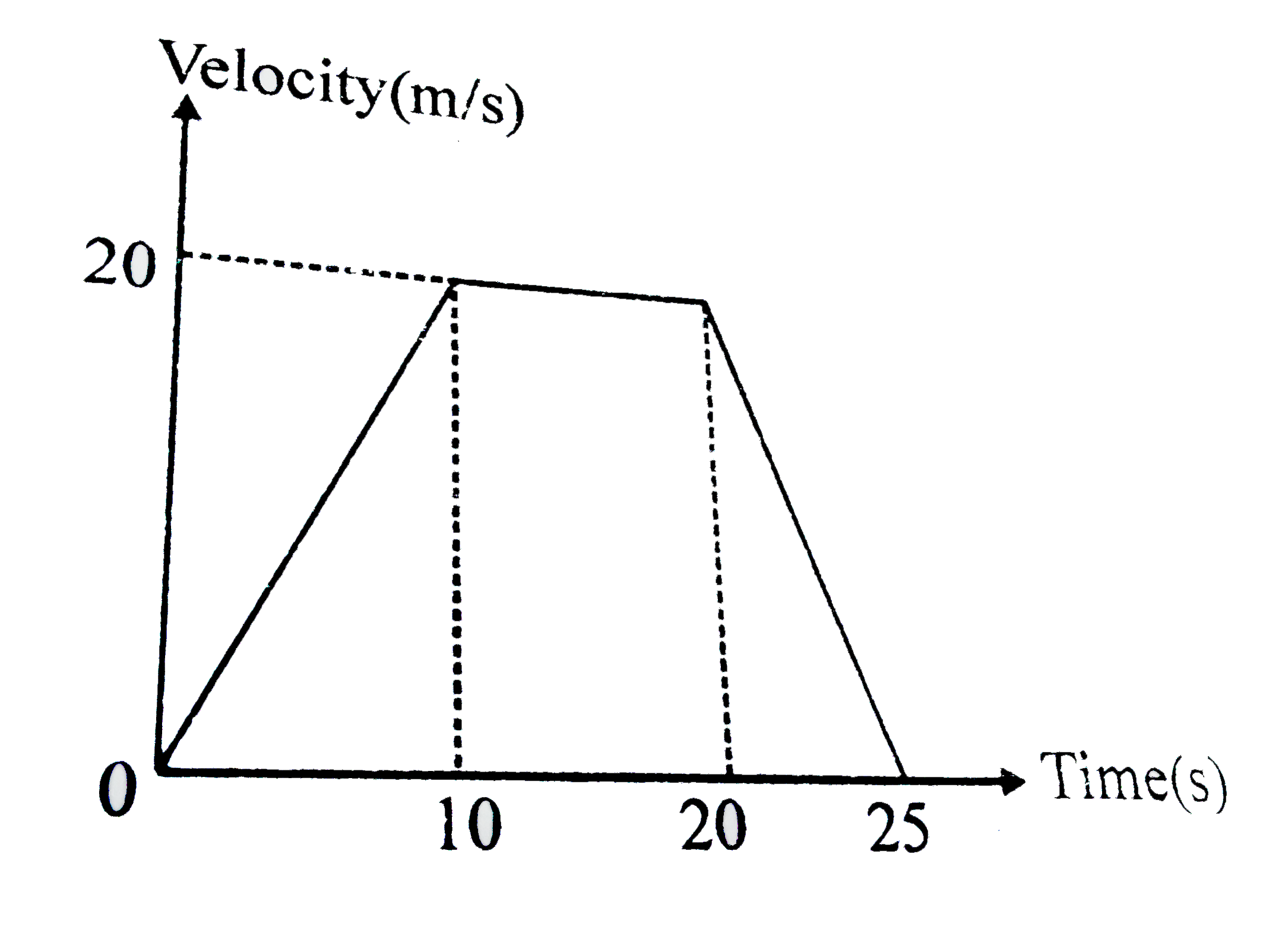
View SolutionA body travelling along a straight line traversed one third of the total distance with a velocity 4m/s. The remaining part of the distance was covered with a velocity 1 m/s for half the time and with velocity 3 m/s for the other half of time. The mean velocity averaged over the whole time of motion is :
View Solution
