A simple pendilum with bob of mass m and length l is held in position at an angle with t he verticle. Find its speed when it passes the lowest position.
To find the speed of the bob of a simple pendulum when it passes the lowest position, we can use the principle of conservation of energy. Here’s a step-by-step solution:
Step 1: Understand the Initial and Final Positions
- The pendulum is initially held at an angle θ with the vertical.
- At this position, the bob has gravitational potential energy and no kinetic energy (since it is at rest).
- When the bob reaches the lowest position, all the potential energy will have been converted into kinetic energy.
Step 2: Calculate the Change in Height
- The height h through which the bob falls can be calculated using the length of the pendulum l and the angle θ.
- The vertical height fallen by the bob is given by:
h=l−lcosθ=l(1−cosθ)
Step 3: Write the Expression for Potential Energy
- The gravitational potential energy (PE) at the initial position is given by:
PE=mgh=mg⋅h=mg⋅l(1−cosθ)
Step 4: Write the Expression for Kinetic Energy
- The kinetic energy (KE) at the lowest position is given by:
KE=12mv2
Step 5: Apply Conservation of Energy
- According to the conservation of energy, the loss in potential energy equals the gain in kinetic energy:
mg⋅l(1−cosθ)=12mv2
Step 6: Simplify the Equation
- We can cancel m from both sides (assuming m≠0):
g⋅l(1−cosθ)=12v2
Step 7: Solve for v2
- Rearranging gives:
v2=2gl(1−cosθ)
Step 8: Solve for v
- Taking the square root of both sides gives us the speed v:
v=√2gl(1−cosθ)
Final Answer
- The speed of the bob when it passes the lowest position is:
v=√2gl(1−cosθ)
- The pendulum is initially held at an angle θ with the vertical.
- At this position, the bob has gravitational potential energy and no kinetic energy (since it is at rest).
- When the bob reaches the lowest position, all the potential energy will have been converted into kinetic energy.
- The height h through which the bob falls can be calculated using the length of the pendulum l and the angle θ.
- The vertical height fallen by the bob is given by:
h=l−lcosθ=l(1−cosθ)
- The gravitational potential energy (PE) at the initial position is given by:
PE=mgh=mg⋅h=mg⋅l(1−cosθ)
- The kinetic energy (KE) at the lowest position is given by:
KE=12mv2
- According to the conservation of energy, the loss in potential energy equals the gain in kinetic energy:
mg⋅l(1−cosθ)=12mv2
- We can cancel m from both sides (assuming m≠0):
g⋅l(1−cosθ)=12v2
- Rearranging gives:
v2=2gl(1−cosθ)
- Taking the square root of both sides gives us the speed v:
v=√2gl(1−cosθ)
- The speed of the bob when it passes the lowest position is:
v=√2gl(1−cosθ)
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-A (OBJECTIVE TYPE QUESTIONS (ONE OPTIONISCORRECT)
60 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-B (SUBJECTIVE TYPE QUESTIONS) (ONE OPTIONS IS CORRECT)
45 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - IILUSTRATION
2 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
A simple pendulum with bob of mass m and length x is held in position at an angle θ1 and then angle θ2 with the vertical. When released from these positions, speeds with which it passes the lowest postions are v1&v2 respectively. Then ,v1v2 is.
View SolutionA simple pendulum of length l is pulled aside to make an angle θ with the vertical. Find the magnitude of the torque of the weight w of the bob about the point of suspension. When is the torque zero?
View SolutionA bob hangs from a rigid support by an inextensible string of length l. It is released from rest when string makes an agngle 60∘ with vertical . The speed of the bob at the lowest position is
View SolutionA simple pendulum of mass m and length L is held in horizontal position. If it is released from this position, then find the angular momentum of the bob about the point of suspension when it is vertically below the point of suspension.
View SolutionWhat is the torque acting on the bob of mass m of a simple pendulum Of length l, when the string of the pendulum makes an angle θ with the vertical ? When is the torque (i) zero and (ii) maximum.
View SolutionA simple pendulum is constructed by attaching a bob of mass m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle θ with the vertical. Find the tension in the string at this instant.
View SolutionA simple pendulum is constructed by attaching a bob of mas m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle θ with the vertical. Find the tension in the string at this instant.
View Solution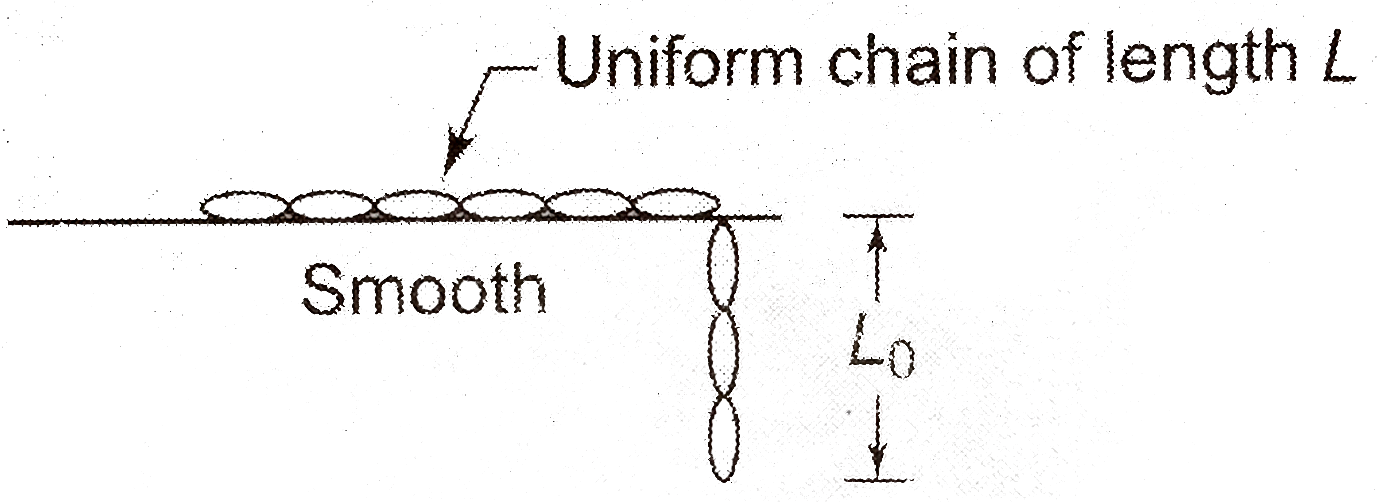
Consider a uniform chain of mass m and length L, the chain is released when hanging length is L0. Find the speed with which the chain leaves the table.
View SolutionA simple pendulum of length l and bob of mass m is displaced from its equilibrium position O to a position P so that hight of P above O is h . If is then released. What is the tension in the string when the bob passes through the through the equilibrium position O ? Neglect friction. v is the velocity of the bob at O
View SolutionA pendulum bob on a 2 m string is displaced 60∘ from the vertical and then released. What is the speed of the bob as it passes through the lowest point in its path
View Solution
