When a stick of length L mass M intially upright on a fricitionless floor starts falling the
Center of mass will fall vertically down
Center of mass will follow a circular path
Center of mass will follow any curved path
All of these
To solve the problem, we need to analyze the motion of a stick of length L and mass M that is initially upright on a frictionless floor and starts to fall.
1. Understanding the Forces Acting on the Stick:
- When the stick is upright, the only forces acting on it are the gravitational force acting downward (weight Mg) and the normal force acting upward from the ground.
- Since the floor is frictionless, there are no horizontal forces acting on the stick.
2. Identifying the Center of Mass:
- The center of mass of a uniform stick is located at its midpoint, which is at a distance of L2 from either end.
- As the stick starts to fall, we need to analyze the motion of this center of mass.
3. Analyzing the Motion:
- Since there are no horizontal forces acting on the stick, the center of mass will not move horizontally.
- The only motion that the center of mass will experience is due to the gravitational force acting on it, which will cause it to fall vertically downward.
4. Conclusion:
- Therefore, as the stick falls, the center of mass will follow a vertical path directly downward.
- The correct answer to the question is that the center of mass will fall vertically down.
Final Answer:
The center of mass will fall vertically down.
---
- When the stick is upright, the only forces acting on it are the gravitational force acting downward (weight Mg) and the normal force acting upward from the ground.
- Since the floor is frictionless, there are no horizontal forces acting on the stick.
- The center of mass of a uniform stick is located at its midpoint, which is at a distance of L2 from either end.
- As the stick starts to fall, we need to analyze the motion of this center of mass.
- Since there are no horizontal forces acting on the stick, the center of mass will not move horizontally.
- The only motion that the center of mass will experience is due to the gravitational force acting on it, which will cause it to fall vertically downward.
- Therefore, as the stick falls, the center of mass will follow a vertical path directly downward.
- The correct answer to the question is that the center of mass will fall vertically down.
The center of mass will fall vertically down.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - C) Objective Type Questions (More than one option are correct)
29 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - D) Linked Comprehension Type Questions
20 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - A) Objective Type Questions (One option is correct)
62 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))
5 Videos
- TEST 1BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE
21 Videos
Similar Questions
A stick of length L and mass M lies on a frictionless horizontal surface on which it is free to move in any way. A ball of mass m moving with speed v collides elastically with the stick as shown in the figure. If after the collision the ball comes to rest, then what would be the mass of the ball?

View SolutionA thin rod of length L and mass M is held vertically with one end on the floor and is allowed to fall. Find the velocity of the other end when it hits the floor, assuming that the end on the floor does not slip?
View SolutionA Stick of length L and mass M lies on a fnctionless horizontal surface on which it is free to move in any way. A ball of mass m moving with speed v collides elastically with the stick as shownin figure-5.115. If after the collision ball comes to rest, then what should be the mass of the ball ?

View SolutionAn insect of mass m is initially at one end of a stick of length L and mass M, which rests on a smooth floor. The coefficientg of friction between the insect and the stick is k. The minimum time in which the insect can reach the other end of the stick is t. Then t2 is equal to
View SolutionA plank of mass 2m and length L is al rest on a frictionless horizontal floor At one end of it, a child of mass m is standing as shown in figure If child walks and reaches the other and then the distance travelled by child wrt ground will be
View SolutionA uniform rod of mass M and length L is held vertically upright on a horizontal surface as shown in figure. Assuming zero potential energy at the base of the rod, determine the potential energy of the rod.
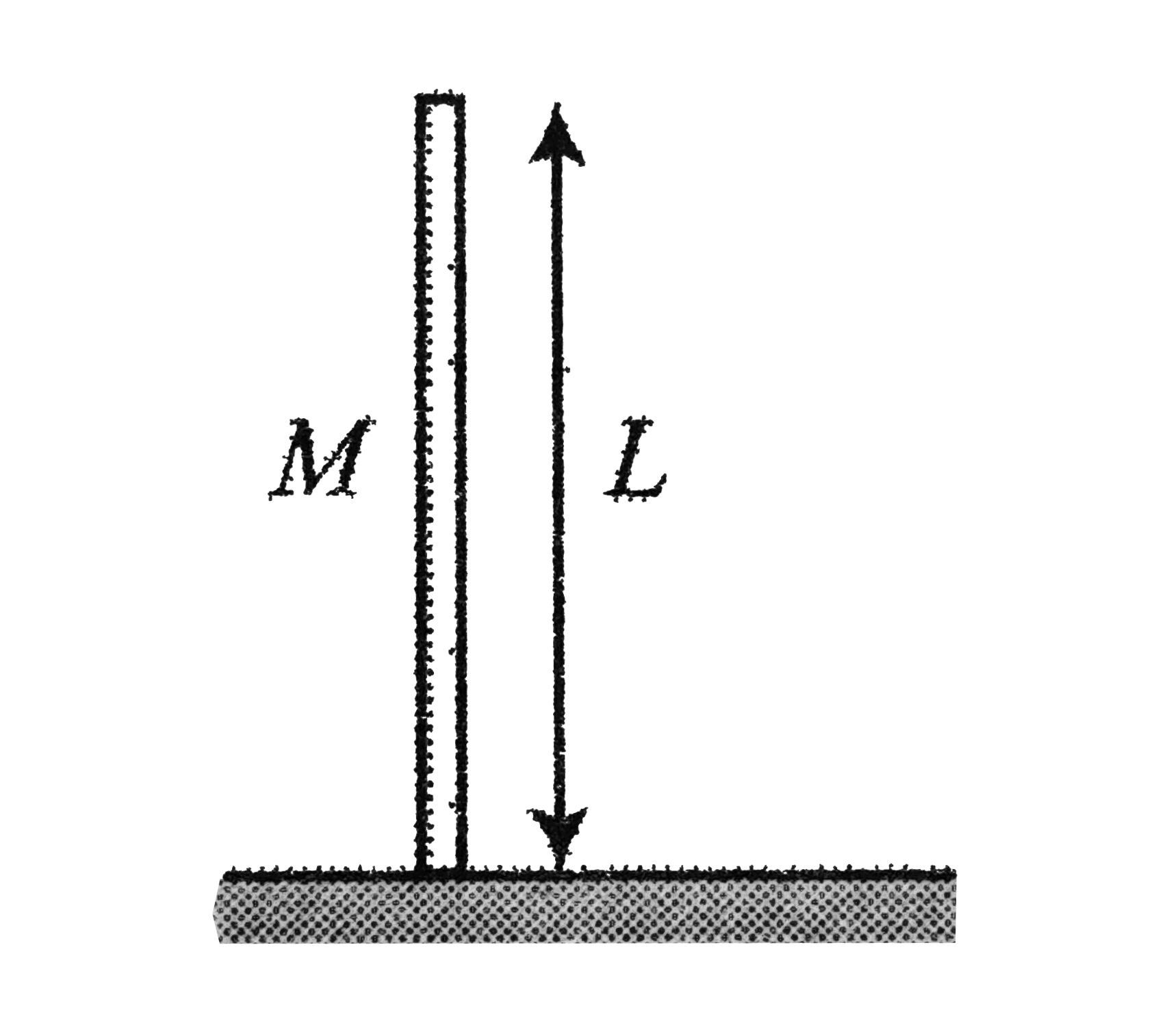
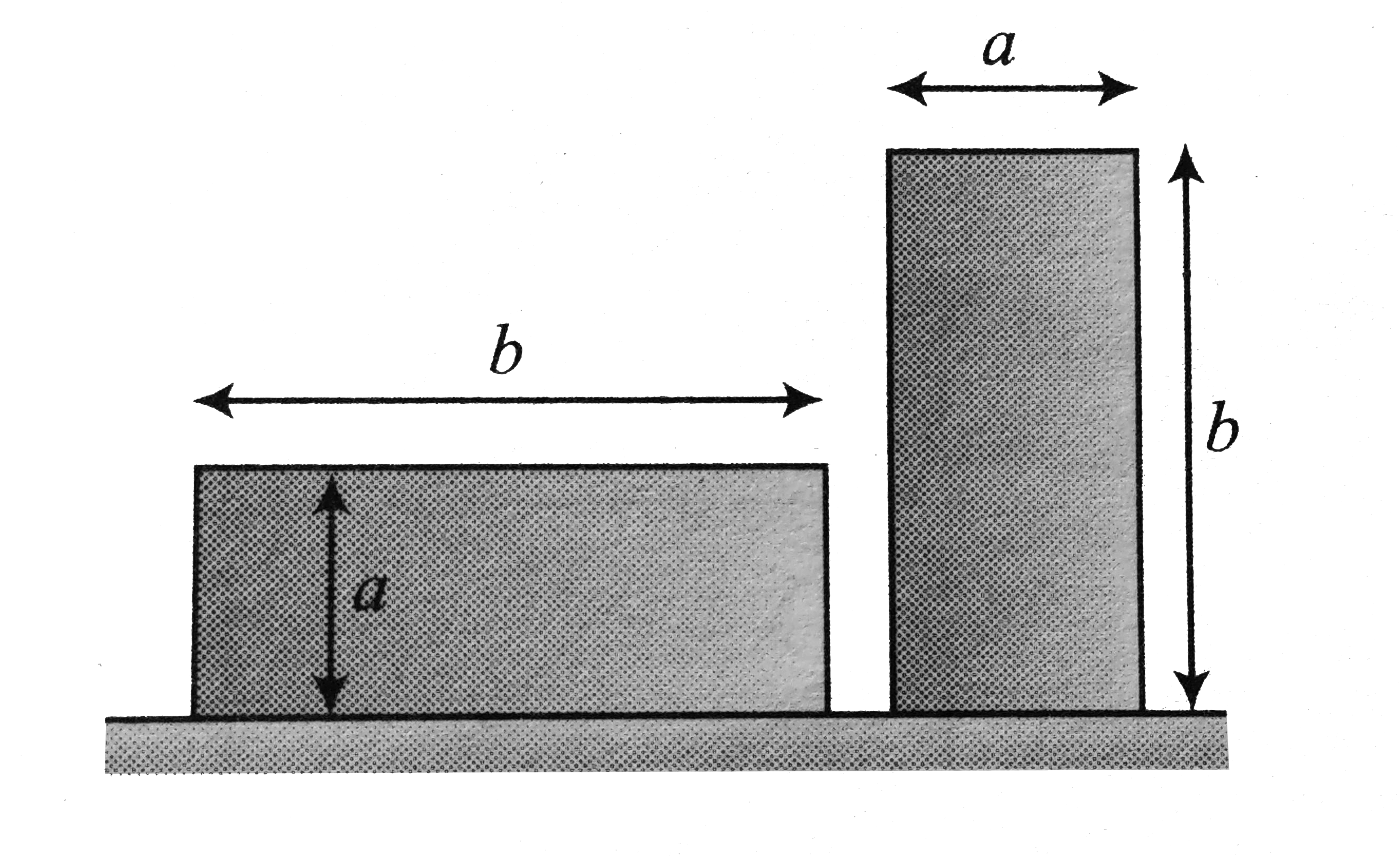
View SolutionA plate of mass m, length b, and breadth a is initially lying on a horizontal floor with length parallel to the floor and breath perpendicular to the floor. Find the work done to erect it on its breadth.
View SolutionA rod AB of length 2L and mass m is lying on a horizontal frictionless surface. A particle of same mass m travelling along the surface hits the rod at distance L2 from COM with a velocity v0 in a direction perpendicular to rod and sticks to it.
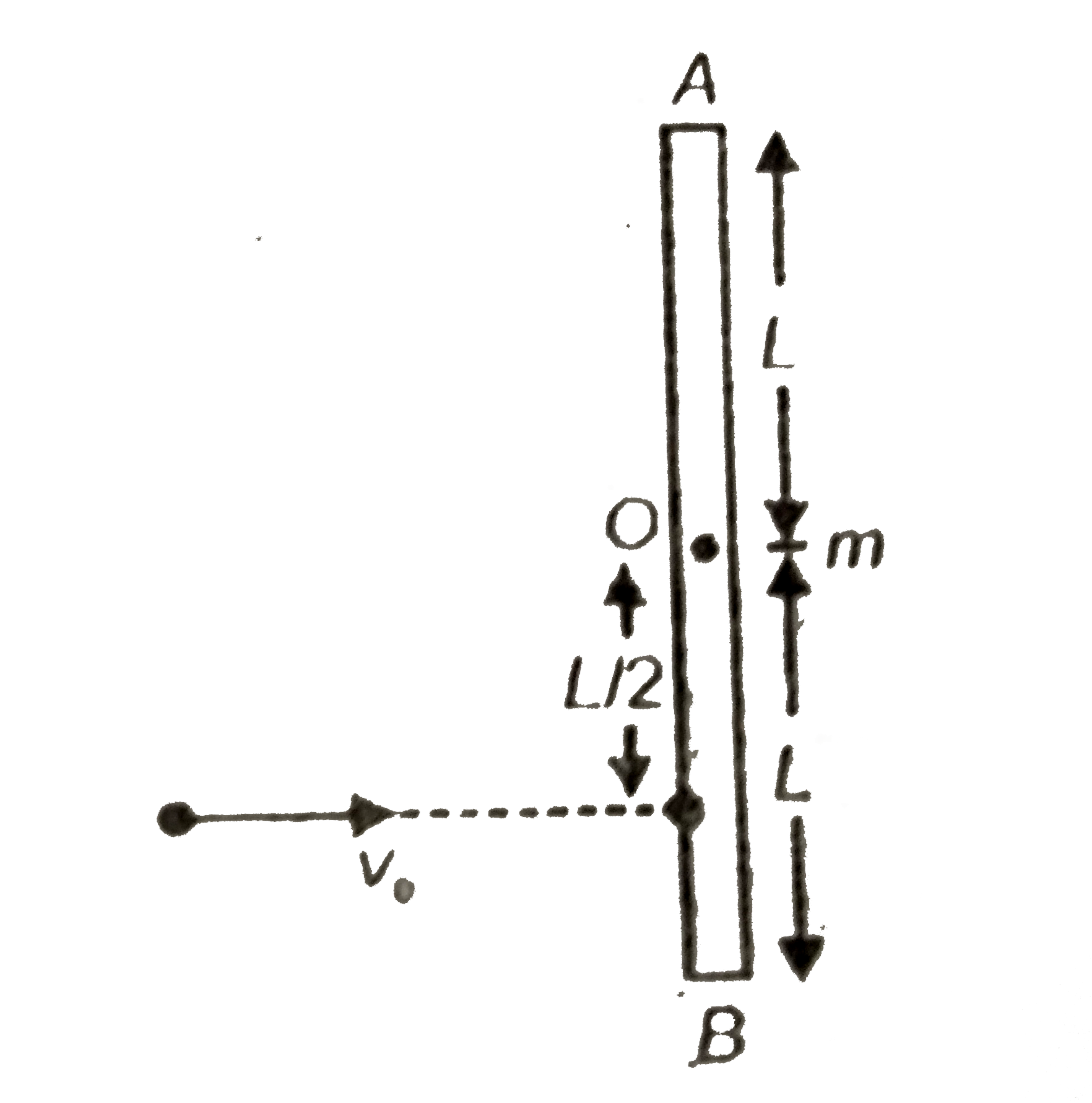
Angular velocity of rod just after collision is
View SolutionA rod AB of length 2L and mass m is lying on a horizontal frictionless surface. A particle of same mass m travelling along the surface hits the rod at distance L2 from COM with a velocity v0 in a direction perpendicular to rod and sticks to it.

Angular velocity of rod just after collision is
View Solution
A uniform rod of length L and mass m is free to rotate about a frictionless pivot at one end as shown in figure. The rod is held at rest in the horizontal position and a coin of mass m is placed at the free end. Now the rod is released The reaction on the coin immediately after the rod starts falling is
View Solution