Calculate the degree of freedom of diatomic molecule?
Non-steady flow
Compressible liquids
Streamlined flow
Turbulent flow
The correct Answer is:C
To calculate the degree of freedom of a diatomic molecule, we can follow these steps:
Step 1: Understand the Concept of Degree of Freedom
The degree of freedom (f) of a molecule refers to the number of independent ways in which the molecule can move. For a diatomic molecule, we need to consider both translational and rotational motions.
Step 2: Identify the Formula
The degree of freedom can be calculated using the formula:
f=3n−c
where:
- n is the number of molecules,
- c is the number of constraints.
Step 3: Determine the Values of n and c
For a diatomic molecule:
- At high temperatures, the number of degrees of freedom due to translational and rotational motion is n=2 (since it consists of two atoms).
- At high temperature, there are no constraints, so c=0.
Step 4: Calculate Degree of Freedom at High Temperature
Substituting the values into the formula:
f=3(2)−0=6
Thus, the degree of freedom at high temperature is 6.
Step 5: Consider Low Temperature Conditions
At low temperatures, the number of degrees of freedom remains n=2 (still a diatomic molecule), but there is a constraint due to the vibrational motion being frozen out. Here, c=1.
Step 6: Calculate Degree of Freedom at Low Temperature
Substituting the values into the formula:
f=3(2)−1=5
Thus, the degree of freedom at low temperature is 5.
Conclusion
- Degree of freedom at high temperature: 6
- Degree of freedom at low temperature: 5
The degree of freedom (f) of a molecule refers to the number of independent ways in which the molecule can move. For a diatomic molecule, we need to consider both translational and rotational motions.
The degree of freedom can be calculated using the formula:
f=3n−c
where:
- n is the number of molecules,
- c is the number of constraints.
For a diatomic molecule:
- At high temperatures, the number of degrees of freedom due to translational and rotational motion is n=2 (since it consists of two atoms).
- At high temperature, there are no constraints, so c=0.
Substituting the values into the formula:
f=3(2)−0=6
Thus, the degree of freedom at high temperature is 6.
At low temperatures, the number of degrees of freedom remains n=2 (still a diatomic molecule), but there is a constraint due to the vibrational motion being frozen out. Here, c=1.
Substituting the values into the formula:
f=3(2)−1=5
Thus, the degree of freedom at low temperature is 5.
- Degree of freedom at high temperature: 6
- Degree of freedom at low temperature: 5
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - B
23 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - C
15 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - TRY YOURSELF
41 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment(Section-D)
14 Videos
Similar Questions
A diatomic molecules has
View SolutionMention the degree of freedom for diatomic gas molecules without vibration.
View SolutionThe energy associated with each degree of freedom of a molecule
View SolutionCalculate the number of degrees of freedom of molecules of hydrogen in 1cc of hydrogen gas at NTP.
View SolutionWhat are degrees of freedom?
View SolutionThe number of degrees of freedom of a rigid diatomic molecule is :
View SolutionThe degrees of freedom of a triatomic gas is
View SolutionThe ratio (CpCv) for a gas is 1.29 . What is the degree of freedom of the molecules of this gas?
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
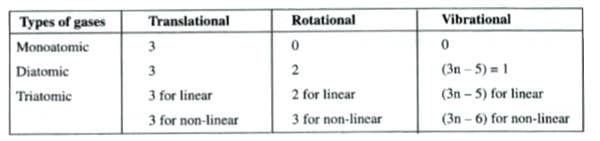
Where 'n' is atomicity of gas.
How many total degrees of freedom are present in H2 molecules in all types of motions ?
View SolutionA gas undergoes a process such that p∝1T. If the molar heat capacity for this process is C=33.24J/mol−K, find the degree of freedom of the molecules of the gas.
View Solution
