An open water tank has an orifice 10 cm below the surface of water. If there is no loss of energy, then what is the speed of efflux ? (Take g = 10 m s−2)
1.414 m s−1
2.5 m s−1
6.32 m s−1
3.7 m s−1
The correct Answer is:A
To find the speed of efflux from the orifice in the open water tank, we can use Torricelli's theorem, which states that the speed of efflux (V) of a fluid under the force of gravity through an orifice is given by the formula:
V=√2gh
where:
- g is the acceleration due to gravity,
- h is the height of the fluid above the orifice.
1. Identify the height (h):
The problem states that the orifice is 10 cm below the surface of the water. Therefore, the height h is 10 cm.
2. Convert height to meters:
Since we need to use SI units, we convert 10 cm to meters:
h=10cm=10×10−2m=0.1m
3. Substitute values into the formula:
We know g=10m/s2 and h=0.1m. Now we can substitute these values into the formula:
V=√2gh=√2×10×0.1
4. Calculate the expression inside the square root:
2×10×0.1=2
5. Take the square root:
V=√2m/s
6. Approximate the value of √2:
The approximate value of √2 is about 1.41. Thus:
V≈1.41m/s
Final Answer:
The speed of efflux is approximately 1.41m/s.
- g is the acceleration due to gravity,
- h is the height of the fluid above the orifice.
The problem states that the orifice is 10 cm below the surface of the water. Therefore, the height h is 10 cm.
Since we need to use SI units, we convert 10 cm to meters:
h=10cm=10×10−2m=0.1m
We know g=10m/s2 and h=0.1m. Now we can substitute these values into the formula:
V=√2gh=√2×10×0.1
2×10×0.1=2
V=√2m/s
The approximate value of √2 is about 1.41. Thus:
V≈1.41m/s
The speed of efflux is approximately 1.41m/s.
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - B23videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - C15videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - TRY YOURSELF41videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)26videos
MAGNETISM AND MATTER
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment(Section-D)14videos
MECHANICAL PROPERTIES OF SOLIDS
Similar Questions
A tank containing water has an orifice 2 m below the surface of water. If there is no wastage of energy. Find the speed of discharge.
View SolutionThe velocity of efflux of a fluid through an orifice 15 cm below the fluid surface (which is open to the atmosphere) is [Take g = 10 m s−2]
View SolutionA water tank is placed on a platform of height 5 m high and there is an orifice near the bottom in the wall of tank at 5 m below the level of water. Find the speed with which water will hit the ground [Take g = 10 m/s2 ]
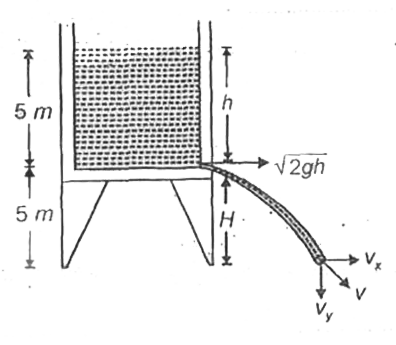 View Solution
View SolutionThe surface of water in a water tank on the top of a house is 4 m above the tap level. Find the pressure of water at the tap, when the tap is closed. Is it necessary to specify that the tap is closed? Take g=10ms−2
View SolutionA ball is projected vertically up with speed 20 m/s. Take g=10m/s2
View SolutionA tank is filled by water (ρ=103kg/m3). A small hole is made in the side wall of the tank at depth 10 m below water surface. A water jet emerges horizontal from the hole and falls at horizontal distance R from it. The amount of extra pressure (in terms of atmospheric pressure) that must be applied on the water surface, so that range becomes 3R on the on the ground will be (cross section area of hole is negligible and 1 atm = 105 Pa, g = 10 m/s2)
View SolutionA ball is throw vertically upward. It has a speed of 10m/s when it has reached on half of its maximum height. How high does the ball rise ? (Taking g=10m/s2).
View SolutionA water tank has a hole at a distance of 7 m from free water surface. Find the velocity of water through the hole. If the radius of the hole is 2 mm what is the rate of flow of water?
View SolutionAn open vessel contains water upto height 50 cm in it. What is the velocity of efflux through an orifice at height 30 cm above the bottom level ? [Take g = 10 m/s2]
View SolutionA water tank is 20 m deep . If the water barometer reads 10 m at the surface , then what is the pressure at the bottom of the tank in atmosphere ?
View Solution

