A body of mass 8 kg performs S.H.M. of amplitude 60 cm. The restoring force is 120 N, when the displacement is 60 cm. The time period is
0.628s
1.256s
1.884s
2.512s
The correct Answer is:2
To solve the problem step by step, we can follow these instructions:
Step 1: Identify the given values
- Mass (m) = 8 kg
- Amplitude (A) = 60 cm = 0.6 m (convert to meters)
- Restoring force (F) = 120 N
- Displacement (x) = 60 cm = 0.6 m (convert to meters)
Step 2: Use the formula for restoring force in SHM
The restoring force in Simple Harmonic Motion (SHM) is given by:
F=−Kx
Where:
- K is the force constant (spring constant)
- x is the displacement
Step 3: Calculate the force constant (K)
From the equation F=Kx, we can rearrange it to find K:
K=Fx
Substituting the values:
K=120N0.6m=200N/m
Step 4: Use the formula for the time period (T) of SHM
The time period T of SHM is given by:
T=2π√mK
Step 5: Substitute the values into the time period formula
Substituting the values of mass and force constant:
T=2π√8kg200N/m
Step 6: Simplify the expression
Calculating the fraction:
8200=0.04
Now, take the square root:
√0.04=0.2
Step 7: Calculate the time period
Now substitute back into the time period formula:
T=2π×0.2
T=0.4π
Step 8: Calculate the numerical value
Using π≈3.14:
T≈0.4×3.14≈1.256s
Final Answer
The time period T is approximately 1.256 seconds.
---
- Mass (m) = 8 kg
- Amplitude (A) = 60 cm = 0.6 m (convert to meters)
- Restoring force (F) = 120 N
- Displacement (x) = 60 cm = 0.6 m (convert to meters)
The restoring force in Simple Harmonic Motion (SHM) is given by:
F=−Kx
Where:
- K is the force constant (spring constant)
- x is the displacement
From the equation F=Kx, we can rearrange it to find K:
K=Fx
Substituting the values:
K=120N0.6m=200N/m
The time period T of SHM is given by:
T=2π√mK
Substituting the values of mass and force constant:
T=2π√8kg200N/m
Calculating the fraction:
8200=0.04
Now, take the square root:
√0.04=0.2
Now substitute back into the time period formula:
T=2π×0.2
T=0.4π
Using π≈3.14:
T≈0.4×3.14≈1.256s
The time period T is approximately 1.256 seconds.
Topper's Solved these Questions
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT (SECTION-B )
25 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT ( SECTION-C )
11 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - Try Yourself
86 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
4 Videos
Similar Questions
A body of mass 36 g moves with S.H.M. of amplitude A = 13 cm and time period T = 12 s. At time t = 0, the displacement x is + 13 cm. The shortest time of passage from x = + 6.5 cm to x = - 6.5 cm is
View SolutionThe system shown in the figure can move on a smooth surface. They are initially compressed by 6cm and then released, then choose the correct options.
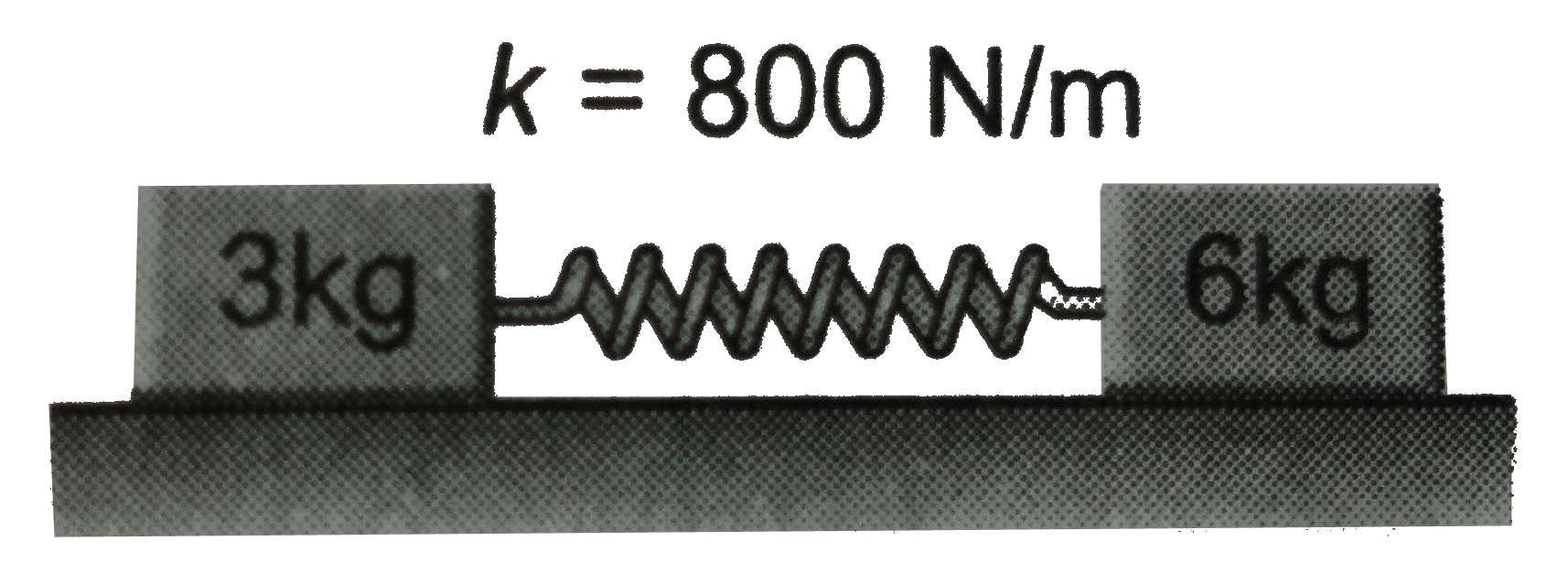
(a) The system performs, SHM with time period π10s
(b) The block of mass 3kg perform SHM with amplitude 4cm
( c) The block of mass 6kg will have maximum momentum of 2.40kg−m/s
(d) The time periods of two blocks are in the ratio of 1:√2
View Solution
Knowledge Check
The total energy of the body executing S.H.M. is E. Then the kinetic energy when the displacement is half of the amplitude is
AE/2BE/4C3E/4D√3/4E
A horizontal platform with a small block of mass 1 kg kept on it performs vertical SHM of amplitude 1 cm. The block does not lose contact with the platform anywhere. The minimum possible time period of the platform
View SolutionBlock A of mass m is performing SHM of amplitude a. Another block B of mas m is gently placed on A when it passes through mean position and B sticks to A. Find the time period and amplitude of new SHM.
View SolutionA block of mass 5 kg executes simple harmonic motion under the restoring force of a spring. The amplitude and the time period of the motion are 0.1 m and 3.14 s respectively. Find the maximum force exerted by the spring on the block.
View SolutionA particle of mass 2 kg executing SHM has amplitude 10 cm and time period is 1 s.Find (i) the angular frequency (ii) the maximum speed (ii) the maximum acceleration (iv) the maximum restoring force (v) the speed when the displacement from the mean position is 8 cm (vi) the speed after 112 s the particle was at the extreme position (vii) the time taken by the particle to go directly from its mean position to half the amplitude (viii) the time taken by the particle to go directly from its exterme position to half the amplitude.
View SolutionAn object performs SHM of amplitude 5 cm and time period 4 s. If timing is started when the object is at the centre of the oscillation i.e., x=0 then calculate.
(i) Frequency of oscillation.
(ii) The displacement at 0.5 S.
View SolutionA particle of mass 200g executes a simple harmonic motion. The restoring force is provided by a spring of spring constant 80N/m. Find the time period.
View SolutionA body of mass 4 kg is executing shm of amplitude 0.4 m and period 2 s. Find (i) the maximum restoring force and (ii) the restoring force at a distance of 0.1 m from extreme position.
View Solution
