Question

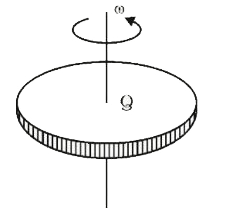
A string has a linear mass density $\mu$. The ends of the string are joined to form a closed loop and is given the shape of a circular ring of radius R. this ring is now rotated about its axis with an angular velocity $\omega$
Q. The tension developed in the string is

Options

A. `muomegaR^(3)`
B. `2muomega^(2)R^(2)`
C. `muomega^(2)R^(2)`
D. `mu^(2)omega^(2)R^(2)`

Answer

C

Explanation

To find the tension developed in a string that is formed into a circular ring and rotated about its axis, we can follow these steps:

Step 1: Understand the Physical Setup
We have a string with a linear mass density \( \mu \) that is shaped into a circular ring of radius \( R \). The ring is rotating about its axis with an angular velocity \( \omega \).

Step 2: Express Linear Mass Density
The linear mass density \( \mu \) can be expressed as:
\( \mu = \frac{m}{2\pi R} \)
where \( m \) is the total mass of the string.

Step 3: Consider a Small Segment of the Ring
Take a small segment of the ring with a central angle \( d\theta \). The length of this segment is:
\( dL = R \, d\theta \)
The mass of this small segment \( dm \) can be expressed as:
\( dm = \mu \, dL = \mu R \, d\theta \)

Step 4: Analyze Forces Acting on the Segment
When the ring rotates, tension \( T \) in the string acts along the segment. The tension has two components at the ends of the segment:
- Horizontal component: \( T \cos\left(\frac{d\theta}{2}\right) \)
- Vertical component: \( T \sin\left(\frac{d\theta}{2}\right) \)

Step 5: Centripetal Force Requirement
For the ring to maintain its circular motion, the net inward force (centripetal force) must equal the required centripetal force for the mass \( dm \):
\( \text{Centripetal Force} = \frac{dm \cdot v^2}{R} \)
where \( v = \omega R \) is the linear velocity of the mass element.

Step 6: Set Up the Equation
The net vertical component of the tension providing the centripetal force is:
\( 2T \sin\left(\frac{d\theta}{2}\right) = \frac{dm \cdot v^2}{R} \)
Substituting \( dm \) and \( v \):
\( 2T \sin\left(\frac{d\theta}{2}\right) = \frac{\mu R \, d\theta \cdot (\omega R)^2}{R} \)
This simplifies to:
\( 2T \sin\left(\frac{d\theta}{2}\right) = \mu \omega^2 R \, d\theta \)

Step 7: Approximate for Small Angles
For small angles, we can use the approximation:
\( \sin\left(\frac{d\theta}{2}\right) \approx \frac{d\theta}{2} \)
Thus, the equation becomes:
\( 2T \left(\frac{d\theta}{2}\right) = \mu \omega^2 R \, d\theta \)
This simplifies to:
\( T = \mu \omega^2 R \)

Conclusion
The tension developed in the string is:
\( \boxed{T = \mu \omega^2 R} \)