A charged particle of charge 1 mC and mass 2g is moving with a speed of 5m/s in a uniform magnetic field of 0.5 tesla. Find the maximum acceleration of the charged particle
To find the maximum acceleration of the charged particle, we can follow these steps:
Step 1: Identify the given values
- Charge of the particle, q=1mC=1×10−3C
- Mass of the particle, m=2g=2×10−3kg
- Speed of the particle, v=5m/s
- Magnetic field strength, B=0.5T
Step 2: Calculate the maximum magnetic force acting on the charged particle
The magnetic force F acting on a charged particle moving in a magnetic field is given by the formula:
F=qvBsinθ
The maximum force occurs when sinθ=1:
Fmax=qvB
Substituting the values:
Fmax=(1×10−3C)×(5m/s)×(0.5T)
Fmax=1×10−3×5×0.5=2.5×10−3N=25×10−4N
Step 3: Calculate the maximum acceleration
Using Newton's second law, the acceleration a is given by:
a=Fm
Substituting the maximum force and mass:
amax=Fmaxm=25×10−4N2×10−3kg
amax=25×10−42×10−3=252×10−1=12.5×10−1m/s2=1.25m/s2
Final Answer
The maximum acceleration of the charged particle is 1.25m/s2.
---
- Charge of the particle, q=1mC=1×10−3C
- Mass of the particle, m=2g=2×10−3kg
- Speed of the particle, v=5m/s
- Magnetic field strength, B=0.5T
The magnetic force F acting on a charged particle moving in a magnetic field is given by the formula:
F=qvBsinθ
The maximum force occurs when sinθ=1:
Fmax=qvB
Fmax=(1×10−3C)×(5m/s)×(0.5T)
Fmax=1×10−3×5×0.5=2.5×10−3N=25×10−4N
Using Newton's second law, the acceleration a is given by:
a=Fm
amax=Fmaxm=25×10−4N2×10−3kg
amax=25×10−42×10−3=252×10−1=12.5×10−1m/s2=1.25m/s2
The maximum acceleration of the charged particle is 1.25m/s2.
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Illustration
14 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Try Yourself
27 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION D
16 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
Similar Questions
A charge particle of charge q and mass m is moving with velocity v as shown in fig In a uniform magnetic field B along -ve z-direction.Select the correct alternative (s).

View SolutionA charged particle of specific charge (i.e.,Charge per unit mass 0 0.2 C/kg has velocity 2ˆi−3ˆjms−1 at some instant in a uniform magnetic field 5ˆi+2ˆj (tesla).Find the acceleration of the particle at this instant
View SolutionA charged particle of mass m is moving with a speed u in a circle of radius r. If the magnetic field induction at the centre is B, the charge on the particle is
View SolutionA charged particle of mass m and charge q describes circular motion of radius r in a uniform magnetic field of strength B the frequency of revolution is
View SolutionA charged particle having charge q is moving in a uniform magnetic field B parallel to the lines of field force, the magnetic force acting on a charge will be qvB
View SolutionTwo charged particles have charges and masses in the ratio 2:3 and 1:4 respectively. If they enter a uniform magnetic field and move with the same velocity then the ratio of their respective time periods of revolutions is
View SolutionA positive charge particle with charge q is moving with speed v in a region of uniform magnetic field B at the instant shown in figure. An external electric field so to be applied so that the charged particle follows a straight line path. The magnitude and direction of electric field required are respectively.
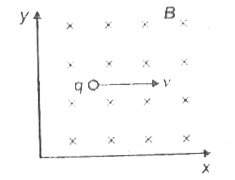
View SolutionWhen would a moving charged particle travel undeviated in a uniform magnetic field ?
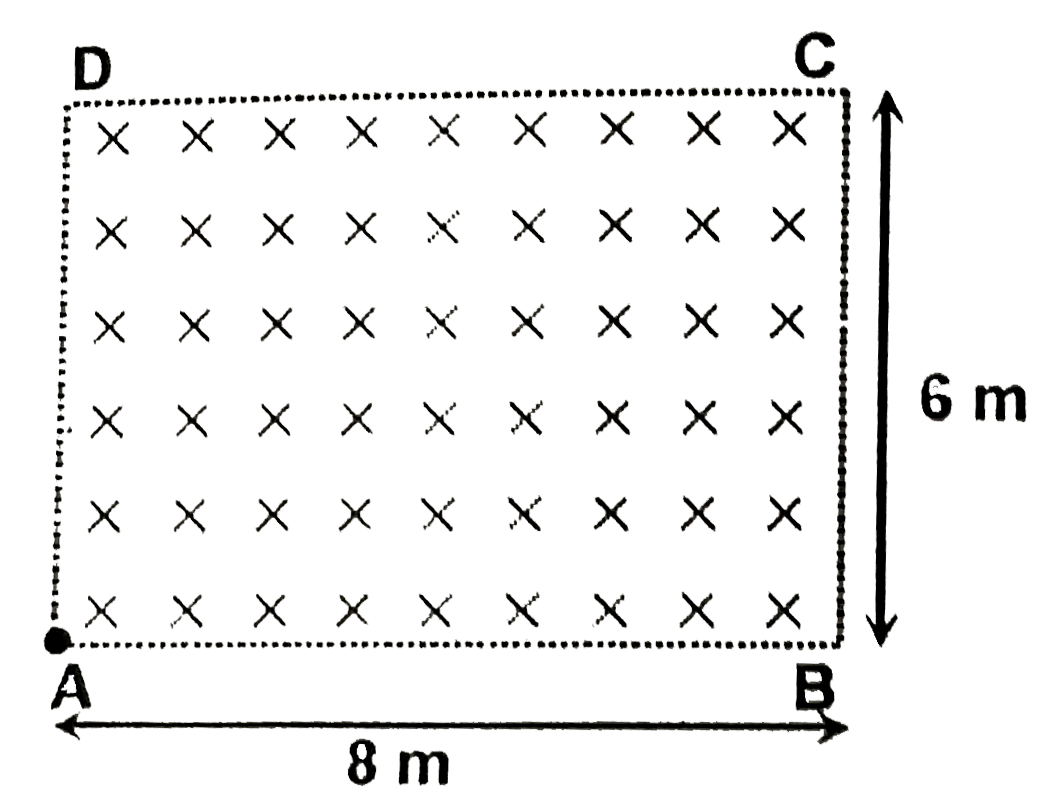
View SolutionA charge particle of mass m=5gm and charge q=12μCenters along AB at point A in a uniform magnetic field of magnitude B=100 Tesla existing in a rectangular region of size (8m×6m) and into the plane of paper as shown in figure. The particle leaves the region at 'C'. The speed of the charge particle (in m/s) as it leaves the region is
View SolutionWhen does a moving charged particle not experience any force while moving through a uniform magnetic field ?
View Solution
