A charged particle of mass m is moving with a speed u in a circle of radius r. If the magnetic field induction at the centre is B, the charge on the particle is
4πr2Bμ0u
2πr2Bμ0u
muBr
2muBr
The correct Answer is:A
To solve the problem, we need to find the charge q on a particle of mass m that is moving in a circular path of radius r with a speed u, while experiencing a magnetic field B at the center of the circle.
1. Identify the Current:
The current I due to the moving charge can be expressed as:
I=qT
where T is the time period of the motion.
2. Calculate the Time Period:
The time period T for a particle moving in a circular path is given by the circumference of the circle divided by the speed:
T=2πru
3. Substitute Time Period into Current:
Now substituting T into the expression for current:
I=qT=q2πru=qu2πr
4. Use the Magnetic Field Formula:
The magnetic field B at the center of a circular loop carrying current I is given by:
B=μ0I2r
where μ0 is the permeability of free space.
5. Substitute Current into Magnetic Field Equation:
Substitute the expression for current I into the magnetic field equation:
B=μ02r⋅qu2πr
Simplifying this gives:
B=μ0qu4πr2
6. Rearranging to Find Charge q:
Now, we can rearrange this equation to solve for q:
q=4πr2Bμ0u
Final Answer:
The charge q on the particle is given by:
q=4πr2Bμ0u
The current I due to the moving charge can be expressed as:
I=qT
where T is the time period of the motion.
The time period T for a particle moving in a circular path is given by the circumference of the circle divided by the speed:
T=2πru
Now substituting T into the expression for current:
I=qT=q2πru=qu2πr
The magnetic field B at the center of a circular loop carrying current I is given by:
B=μ0I2r
where μ0 is the permeability of free space.
Substitute the expression for current I into the magnetic field equation:
B=μ02r⋅qu2πr
Simplifying this gives:
B=μ0qu4πr2
Now, we can rearrange this equation to solve for q:
q=4πr2Bμ0u
The charge q on the particle is given by:
q=4πr2Bμ0u
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment Section C (Objective Type Questions (More than one option are correct)
14 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment Section D (Linked Comprehension Type Questions)
10 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment (Section A) Objective Type Questions (One option is correct)
55 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION D
16 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
Similar Questions
A particle of mass m is being circulated on a vertical circle of radius r. If the speed of particle at the highest point be v, then
View SolutionA charged particle of mass m and charge q travels on a circular path of radius r that is perpendicular to a magnetic field B. The time takeen by the particle to complete one revolution is
View SolutionFind the condition under which the charged particles moving with different speeds in the presence of electric and magnetic field vectors can be used to select charged particles of a particular speed.
View SolutionA particle initially moving towards south in a vertically downward magnetic field is deflected toward the east. What is the sign of the charge on the particle?
View SolutionA charged particle of charge 1 mC and mass 2g is moving with a speed of 5m/s in a uniform magnetic field of 0.5 tesla. Find the maximum acceleration of the charged particle
View SolutionA charged particle of charge 1 mC and mass 2g is moving with a speed of 5m/s in a uniform magnetic field of 0.5 tesla. Find the maximum acceleration of the charged particle
View SolutionA positive charge particle with charge q is moving with speed v in a region of uniform magnetic field B at the instant shown in figure. An external electric field so to be applied so that the charged particle follows a straight line path. The magnitude and direction of electric field required are respectively.
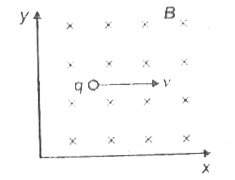
View SolutionA charged particle of mass m and charge q describes circular motion of radius r in a uniform magnetic field of strength B the frequency of revolution is
View SolutionA particle of mass m having a charge q enters into a circular region of radius R with velocity v directed towards the centre. The strength of magnetic field is B. Find the deviation in the path of the particle.
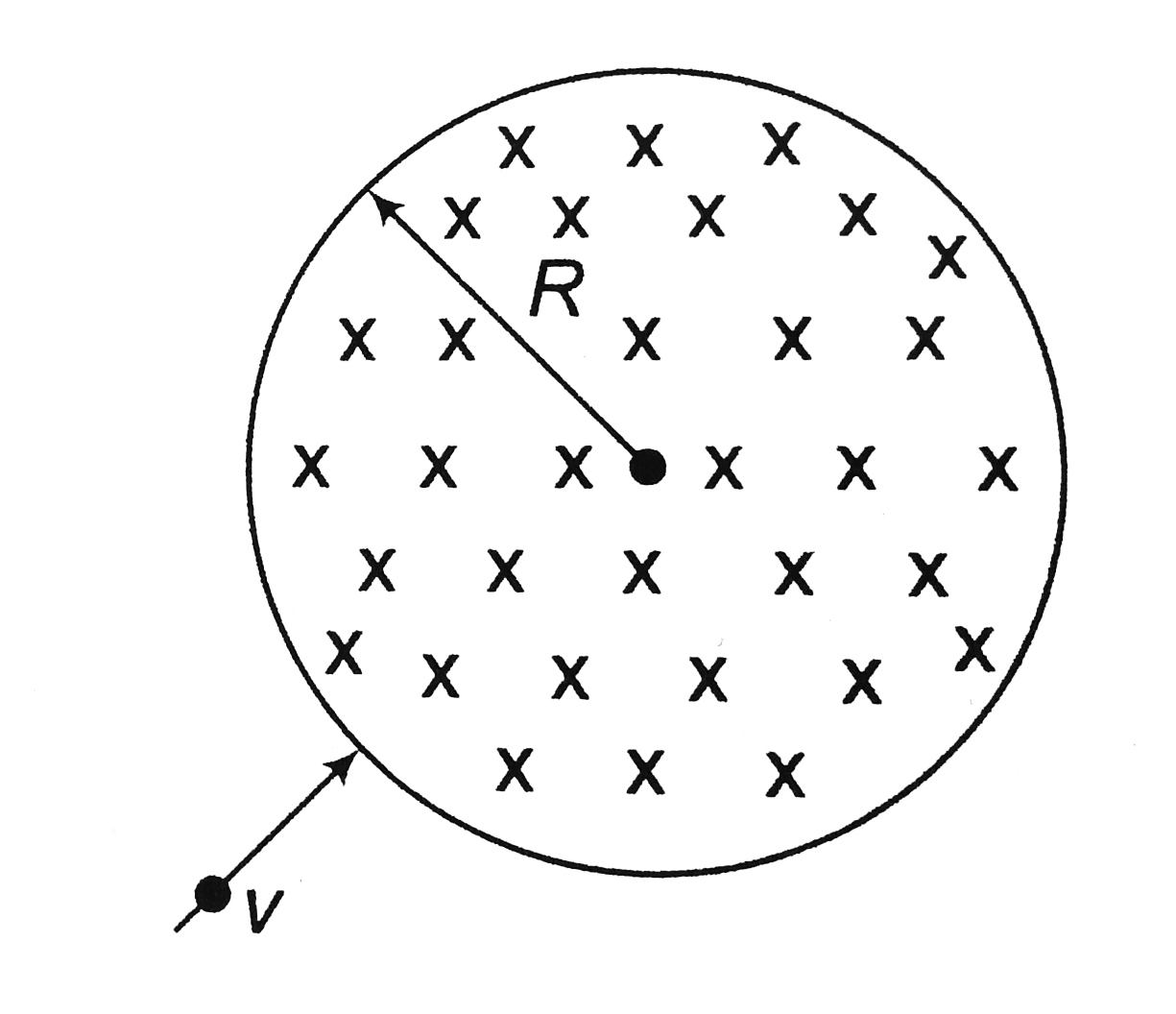
View SolutionA particle having a charge of 10.0μC and mass 1μg moves in a circle of radius 10 cm under the influence of a magnetic field of induction 0.1T. When the particle is at a point P, a uniform electric field is switched on so that the particle starts moving along the tangent with a uniform velocity. The electric field is
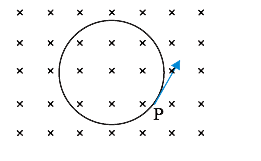
View Solution
