A disc is placed on the surface of pond filled with liquid of refractive index 53. A source of light is placed 4m below the surface of liquid. Calculate the minimum area of the disc so that light does not come out of liquid.
To solve the problem, we need to determine the minimum area of the disc that will prevent light from escaping the liquid. This involves calculating the critical angle for total internal reflection and the radius of the circular area from which light can escape.
1. Identify the Given Data:
- Depth of the light source below the surface of the liquid, d=4m
- Refractive index of the liquid, μ=53
2. Calculate the Critical Angle:
The critical angle θc for total internal reflection can be calculated using the formula:
sin(θc)=1μ
Substituting the value of μ:
sin(θc)=153=35
Therefore, the critical angle θc is:
θc=arcsin(35)
3. Determine the Radius of the Circular Area:
The radius r of the circular area from which light can escape is given by:
r=d√μ2−1
First, calculate μ2:
μ2=(53)2=259
Now, calculate μ2−1:
μ2−1=259−1=259−99=169
Now, substitute d and μ2−1 into the radius formula:
r=4√169=443=3m
4. Calculate the Area of the Disc:
The area A of the circular disc is given by:
A=πr2
Substituting the value of r:
A=π(3)2=9π≈28.26m2
5. Final Result:
The minimum area of the disc so that light does not come out of the liquid is approximately:
A≈28.26m2
- Depth of the light source below the surface of the liquid, d=4m
- Refractive index of the liquid, μ=53
The critical angle θc for total internal reflection can be calculated using the formula:
sin(θc)=1μ
Substituting the value of μ:
sin(θc)=153=35
Therefore, the critical angle θc is:
θc=arcsin(35)
The radius r of the circular area from which light can escape is given by:
r=d√μ2−1
First, calculate μ2:
μ2=(53)2=259
Now, calculate μ2−1:
μ2−1=259−1=259−99=169
Now, substitute d and μ2−1 into the radius formula:
r=4√169=443=3m
The area A of the circular disc is given by:
A=πr2
Substituting the value of r:
A=π(3)2=9π≈28.26m2
The minimum area of the disc so that light does not come out of the liquid is approximately:
A≈28.26m2
Similar Questions
A disc is placed on a surface of pond which has refractive index 35 . A source of light is placed 4 m below the surface of liquid. The minimum radius of disc will be so light is not coming out
View SolutionA point source of light is placed 4 m below the surface of water of refractive index 53. The minimum diameter of a disc, which should be placed over the source, on the surface of water to cut off all light coming out of water is
View Solution
Knowledge Check
- Question 1 - Select One
A point source of light is placed at a depth of h below the surface of water of refractive index μ. A floating opaque disc is placed on the surface of water so that light from the source is not visible from the surface. The minimum diameter of the disc is
A2h(μ2−1)1/2B2h(μ2−1)1/2Ch2(μ2−1)1/2Dh(μ2−1)1/2
A point source of light is placed at a depth h=0.5 m below the surface of a liquid (μ=54) , Then , the fraction of light energy that escape directly from the liquid surface is
View SolutionA thin equiconvex lens of refractive index 3/2 is placed on a horizontal plane mirror as shown in figure. The space between the lens and the mirror is filled with a liquid of refractive index 4/3 . It is found that when a point object is placed 15 cm above the lens on its priincipal axis, the object coincides with its own image.
Q. If another liquid is filled instead of water, the object and the image coincide at a distance 25 cm from the lens.
Calculate the refractive index of the liquid.View SolutionA point source is placed at a depth h below the surface of water (refractive index =μ). The medium above the surface area of water is air from water.
View SolutionA transparent solid cube of side 'a' has refractive index 3/2. A point source of light is embedded in it at its centre. Find the minimum area of the surface of the cube which must be painted black so that the source is not visible from outside.
View SolutionA point source of light S is placed at the bottom of a vessel containg a liquid of refractive index 5/3. A person is viewing the source from above the surface. There is an opaque disc of radius 1cm floating on the surface. The centre of the disc lies vertically above the source S. The liquid from the vessel is gradually drained out through a tap. What is the maximum height of the liquid for which the source cannot at all be seen from above?
View SolutionA jar of height h is filled wih a transparent liquid of refractive index μ, Fig. At the centre of the jar on the botom surface is a dot. Find the minimum diameter of a disc, such that when placed on the top surface symmetrically about the centre, the dot is invisible.
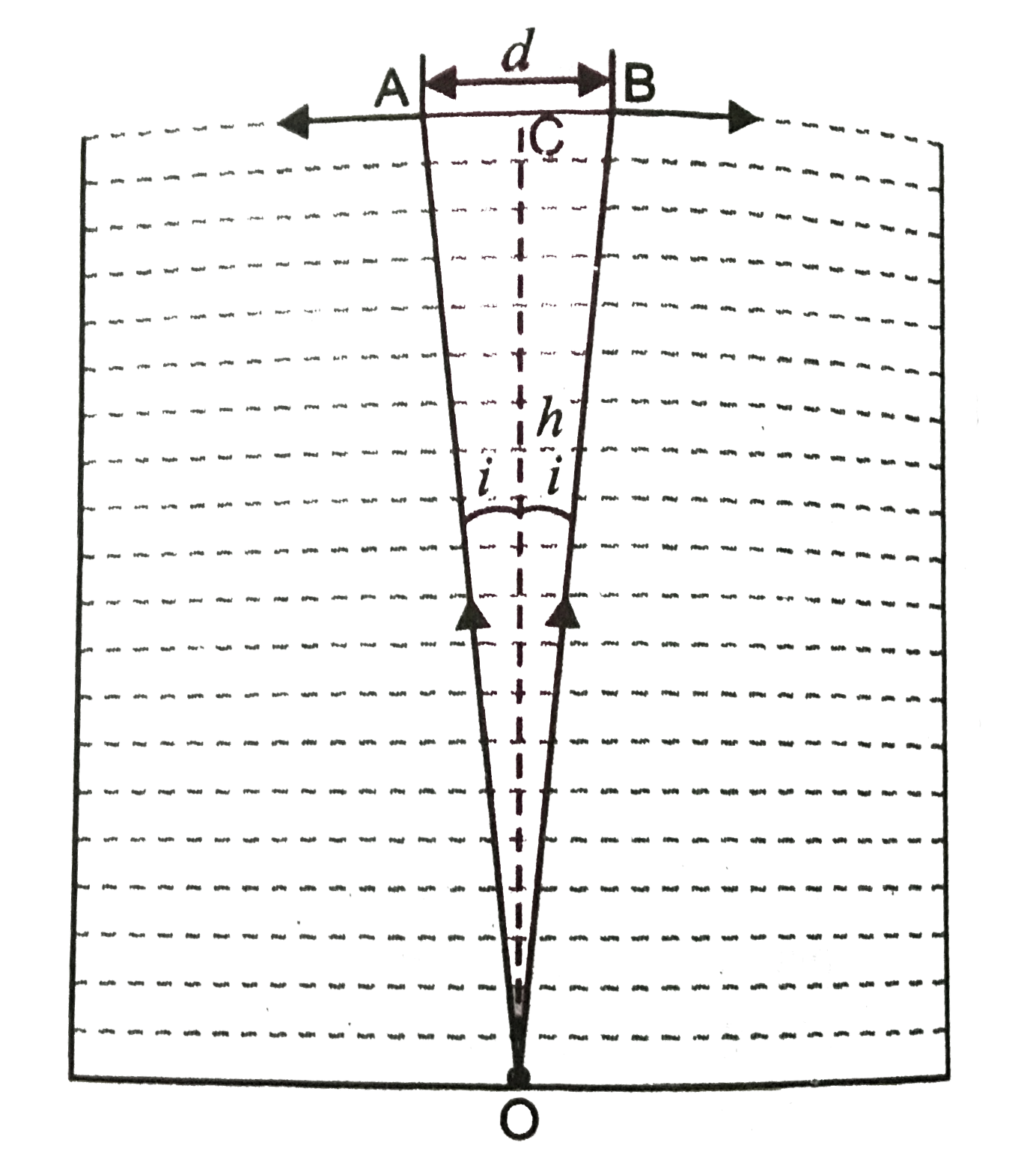 .View Solution
.View SolutionA small coin is resting on the bottom of a beaker filled with a liquid. A ray of light from the coin travels up to the surface of the liquid and moves along its surface (see figure ).
How fast is the light travelling in the liquid ?
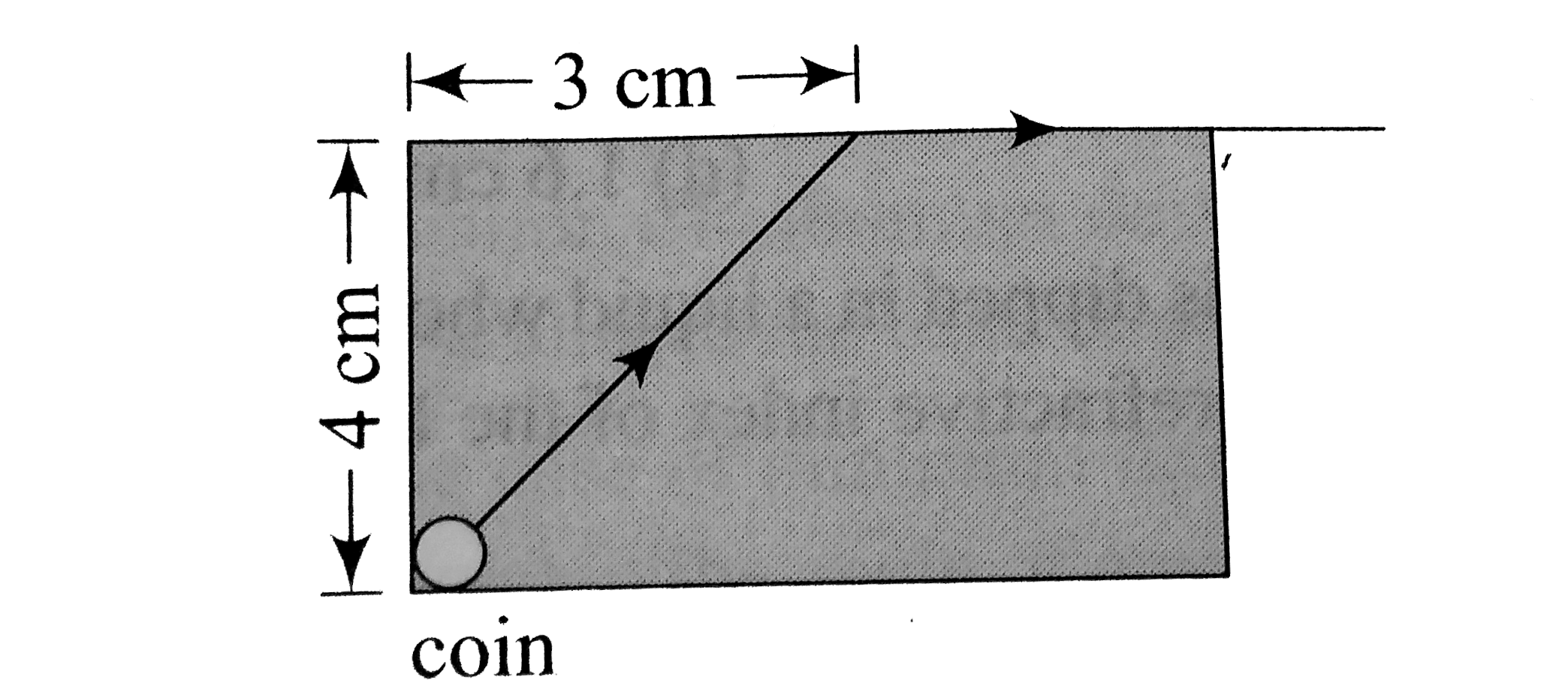 View Solution
View Solution

