Question

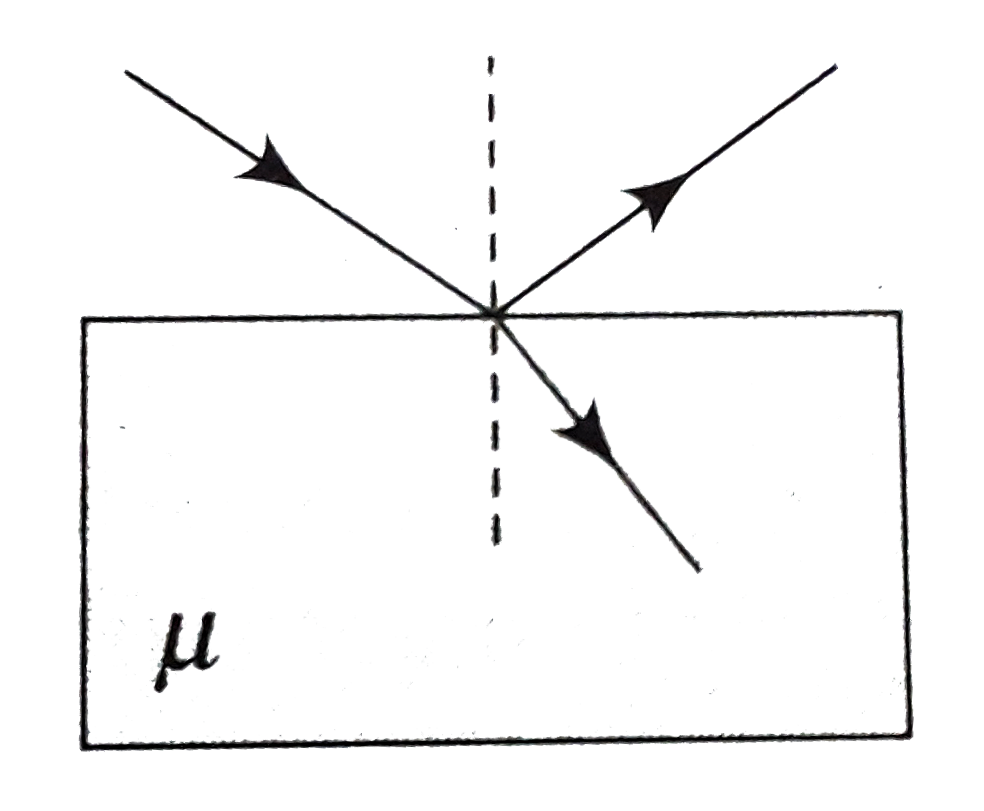
A light ray is travelling from air to glass. True reflected and refracted rays are perpendicular to each other. If the angle of incidence in air is I the refractive index of glass is

Options

A. sin i
B. cos i
C. tan i
D. cot i

Answer

C

Explanation

To solve the problem, we will follow these steps:

Step 1: Understand the situation
We have a light ray traveling from air into glass. The angle of incidence in air is \( I \), and we know that the reflected ray and the refracted ray are perpendicular to each other.

Step 2: Draw the diagram
Draw a diagram showing the incident ray, reflected ray, and refracted ray. Label the angle of incidence \( I \) and the angle of reflection (which will also be \( I \) due to the law of reflection).

Step 3: Determine the angles
Since the reflected and refracted rays are perpendicular, we can express this relationship mathematically. If we denote the angle of refraction as \( r \), we have:
\( \text{Angle between reflected and refracted rays} = 90^\circ \)
Thus, we can write:
\( r + 90^\circ = 90^\circ + I \implies r = 90^\circ - I \)

Step 4: Apply Snell's Law
Using Snell's Law, which states:
\( \mu_1 \sin I = \mu_2 \sin r \)
where \( \mu_1 \) is the refractive index of air (approximately 1), and \( \mu_2 \) is the refractive index of glass. We can substitute the known values:
\( 1 \cdot \sin I = \mu_2 \cdot \sin(90^\circ - I) \)

Step 5: Simplify using trigonometric identities
Using the identity \( \sin(90^\circ - I) = \cos I \), we can rewrite the equation:
\( \sin I = \mu_2 \cdot \cos I \)

Step 6: Solve for the refractive index
Rearranging the equation gives:
\( \mu_2 = \frac{\sin I}{\cos I} \)
This can be simplified to:
\( \mu_2 = \tan I \)

Conclusion
Thus, the refractive index of glass is given by:
\( \mu = \tan I \)

Final Answer
The refractive index of glass is \( \tan I \).

---