A cylindrical bar magnet is kept along the axis of a circular coil. If the magnent is rotated about its axis, then
A current will be induced in the coil
No current will be induced in the coil
An emf and a current both will be induced in the coil
None of these
The correct Answer is:B
Step by step video & image solution for A cylindrical bar magnet is kept along the axis of a circular coil. If the magnent is rotated about its axis, then by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - B)22videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assignment (SECTION - C)10videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Try Yourself42videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - comprehension3videos
ELECTRIC CHARGES AND FIELDS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT SECTION - D Assertion-Reason Type Questions25videos
ELECTROMAGNETIC WAVES
Similar Questions
On account of the earth rotating about its axis
View SolutionIn an experiment, a magnet with its magnetic moment along the axis of circular coil and directed towards the coil, is withdrawn away from the coil and parallel to itself. The current in the coil, as seen by the withdrawing magnet, is
View SolutionFigure shows a magnet suspended at the lower end of a spring while its length lies along the axis of a fixed circular conducting coil. The magnet is made to oscillate,
Deflection in the galvanometer is
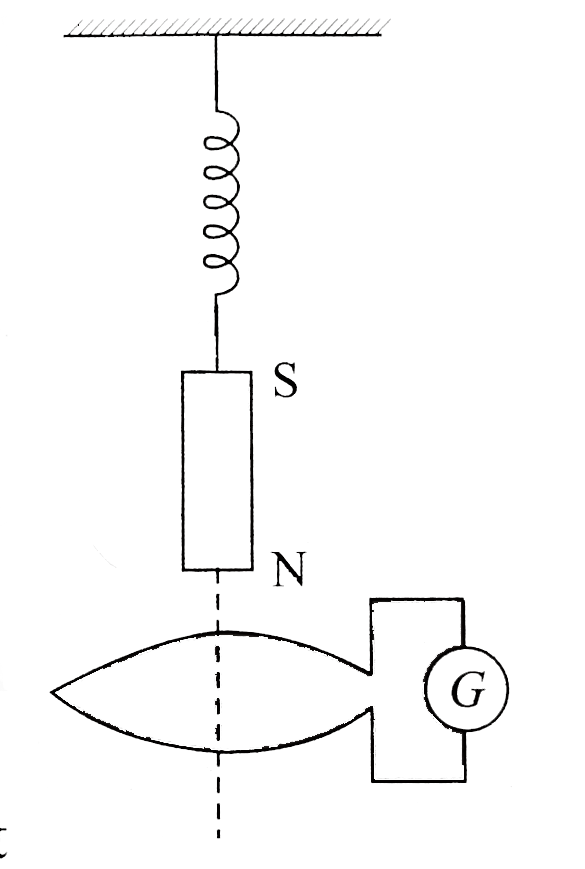 View Solution
View SolutionA bar magnet is freel falling along the axis of a circular loop as shown in Figure. State whether its acceleration a is equal to greater than or less than the acceleration due to gravity g.
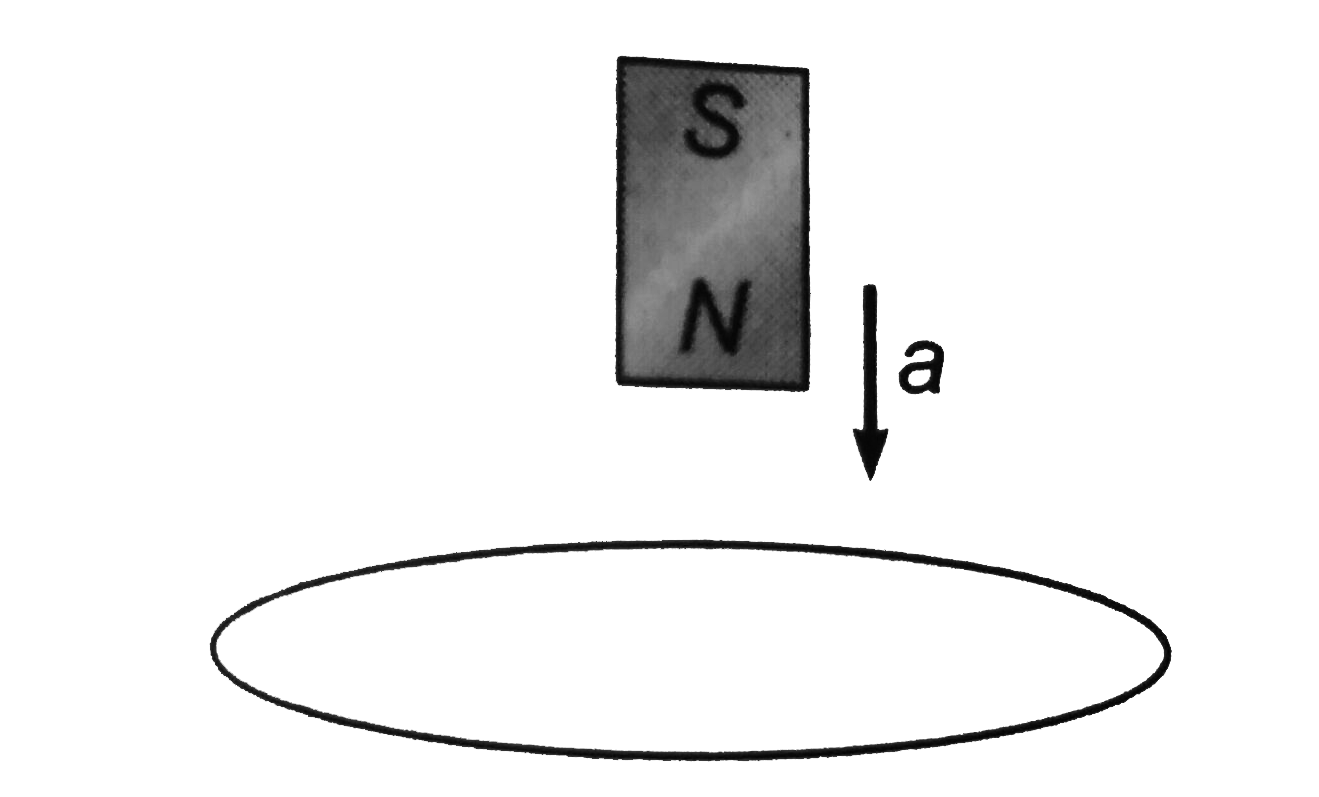 View Solution
View SolutionA cylindrical bar magnet is rotated about its axis (Figure). A wire is connect from the axis and is made to touch the cylindrical surface through a contact. Then
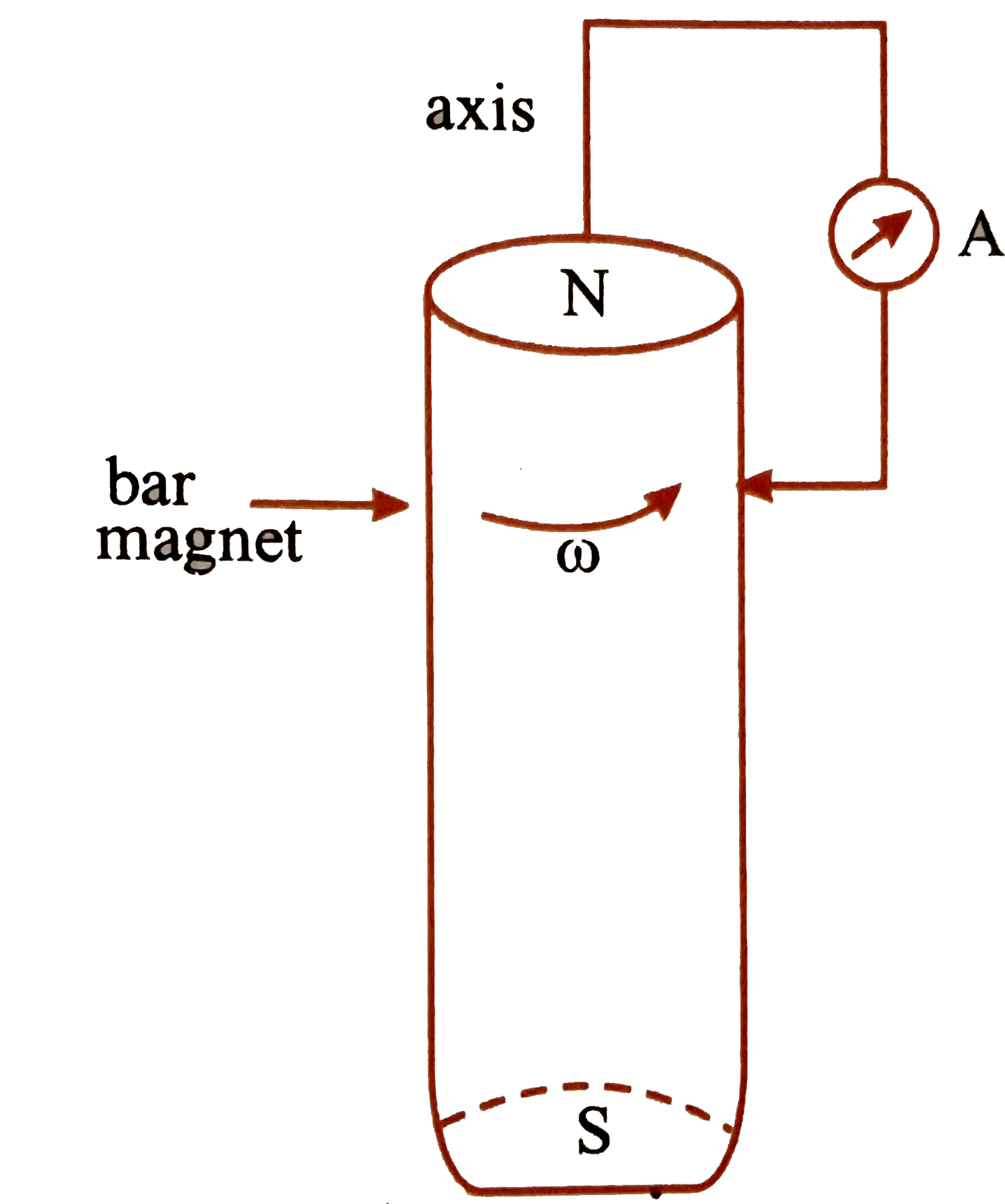 View Solution
View SolutionA cylindrical bar magnet is rotated about its axis (Figure). A wire is connect from the axis and is made to touch the cylindrical surface through a contact. Then
 View Solution
View SolutionConsider metal ring kept on a horizontal plane . A bar magnet is held above the ring with its length along the axis is held above the ring with its length along the axis of the ring . If the magnet is dropped freely the acceleration of the falling magnet is : ( g is accelration due to gravity )
View SolutionFind the magnetic field induction at a point on the axis of a circular coil carrying current and hence find the magnetic field at the centre of circular coil carrying current.
View SolutionA current- carrying straight wire is kept along the axis of a circular loop carrying a current. The straight wire
View SolutionA bar magnet is dropped along the axis of copper ring held horizontally. The acceleration of fall is
View Solution
