Find the energy stored in the capacitor using Kirchhoff's Laws.
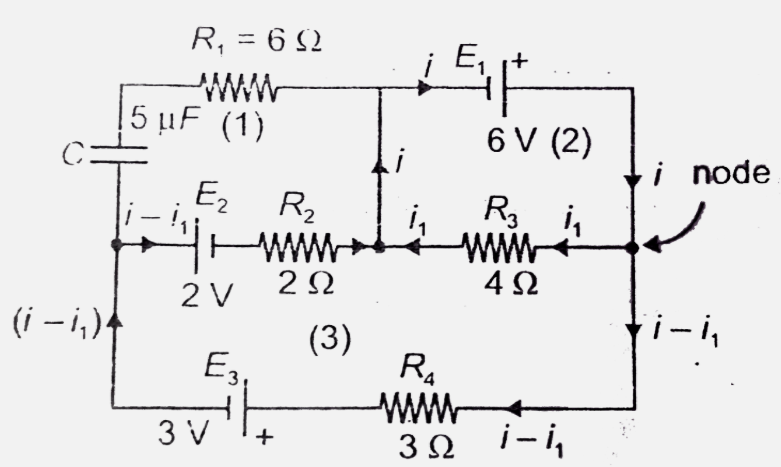
Strategy : Step-1 : Take the current from the batter of highest EMF
Step -2: Divide the total current into branch currents, keeping in mind that no current flows through capacitor.
Step-3 : Apply KVL to 3 loops shown in the figure.
Step -4 : Find the voltage across the capacitor using KVL
Step -5 : Apply the formula E=12CV2 to find energy
(1) Applying KVL to loop 2.
−4i1+6=0⇒i1=1.5A
(2) Applying KVL to loop -3.
=(i−i1)×3−3−(i−i1)×2+i1×4=0
i.e., −5i+9i1=5
∴I=95i1−1
I=1.7A(∵i1=32A)
(3) Applying KVL to loop C , during steady state capacitor acts as a open circuit having voltage across it.
∴2+(1.7−1.5)×+V=0
∴V=−2.4 volts.
(4) Energy stored in the capacitor
E=12CV2=12×5×10−6×(2.4)2
∴E=14.4×10−6J
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Try Yourself34videos
CURRENT ELECTRICITY
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - ASSIGNMENT(SECTION-A(OBJECTIVE TYPE QUESTIONS))69videos
CURRENT ELECTRICITY
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - COMMUNICATION SYSTEMS EXERCISE - ASSIGNMENT SECTION D (Assertion-Reason)10videos
COMMUNICATION SYSTEMS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT (SECTION-D)10videos
DUAL NATURE OF RADIATION AND MATTER
Similar Questions
Find the current in each wire applying only Kirchhoff voltage law
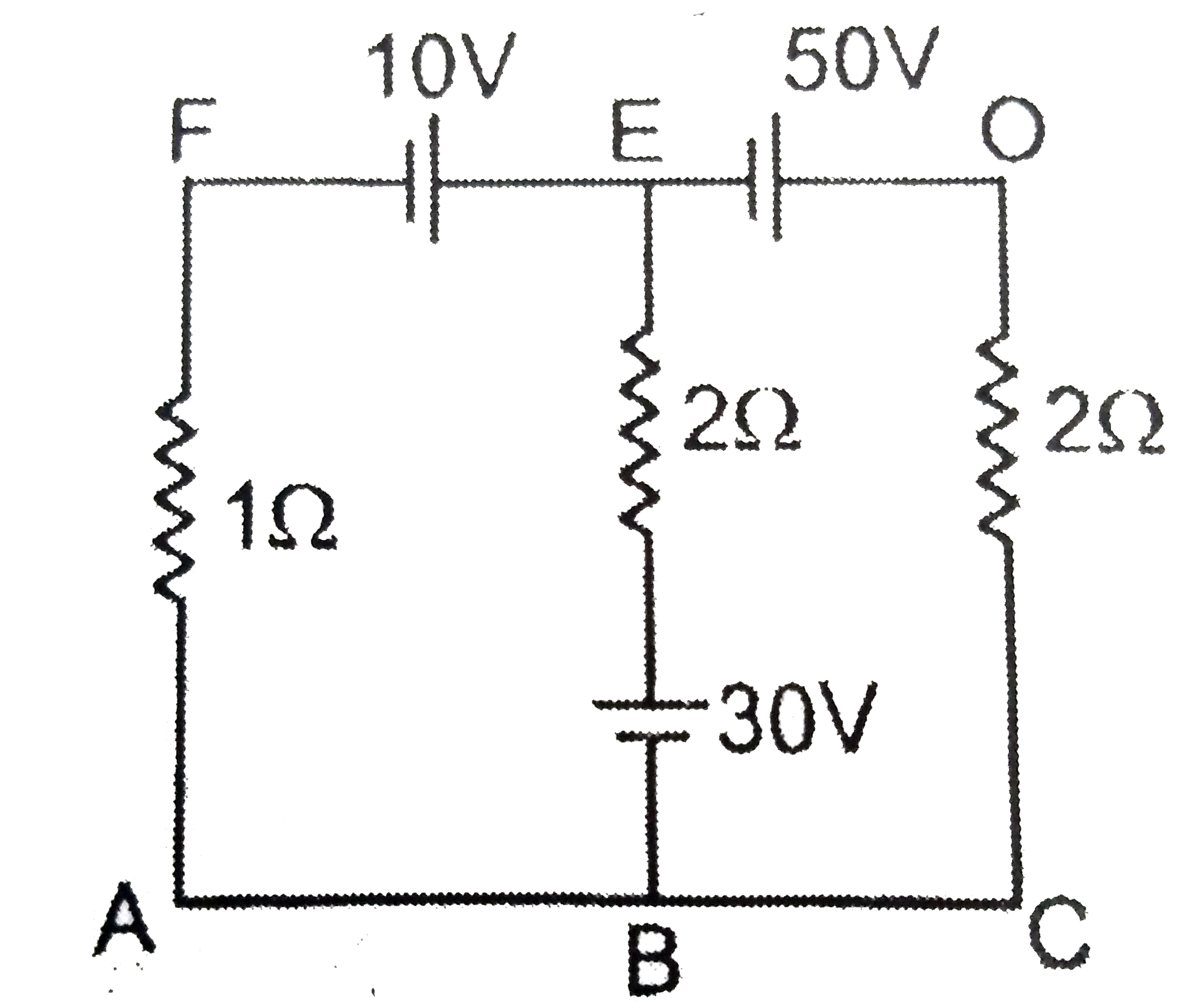 View Solution
View SolutionThe current in 1Ω resistance and charge stored in the capacitor are
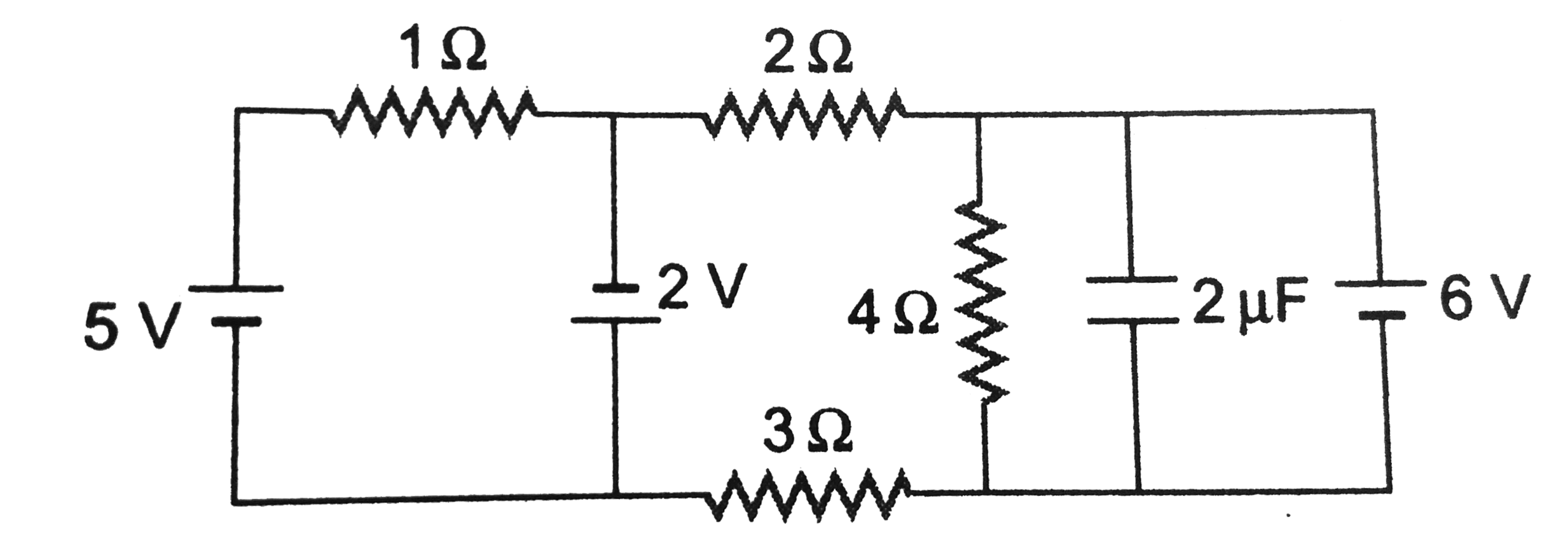 View Solution
View SolutionThe current through the capacitor in the given circuit is
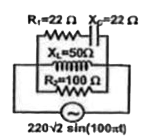 View Solution
View SolutionIn the figure shown the current flowing through 2R is:
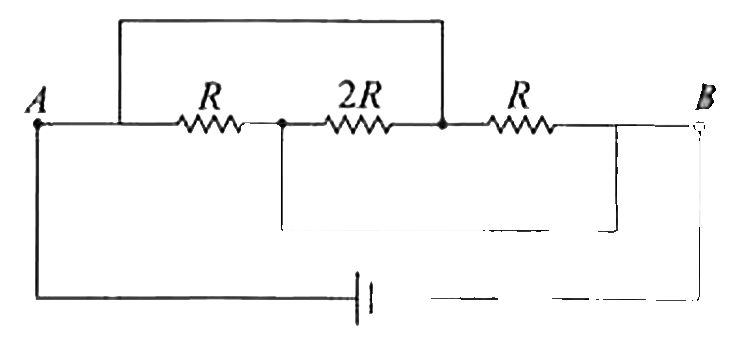 View Solution
View SolutionFind the current in each branch f the given circuit in steady state. Find the charge on each capacitor.
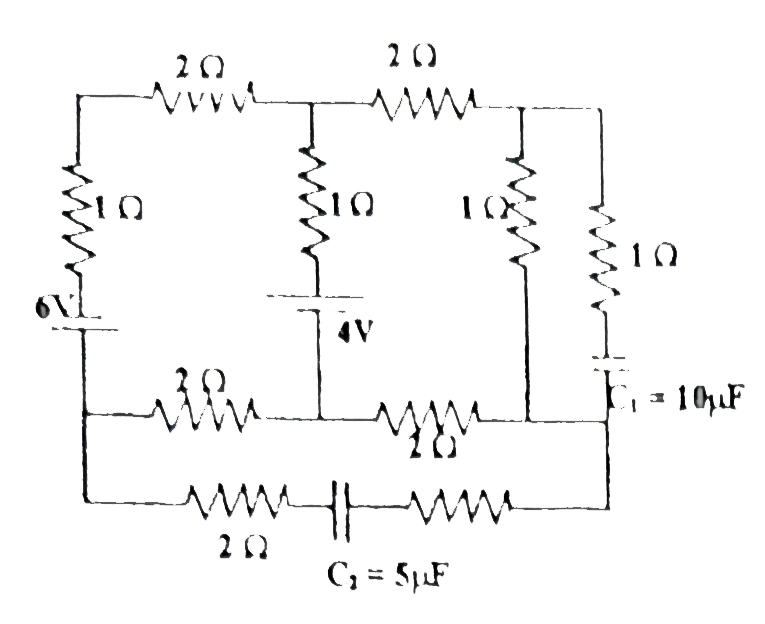 View Solution
View SolutionIn the circuit shown in figure, find the current through the branch BD.
 View Solution
View SolutionThe current flows from A to B as shown in the figure. What is the direction of current in circle?
 View Solution
View SolutionIn given figure, the current (/) is flowing through straight wire annd divided equally in two loops as shown in the figure. The magnitude field intensity at center (O) is
View SolutionIn given figure, current I is flowing through straight wire and divided equally in two loops as shown in the figure. The magnitude of magnetic field intensity at the center (O) is
View SolutionFind the current through 4Ω resistor in the circuit shown in figure.
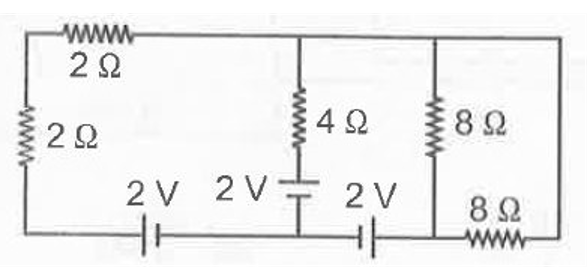 View Solution
View Solution

