The amplitude of electric field at a distance r from a point source of power P is (taking 100% efficiency).
√P2πr2cε0
√P4πr2cε0
√P8πr2cε0
P2πr2cε0
The correct Answer is:A
To find the amplitude of the electric field E0 at a distance r from a point source of power P, we can follow these steps:
1. Understanding Intensity: The intensity I of the electromagnetic wave is defined as the power P per unit area. For a point source radiating uniformly in all directions, the area A at a distance r is given by the surface area of a sphere, which is 4πr2. Thus, the intensity can be expressed as:
I=PA=P4πr2
2. Relating Intensity to Electric Field: The intensity I is also related to the root mean square (RMS) value of the electric field Erms by the formula:
I=E2rmscμ0
where c is the speed of light and μ0 is the permeability of free space.
3. Expressing Erms: We know that the peak amplitude E0 is related to the RMS value by:
Erms=E0√2
Therefore, we can rewrite the intensity in terms of E0:
I=(E0√2)2cμ0=E202cμ0
4. Setting the Two Expressions for Intensity Equal: Now, we can set the two expressions for intensity equal to each other:
P4πr2=E202cμ0
5. Solving for E20: Cross-multiplying gives us:
E20=2cμ0P4πr2
Simplifying this, we have:
E20=cμ0P2πr2
6. Taking the Square Root: Finally, we take the square root to find the amplitude of the electric field:
E0=√cμ0P2πr2
7. Substituting μ0: We know that c=1√μ0ϵ0, thus μ0=1c2ϵ0. Substituting this into our expression for E0:
E0=√P2πr2ϵ0c
Final Result:
Thus, the amplitude of the electric field at a distance r from a point source of power P is given by:
E0=√P2πr2ϵ0c
I=PA=P4πr2
I=E2rmscμ0
where c is the speed of light and μ0 is the permeability of free space.
Erms=E0√2
Therefore, we can rewrite the intensity in terms of E0:
I=(E0√2)2cμ0=E202cμ0
P4πr2=E202cμ0
E20=2cμ0P4πr2
Simplifying this, we have:
E20=cμ0P2πr2
E0=√cμ0P2πr2
E0=√P2πr2ϵ0c
Thus, the amplitude of the electric field at a distance r from a point source of power P is given by:
E0=√P2πr2ϵ0c
Topper's Solved these Questions
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT (SECTION-C) (LINKED COMPREHENSION TYPE QUESTIONS)
3 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT (SECTION-D) (ASSERTION-REASON TYPE QUESTIONS)
8 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - ASSIGNMENT (SECTION-A)(OBJECTIVE TYPE QUESTIONS (ONE OPTIONE IS CORRECT)
50 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION -D) Assertion-Reason type Question)
15 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - ASSIGNMENT SECTION - D
9 Videos
Similar Questions
If the amplitude of waves at a distance r from line source is A. The amplitude at a distance 4r will be
View SolutionThe electric field at a point at a distance r from an electric dipole is proportional to ......
View SolutionA uniform but time-varying magnetic field B(t) exists in a circular region of radius a and is directed into the plane of the paper, as shown. The magnitude of the induced electric field at point P at a distance r from the centre of the circular region
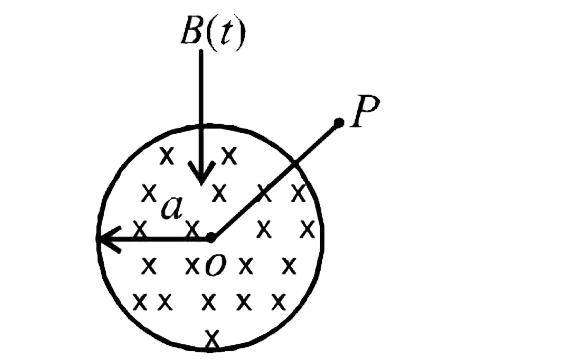
View SolutionA sphere of radius R carries charge such that its volume charge density is proportional to the square of the distance from the centre. What is the ratio of the magnitude of the electric field at a distance 2 R from the centre to the magnitude of the electric field at a distance of R/2 from the centre?
View SolutionA point source of electromagnetic radiation has average power output of 800 watt, then find
(a) Maximum value of electric field at a distance 3.5 m from the source
(b) Maximum value of magnetic field
(c) Average energy density at 3.5 m from the source.
View SolutionA point charge Q is placed at the centre of a spherical conducting shell. A total charge of -q is placed on the shell. The magnitude of the electric field at point P1 at a distance R1 from the centre is X. The magnitude of the electric field due to induced charges at point P2 a distance R2 from the centre is Y. The values of X and Y are respectively.

View SolutionAn isolated solid metal sphere of radius R is given an electric charge. The variation of the intensity of the electric field with the distance r from the centre of the sphere is best shown by
View SolutionEvaluate the amplitude of electric and magnetic field produced by the radiation coming from a 20π W bulb 20% and it is a point source.
View SolutionIf A is the amplitude of the wave coming from a line source at a distance r, then :
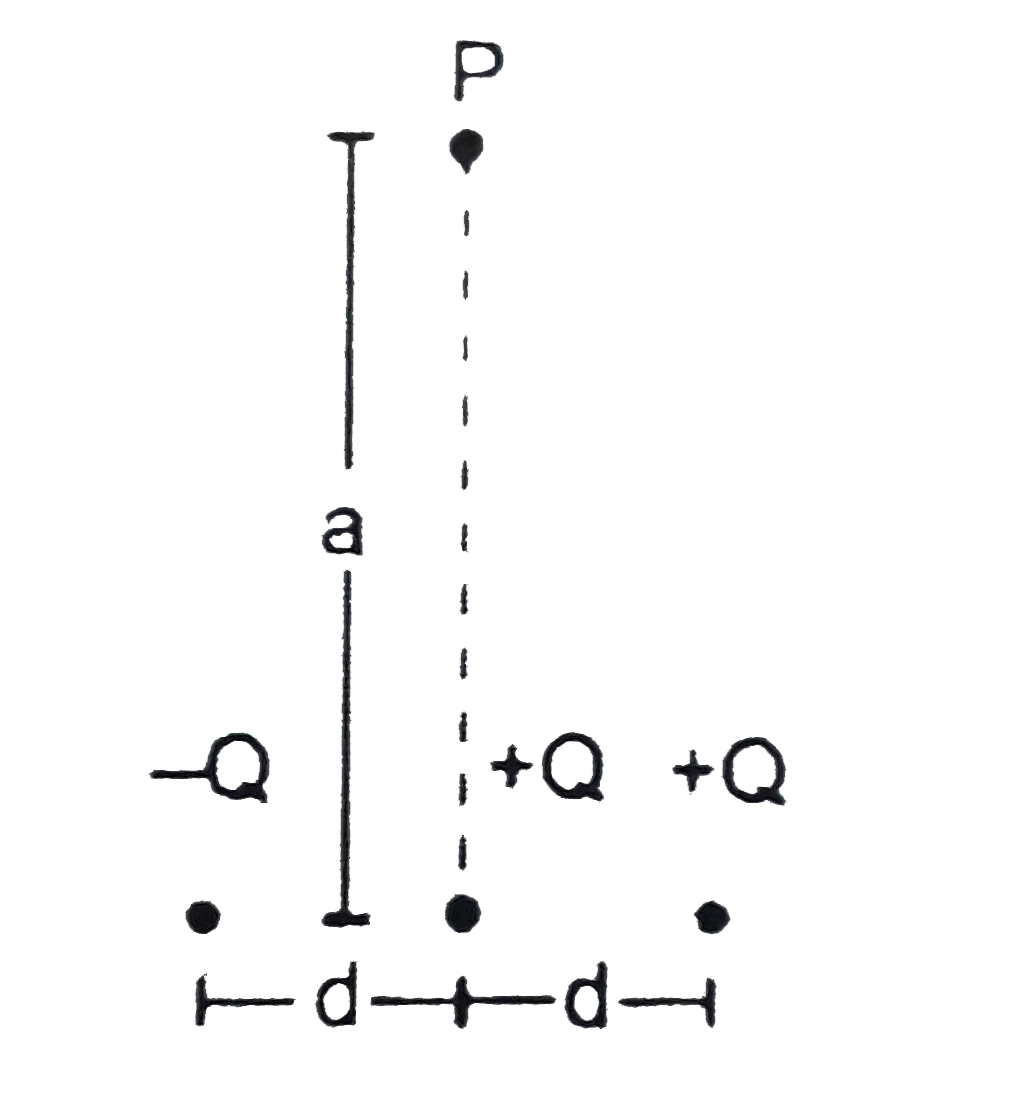
View SolutionThree point charges -Q, Q and Q are placed on a straight line with distance d between charges as shown. Find the magnitude of the electric field at the point P in the configuration shown which is at a distance a from middle charge Q in the system provided that a>>d. Take 2Qd=p.
View Solution
