Ball A is thrown up vertically with speed 10ms. At the same instant another ball B is released from rest at height h. At time t, the speed of A relative to B is
10
10−2gt
√102−2gh
10−gt
The correct Answer is:A
To solve the problem, we need to find the speed of ball A relative to ball B at a given time t.
1. Identify the initial conditions:
- Ball A is thrown upwards with an initial speed uA=10m/s.
- Ball B is released from rest from a height h, so its initial speed uB=0m/s.
2. Calculate the speed of ball A at time t:
- The formula for the speed of an object under uniform acceleration is given by:
v=u+at
- For ball A, the acceleration a is −g (since it is moving upwards against gravity):
vA=uA−gt=10−gtm/s
3. Calculate the speed of ball B at time t:
- For ball B, which is falling under the influence of gravity, the initial speed is 0 and the acceleration is g:
vB=uB+gt=0+gt=gtm/s
4. Determine the relative speed of ball A with respect to ball B:
- Since ball A is moving upwards and ball B is moving downwards, their speeds will be added to find the relative speed:
vAB=vA+vB
- Substituting the expressions we found for vA and vB:
vAB=(10−gt)+(gt)
- Simplifying this gives:
vAB=10m/s
5. Conclusion:
- The relative speed of ball A with respect to ball B at time t is 10m/s.
Final Answer:
The speed of ball A relative to ball B at time t is 10m/s.
---
- Ball A is thrown upwards with an initial speed uA=10m/s.
- Ball B is released from rest from a height h, so its initial speed uB=0m/s.
- The formula for the speed of an object under uniform acceleration is given by:
v=u+at
- For ball A, the acceleration a is −g (since it is moving upwards against gravity):
vA=uA−gt=10−gtm/s
- For ball B, which is falling under the influence of gravity, the initial speed is 0 and the acceleration is g:
vB=uB+gt=0+gt=gtm/s
- Since ball A is moving upwards and ball B is moving downwards, their speeds will be added to find the relative speed:
vAB=vA+vB
- Substituting the expressions we found for vA and vB:
vAB=(10−gt)+(gt)
- Simplifying this gives:
vAB=10m/s
- The relative speed of ball A with respect to ball B at time t is 10m/s.
The speed of ball A relative to ball B at time t is 10m/s.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINEBook:AAKASH INSTITUTE ENGLISHChapter:MOTION IN A STRAIGHT LINEExercise:ASSIGNMENT (SECTION - C)
Explore 36 Videos
- MOTION IN A STRAIGHT LINEBook:AAKASH INSTITUTE ENGLISHChapter:MOTION IN A STRAIGHT LINEExercise:ASSIGNMENT (SECTION - D)
Explore 15 Videos
- MOTION IN A STRAIGHT LINEBook:AAKASH INSTITUTE ENGLISHChapter:MOTION IN A STRAIGHT LINEExercise:ASSIGNMENT (SECTION - A)
Explore 58 Videos
- MOTION IN A PLANEBook:AAKASH INSTITUTE ENGLISHChapter:MOTION IN A PLANEExercise:Assignement section -J (Aakash Challengers Questions)
Explore 7 Videos
- MOTION IN STRAIGHT LINEBook:AAKASH INSTITUTE ENGLISHChapter:MOTION IN STRAIGHT LINEExercise:Assignment (SECTION - J)
Explore 2 Videos
Similar Questions
Knowledge Check
A ball A is thrown up vertically with a speed u and at the same instant another ball B is released from a height h. At time t, the speed A relative to B is
AuBu - 2gtC√u2−2ghDu - gt
Two balls are thrown simultaneously, A vertically upwards with a speed of 20 m/s from the ground, and B vertically downwards from a height of 40 m with the same speed along the same line of motion. At what point do the two balls collide? Take g=9.8m/s2.
View SolutionA ball is thrown up at a speed of 4.0 m/s. Find the maximum height reached by the ball. Take g=10m/s2.
View SolutionA ball of mass 1kg is thrown up with an initial speed of 4m/s. A second ball of mass 2kg is released from rest from some height as shown in Fig. Choose the correct statement (s).
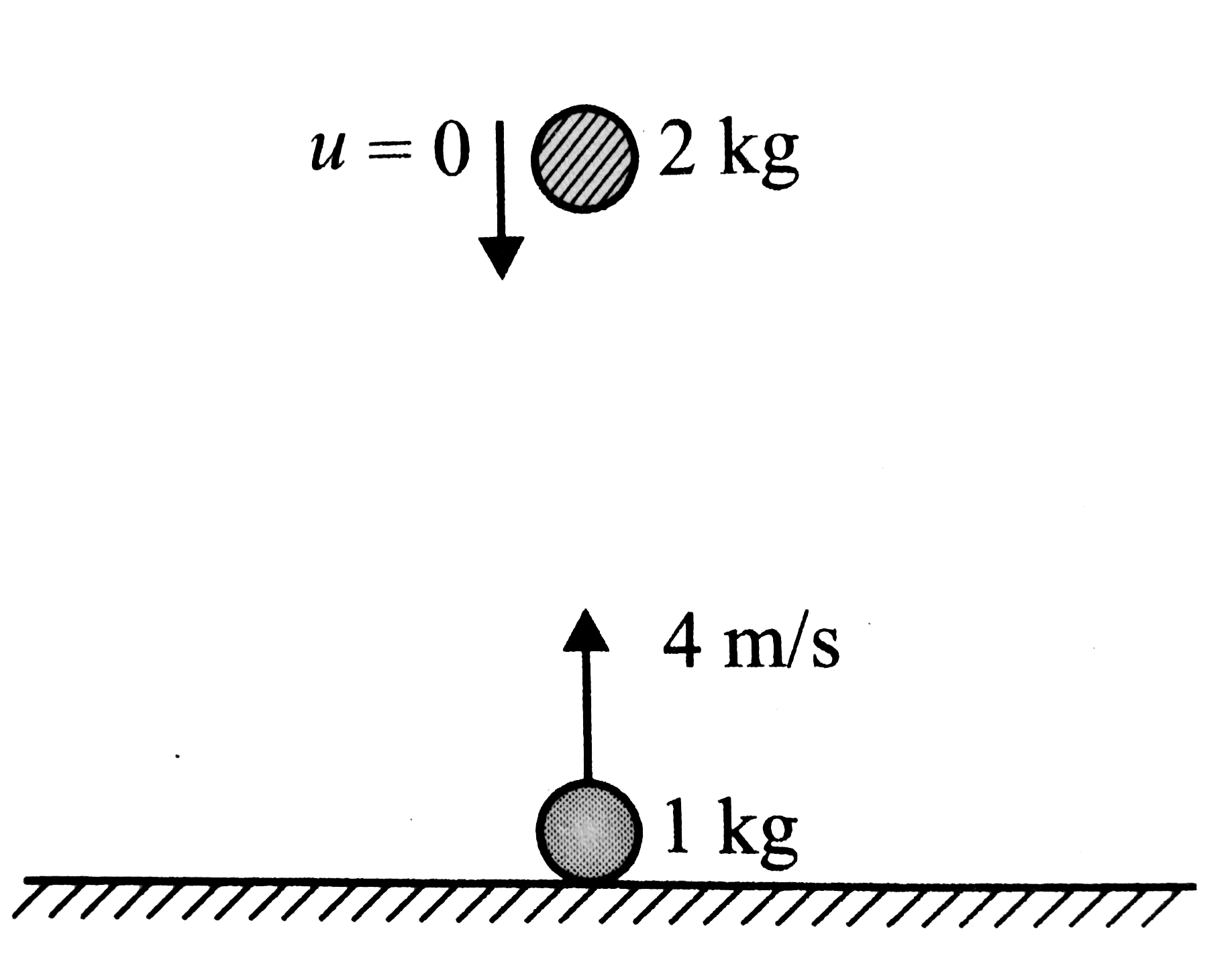
View SolutionA body is dropped from a height h=50 m. Another body is projected vertically up with a speed V=10 m//sec after a time t0= 2 sec from the instant of release of the first body. Find the time of their meetings.
View SolutionA ball weighing 10g hits a hard surface vertically with a speed of 5m/s and rebounds with the same speed The ball remains in contact with the surface speed The ball remains in contact with the surface for 0.01s The average force exerted by the surface on the ball is .
View SolutionA ball is thrown vertically upward with a speed of 20 m/s. Draw a graph showing the velocity of the ball as a function of time as it goes up and then comes back.
View SolutionA ball is projected vertically upward with speed of 50 m/s. Find a. the maximum height, b. the time to reach the maximum height, c. the speed at half the maximum height. Take g=10ms2.
View Solution