A 1000 kg engine pulls a train of 4 wagons each of 2500 kg along a horizontal railway track. If the engine exerts a force of 50000 N on wagons and track offers force of friction 10000 N, then calculate
(i) The net accelerating force
(ii) The acceleration of the train
(iii) The force of wagon-1 on wagon-2
Let's solve the problem step by step.
Given Data:
- Mass of the engine, me=1000kg
- Mass of each wagon, mw=2500kg
- Number of wagons, n=4
- Force exerted by the engine, Fengine=50000N
- Force of friction, Ffriction=10000N
Step 1: Calculate the Net Accelerating Force
The net accelerating force can be calculated using the formula:
Fnet=Fengine−Ffriction
Substituting the values:
Fnet=50000N−10000N=40000N
Step 2: Calculate the Total Mass of the Train
The total mass of the train is the sum of the mass of the engine and the mass of all the wagons:
mtotal=me+n⋅mw
Substituting the values:
mtotal=1000kg+4⋅2500kg=1000kg+10000kg=11000kg
Step 3: Calculate the Acceleration of the Train
Using Newton's second law, the acceleration can be calculated as:
a=Fnetmtotal
Substituting the values:
a=40000N11000kg≈3.636m/s2
Step 4: Calculate the Force of Wagon-1 on Wagon-2
To find the force exerted by wagon-1 on wagon-2, we consider the force required to accelerate the remaining three wagons (wagon-2, wagon-3, and wagon-4):
F1→2=mremaining⋅a
Where mremaining=3⋅mw:
mremaining=3⋅2500kg=7500kg
Now substituting the values:
F1→2=7500kg⋅3.636m/s2≈27270N
Summary of Answers:
1. Net Accelerating Force: Fnet=40000N
2. Acceleration of the Train: a≈3.636m/s2
3. Force of Wagon-1 on Wagon-2: F1→2≈27270N
- Mass of the engine, me=1000kg
- Mass of each wagon, mw=2500kg
- Number of wagons, n=4
- Force exerted by the engine, Fengine=50000N
- Force of friction, Ffriction=10000N
The net accelerating force can be calculated using the formula:
Fnet=Fengine−Ffriction
Fnet=50000N−10000N=40000N
The total mass of the train is the sum of the mass of the engine and the mass of all the wagons:
mtotal=me+n⋅mw
Substituting the values:
mtotal=1000kg+4⋅2500kg=1000kg+10000kg=11000kg
Using Newton's second law, the acceleration can be calculated as:
a=Fnetmtotal
Substituting the values:
a=40000N11000kg≈3.636m/s2
To find the force exerted by wagon-1 on wagon-2, we consider the force required to accelerate the remaining three wagons (wagon-2, wagon-3, and wagon-4):
F1→2=mremaining⋅a
Where mremaining=3⋅mw:
mremaining=3⋅2500kg=7500kg
Now substituting the values:
F1→2=7500kg⋅3.636m/s2≈27270N
1. Net Accelerating Force: Fnet=40000N
2. Acceleration of the Train: a≈3.636m/s2
3. Force of Wagon-1 on Wagon-2: F1→2≈27270N
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE
69 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-A)
65 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - ASSIGNMENT ( SECTION -J ) Aakash Challengers Questions
12 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
Similar Questions
A 8000kg engine pulls a train of 5wagons, each of 2000kg, along a horizontal track. If the engine exerts a force of 40000N and the track offers a frictional force of 5000N, then calculate:
(a) the net accelerating force, (b) the acceleration of the train, and
(c ) the force of wagon 1 on wagon 2.
View SolutionA force of 15 N acts on a body of mass 2 kg. Calculate the acceleration produced.
View Solution
Knowledge Check
A stone of mass 1 kg is lying on the floor of a train which is accelerating with 1ms−2. The net force acting on the stone is
AzeroB1NC5ND10N
A force of 15 N acts on a body of mass 2 kg. Calculate the acceleration produced.
View SolutionThe acceleration of the 2 kg block if the free end of string is pulled with a force of 20 N as shown is
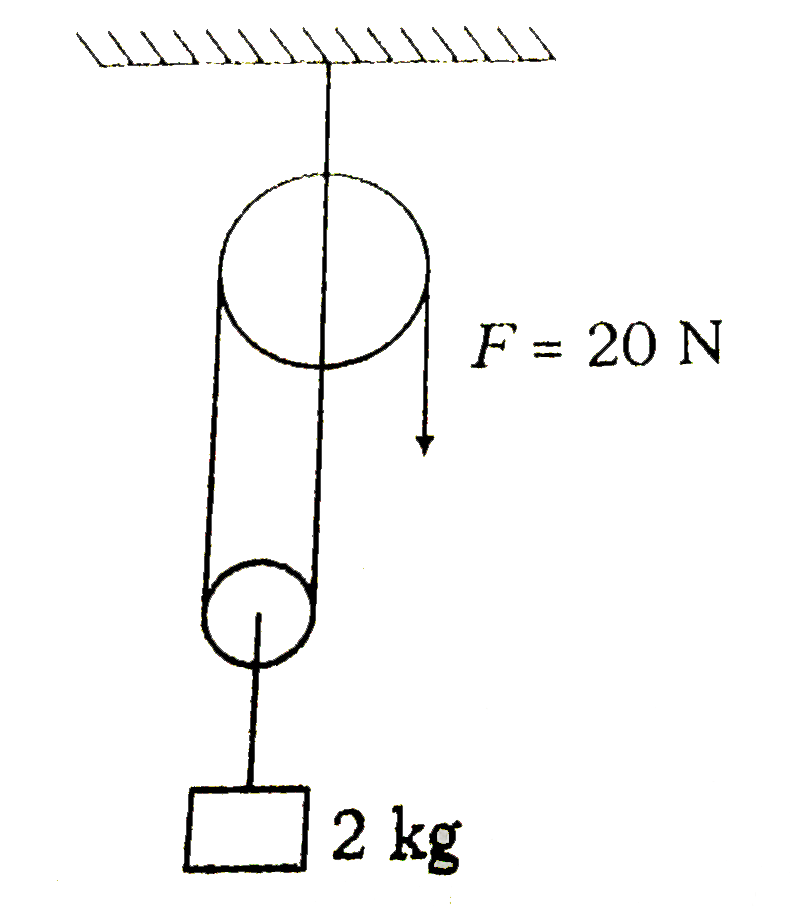
View SolutionA block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
View SolutionA block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
View SolutionA block of mass 2 kg rests on a horizontal surface. If a horizontal force of 5 N is applied on the block, the frictional force on it is (take,uk=0.4,μs=0.5)
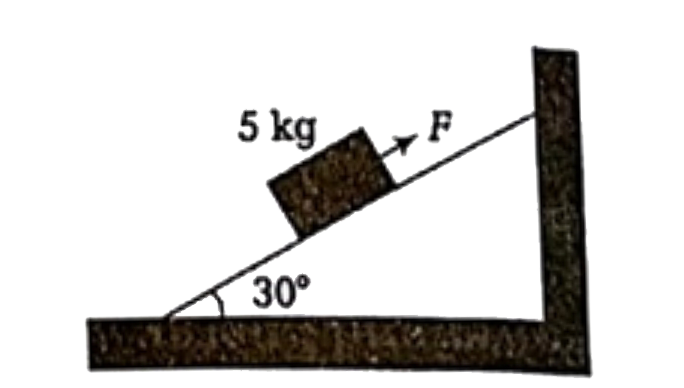
View SolutionA force F=75 N is applied on a block of mass 5 kg along the fixed smooth incline as as shown in the figure. Here gravitational acceleration g = 10 m s−2 . The acceleration of the block is
View SolutionA roller of mass 500kg is attached by a light horizontal chain to a tractor of mass 500kg. Assume the force of friction exerted by the ground to be 1000N. If the system has a forward acceleration of 2ms−2, calculate (i) the total force required for the motion and (ii) the tension in the chain.
View Solution
