If angle of repose is , the coefficient of static friction between body and the plane is
To find the coefficient of static friction (μ) between a body and an inclined plane when the angle of repose is ϕ, we can follow these steps:
1. Understanding the Angle of Repose:
- The angle of repose (ϕ) is the maximum angle at which a body can remain at rest on an inclined plane without sliding down. At this angle, the forces acting on the body are balanced.
2. Identify the Forces:
- When the body is on the inclined plane, the weight of the body (mg) can be resolved into two components:
- Perpendicular to the incline: mgcosϕ (this is the normal force, N)
- Parallel to the incline: mgsinϕ (this is the force trying to pull the body down the incline)
3. Frictional Force:
- The frictional force (f) that opposes the motion of the body is given by:
f=μN
- Substituting for N:
f=μ(mgcosϕ)
4. Setting Up the Equilibrium Condition:
- At the angle of repose, the frictional force balances the component of the weight acting down the incline:
f=mgsinϕ
5. Equating the Forces:
- Setting the expressions for friction equal gives:
μ(mgcosϕ)=mgsinϕ
6. Canceling mg:
- Since mg is present on both sides of the equation, we can cancel it out (assuming mg≠0):
μcosϕ=sinϕ
7. Solving for the Coefficient of Static Friction:
- Rearranging the equation gives:
μ=sinϕcosϕ
- This simplifies to:
μ=tanϕ
Final Answer:
The coefficient of static friction between the body and the plane is:
μ=tanϕ
- The angle of repose (ϕ) is the maximum angle at which a body can remain at rest on an inclined plane without sliding down. At this angle, the forces acting on the body are balanced.
- When the body is on the inclined plane, the weight of the body (mg) can be resolved into two components:
- Perpendicular to the incline: mgcosϕ (this is the normal force, N)
- Parallel to the incline: mgsinϕ (this is the force trying to pull the body down the incline)
- The frictional force (f) that opposes the motion of the body is given by:
f=μN
- Substituting for N:
f=μ(mgcosϕ)
- At the angle of repose, the frictional force balances the component of the weight acting down the incline:
f=mgsinϕ
- Setting the expressions for friction equal gives:
μ(mgcosϕ)=mgsinϕ
- Since mg is present on both sides of the equation, we can cancel it out (assuming mg≠0):
μcosϕ=sinϕ
- Rearranging the equation gives:
μ=sinϕcosϕ
- This simplifies to:
μ=tanϕ
The coefficient of static friction between the body and the plane is:
μ=tanϕ
Topper's Solved these Questions
- LAWS OF MOTIONBook:AAKASH INSTITUTE ENGLISHChapter:LAWS OF MOTIONExercise:Assignment (SECTION-A)
Explore 65 Videos
- LAWS OF MOTIONBook:AAKASH INSTITUTE ENGLISHChapter:LAWS OF MOTIONExercise:Assignment (SECTION-B)
Explore 44 Videos
- LAWS OF MOTIONBook:AAKASH INSTITUTE ENGLISHChapter:LAWS OF MOTIONExercise:Example
Explore 24 Videos
- KINETIC THEORYBook:AAKASH INSTITUTE ENGLISHChapter:KINETIC THEORYExercise:EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
Explore 10 Videos
- MAGNETISM AND MATTER Book:AAKASH INSTITUTE ENGLISHChapter:MAGNETISM AND MATTER Exercise:ASSIGNMENT (SECTION D)
Explore 26 Videos
Similar Questions
Knowledge Check
A block of mass 3 kg rests on a rough inclined plane making an angle of 30∘. With the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
A9.8 NB0.7×9.8×√3 NC9.8×√3 ND0.7×9.8 N
The coefficient of static friction between two surfaces depend on
View SolutionA block kept on a rough inclined plane, as shown in the figure, remains at rest upto a maximum force 2N down the inclined plane. The maximum external force up the inclined plane that does not move the block is 10N. The coefficient of static friction between the block and the plane is : : [Taking g=10m/s2].

View SolutionA body is moving down along inclined plane of angle of inclination q. The coefficient of friction between the body and the plane varies as mu = 0.5x, where x is the distance moved down the plane. The body will have the maximum velocity when it has travelled a distance x given by
View SolutionA block of mass 4kg rests on a rough horizontal plane. The plane is gradually inclined until at an angle θ=60∘ , with the vertical, the mass just begins to side. What is the coefficient of static friction between the block and the plane?
View SolutionStatic friction between two surfaces
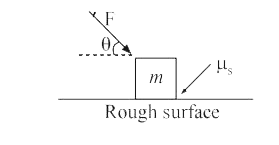
View SolutionWhat is the value of applied force ‘F’ which is exerted at an angle θ with the horizontal on a block of mass ‘m’ placed on a horizontal rough surface, if the coefficient of static friction between the block and the surface is , such that the block is on the verge of moving horizontally?
View SolutionA block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
View Solution