A block of mass m kg is kept on a weighing machine in an elevator. If the elevator is retarding upward by a ms−2, the reading of weighing machine is
mg
m(g−a)
m(1−1g)
m(g+a)
To solve the problem, we need to analyze the forces acting on the block of mass m in the elevator that is accelerating upwards with an acceleration of a m/s².
1. Identify the Forces:
- The weight of the block acting downwards: Fg=mg (where g is the acceleration due to gravity).
- The pseudo force acting upwards due to the upward acceleration of the elevator: Fp=ma.
2. Determine the Net Force:
- In the frame of reference of the elevator, the effective force acting on the block is the sum of the gravitational force and the pseudo force. Therefore, the total effective force F acting on the block is:
F=mg+ma
3. Calculate the Reading of the Weighing Machine:
- The weighing machine measures the normal force N exerted by the block on it. In this case, since the elevator is accelerating upwards, the reading of the weighing machine will be equal to the effective force acting on the block:
N=mg+ma
4. Final Expression for the Reading:
- Thus, the reading of the weighing machine can be expressed as:
N=m(g+a)
Conclusion:
The reading of the weighing machine when the elevator is retarding upwards by a m/s² is:
Reading=m(g+a)
- The weight of the block acting downwards: Fg=mg (where g is the acceleration due to gravity).
- The pseudo force acting upwards due to the upward acceleration of the elevator: Fp=ma.
- In the frame of reference of the elevator, the effective force acting on the block is the sum of the gravitational force and the pseudo force. Therefore, the total effective force F acting on the block is:
F=mg+ma
- The weighing machine measures the normal force N exerted by the block on it. In this case, since the elevator is accelerating upwards, the reading of the weighing machine will be equal to the effective force acting on the block:
N=mg+ma
- Thus, the reading of the weighing machine can be expressed as:
N=m(g+a)
The reading of the weighing machine when the elevator is retarding upwards by a m/s² is:
Reading=m(g+a)
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-A)
65 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-B)
44 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Example
24 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
Similar Questions
A body of mass 40 kg stands on a weighing machine in an accelerated lift. The reading on the scale of the weighing machine is 300 N. Find the magnitude and direction of acceleration. (g=9.8ms−2)
View SolutionA man of mass 40kg is standing on a weighting machine kept on the floor of an elevator which is moving up with an acceleration of 2m/s2 Find the reading of the weighing maching.

View Solution
Knowledge Check
The person o( mass 50 kg slands on a weighing scale on a lift. If the lift is ascending upwards with a uniform acceleration of 9ms−2, what would be the reading of the weighting scale? (Take g=10ms−2)
A50kgB60kgC96kgD176kg
A man of mass 40kg is standing on a weighting machine kept on the floor of an elevator which is moving up with an acceleration of 2m/s2 Find the reading of the weighing maching.

View SolutionA man of mass M stands on a weighing machine in an elevator accelerating upwards with an acceleration a. Draw the free-body diagram of the man as observed by the observer A (stationary on the ground) and observer B (stationary on the elevator). Also, calculate the reading of the weighing machine.

View SolutionA 80 kg man is standing on a weightless weighing machine as shown in the figure if mass of hanging block is 40 kg, then reading of weighing machine, is
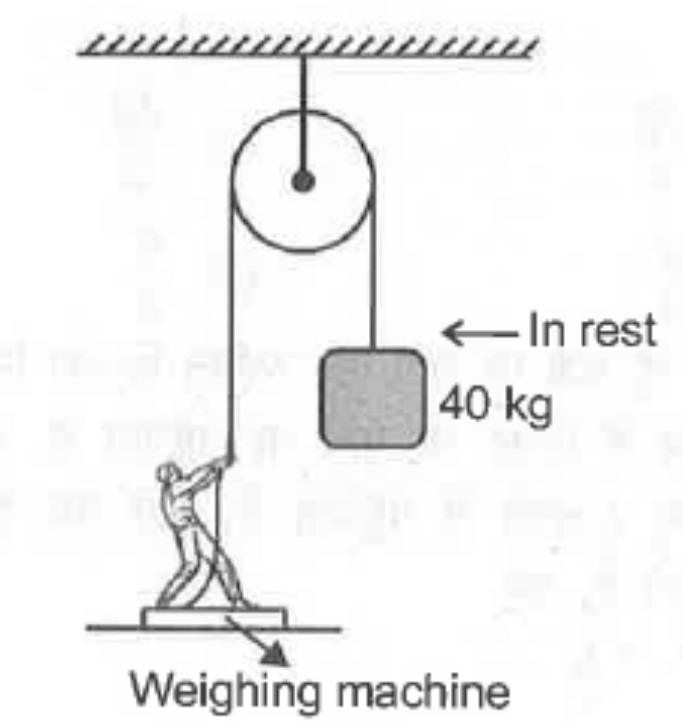
View SolutionA person of mass 50 kg stands on a weighing scale on a lift . If the lift is descending with a downward acceleration of 9ms−2 what would be the reading of the weighing scale? (g=10ms−2) .
View SolutionA person of mass 50 kg stands on a weighing scale on a lift . If the lift is descending with a downward acceleration of 9ms−2 what would be the reading of the weighing scale? (g=10ms−2) .
View SolutionA block of mass m is suspended by a light thread from an elevator. The elevator is accelerating upward with uniform acceleration a. The work done by tension on the block during t seconds is (u = 0)
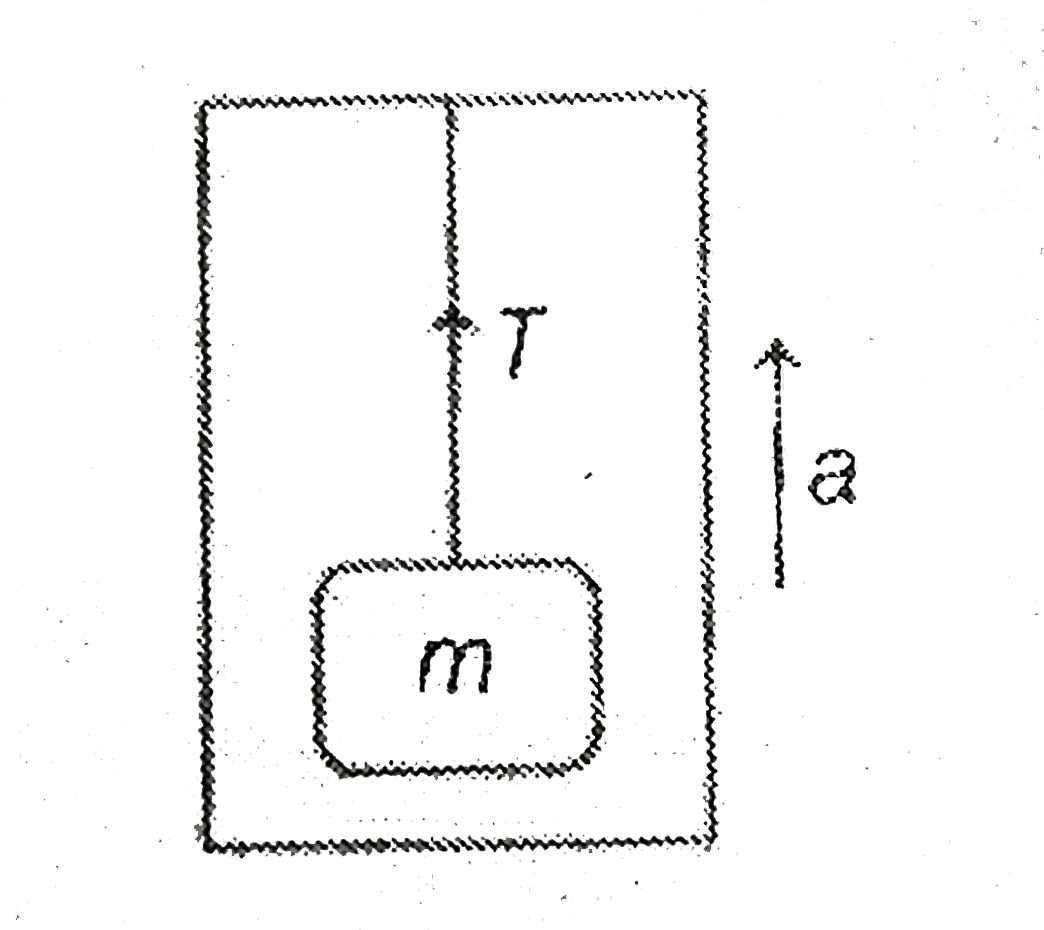
View SolutionA small block of mass 1kg is kept on a rough inclined wedge of inclination 45∘ fixed in an elevator. The elevator goes up with a uniform velocity v=2m/s and the block does not slide on the wedge. Find the work done by the force of friction on the block in 1s. (g=10m/s2)
View Solution
