During rolling without slipping, what is the velocity of the point in contact with the surface.
To solve the problem of finding the velocity of the point in contact with the surface during rolling without slipping, we can follow these steps:
1. Understand the Concept of Rolling Without Slipping:
- When an object rolls without slipping, the point of contact between the object and the surface does not slide. This means that the velocity of this point is zero relative to the surface.
2. Define the Variables:
- Let R be the radius of the rolling object (for example, a ring or a sphere).
- Let V be the linear velocity of the center of mass of the rolling object.
- Let ω be the angular velocity of the rolling object.
3. Identify the Velocity Components:
- The linear velocity V acts at the center of mass of the object.
- The point of contact has a velocity due to the rotation of the object, which is given by Rω but acts in the opposite direction to the linear velocity V.
4. Set Up the Equation:
- Since the point of contact is at rest relative to the surface, we can express this condition mathematically:
V−Rω=0
5. Solve for the Velocity of the Point of Contact:
- Rearranging the equation gives:
V=Rω
- This means the linear velocity of the center of mass is equal to the radius times the angular velocity.
6. Conclusion:
- Therefore, the velocity of the point in contact with the surface during rolling without slipping is zero.
Final Answer:
The velocity of the point in contact with the surface during rolling without slipping is 0.
- When an object rolls without slipping, the point of contact between the object and the surface does not slide. This means that the velocity of this point is zero relative to the surface.
- Let R be the radius of the rolling object (for example, a ring or a sphere).
- Let V be the linear velocity of the center of mass of the rolling object.
- Let ω be the angular velocity of the rolling object.
- The linear velocity V acts at the center of mass of the object.
- The point of contact has a velocity due to the rotation of the object, which is given by Rω but acts in the opposite direction to the linear velocity V.
- Since the point of contact is at rest relative to the surface, we can express this condition mathematically:
V−Rω=0
- Rearranging the equation gives:
V=Rω
- This means the linear velocity of the center of mass is equal to the radius times the angular velocity.
- Therefore, the velocity of the point in contact with the surface during rolling without slipping is zero.
The velocity of the point in contact with the surface during rolling without slipping is 0.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - J) Aakash Challengers Questions
13 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))
5 Videos
- TEST 1BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE
21 Videos
Similar Questions
In the figure shown, a ring A is initially rolling without sliding with a velocity 1, on the horizontal surface of the body B (of same mass as A). All surfaces arc smooth. B has no initial velocity. What will be the maximum height reached by A on B?

View SolutionA cylinder executes pure rolling without slipping with a constant velocity on a plank, whose upper surface is rough enough, but lower surface is smooth. The plank is kept at rest on a smooth horizontal surface by the application of an external force F. The value of F is ?
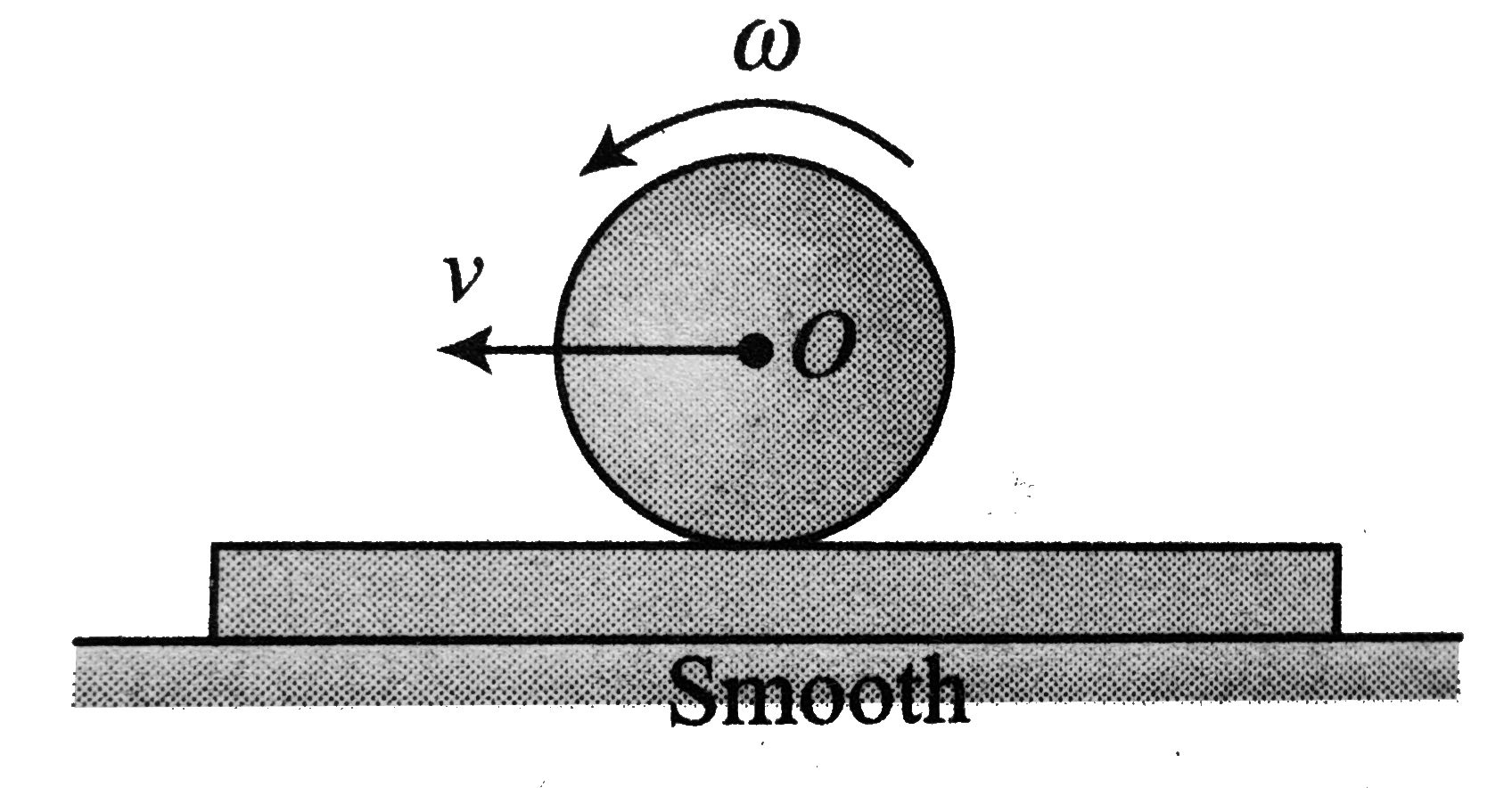
View Solution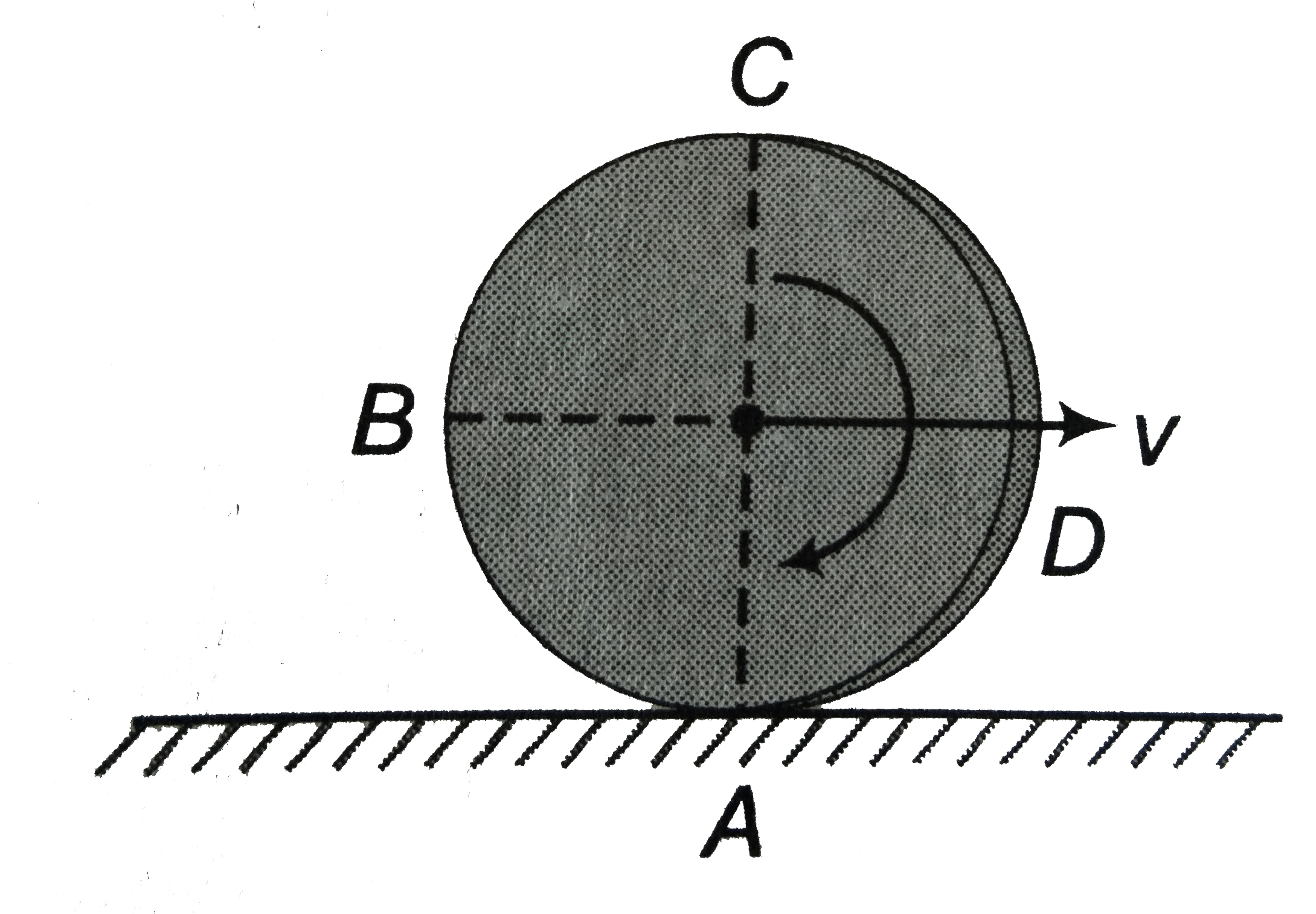
A disc is rolling without slipping with linear velocity v as shown in figure. With the concept of instantaneous axis of rotation, find velocities of point A, B, C and D.
View SolutionA bullet of mass m moving with a velocity of u just grazes the top of a solid cylinder of mass M and radius R resting on a rough horizontal surface as shown and is embedded in the cylinder after impact. Assuming that the cylinder rolls without slipping, find the angular velocity of the cylinder and the final velocity of the bullet.

View SolutionAs shown in the figure, a disc of mass m is rolling without slipping with angular velocity ω. When it crosses point B disc will be in
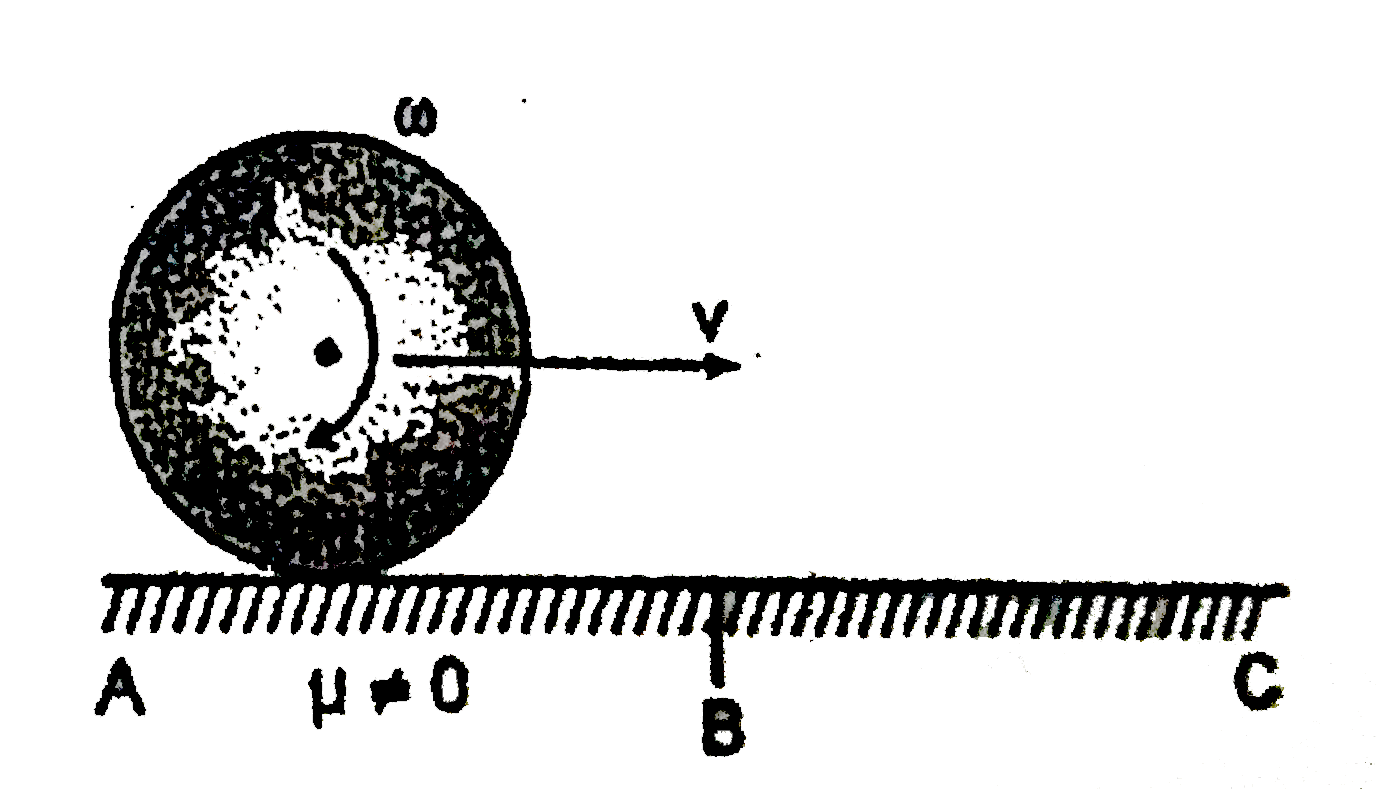
View SolutionA wheel of bicycle is rolling without slipping on a level road. The velocity of the centre of mass is vCM, then true statement is

View SolutionA wheel of bicycle is rolling without slipping on a level road. The velocity of the centre of mass is vCM, then true statement is

View SolutionA solid cylinder is rolling without slipping with velocity of its centre of mass v and angular velocity about its centre of mass ω on a horizontal frictionless surface as shown in figure. If it collides with a frictionless vertical wall X, then after collision its velocity and angular velocity respectively become
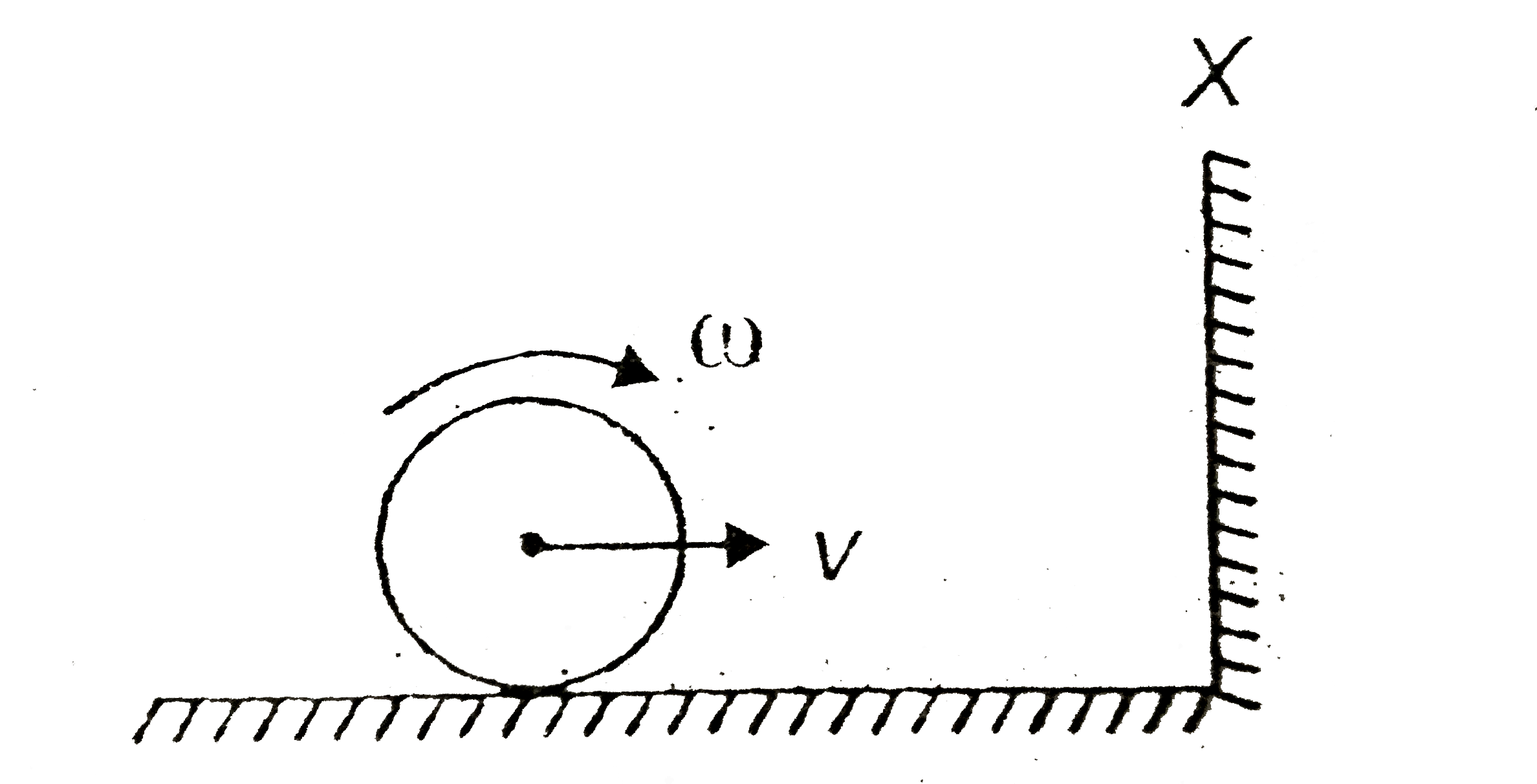
View SolutionA disc of radius R rolls without slipping at speed v along positive x-axis. Velocity of point P at the instant shown in Fig. is
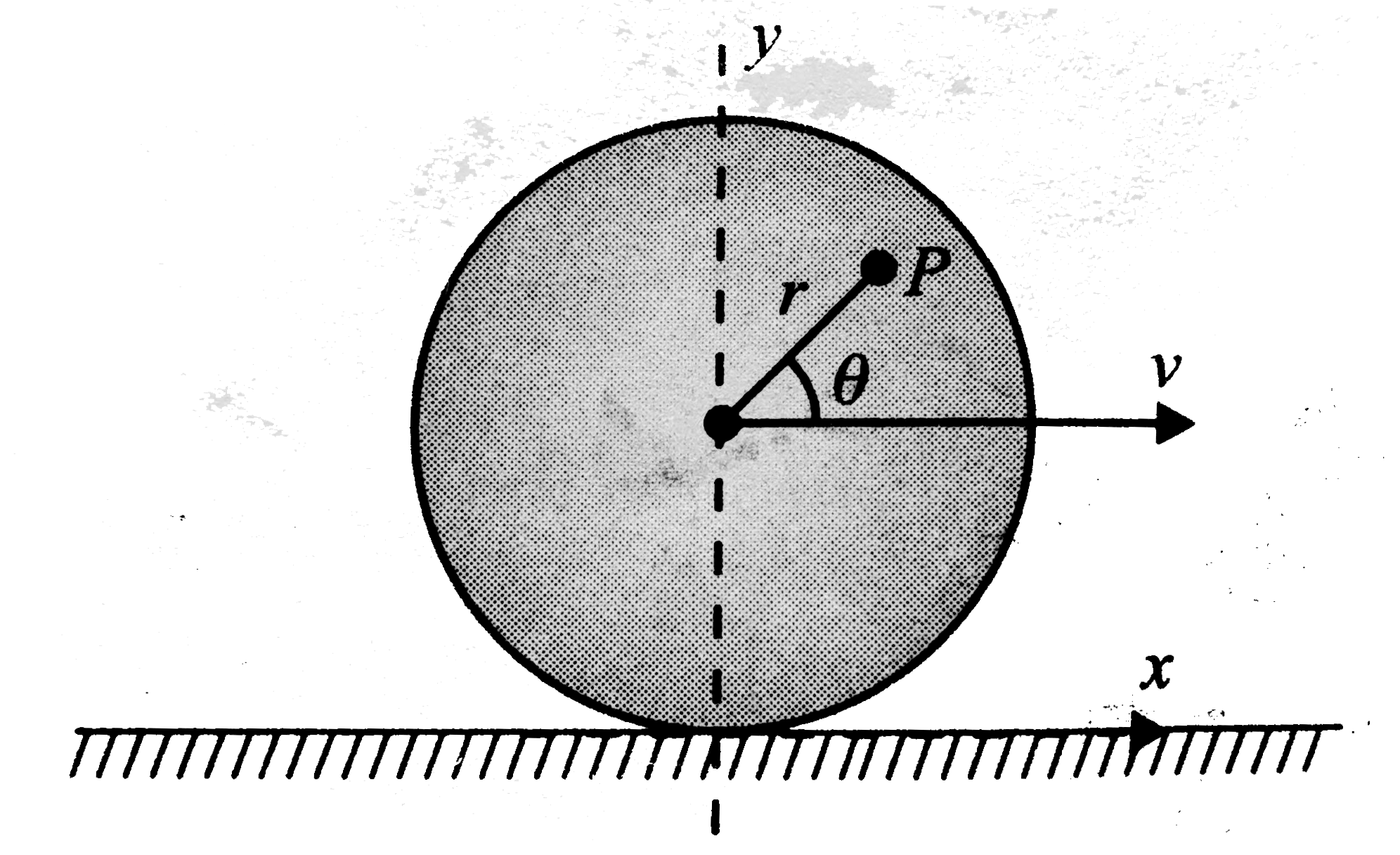
View SolutionA disc is rolling without sliding on a horizontal surface. Velocity of the centre of the disc is V. Then the maximum relative sped of any point on the circumference of the disc with respect to point P is.
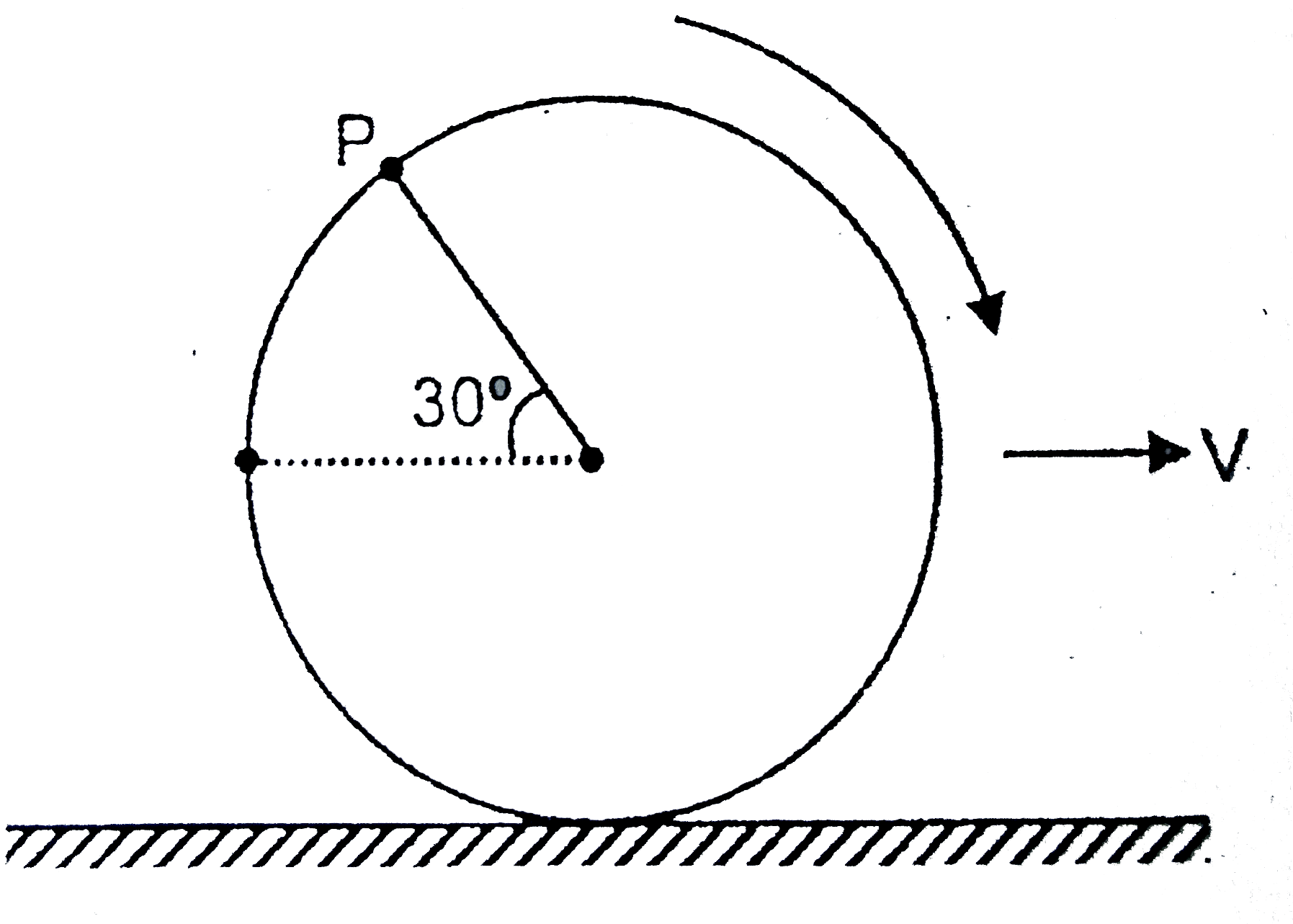
View Solution
