If gravitational field Intensity is E at distance R/2 outside from then surface of a thin shell of radius R , the gravitational field intensity at distance R/2 from its centre is
Zero
2E
2E3
3E2
The correct Answer is:A
To solve the problem, we need to determine the gravitational field intensity at a distance R2 from the center of a thin spherical shell of radius R, given that the gravitational field intensity is E at a distance R2 outside the surface of the shell.
1. Understand the Setup:
- We have a thin spherical shell of radius R.
- The gravitational field intensity E is given at a distance R2 outside the shell. This means the distance from the center of the shell to this point is R+R2=3R2.
2. Apply Gauss's Law for Gravitation:
- According to Gauss's law for gravitation, the gravitational field intensity g at a distance r from the center of a spherical shell is given by:
g=−GMr2
- However, this applies only outside the shell. Inside the shell, the gravitational field intensity behaves differently.
3. Evaluate the Gravitational Field Inside the Shell:
- For a hollow thin spherical shell, the gravitational field inside the shell (at any point within the shell) is zero. This is a result of the symmetry of the shell and can be derived from Gauss's law:
- If we consider a Gaussian surface inside the shell, the mass enclosed by this surface is zero since all the mass is on the shell's surface.
- Thus, by Gauss's law:
∮→E⋅d→A=−4πGMenclosed=0
- This implies that the gravitational field intensity E inside the shell is zero.
4. Conclusion:
- Therefore, at a distance R2 from the center of the shell, the gravitational field intensity is:
Einside=0
Final Answer:
The gravitational field intensity at a distance R2 from the center of the shell is 0.
- We have a thin spherical shell of radius R.
- The gravitational field intensity E is given at a distance R2 outside the shell. This means the distance from the center of the shell to this point is R+R2=3R2.
- According to Gauss's law for gravitation, the gravitational field intensity g at a distance r from the center of a spherical shell is given by:
g=−GMr2
- However, this applies only outside the shell. Inside the shell, the gravitational field intensity behaves differently.
- For a hollow thin spherical shell, the gravitational field inside the shell (at any point within the shell) is zero. This is a result of the symmetry of the shell and can be derived from Gauss's law:
- If we consider a Gaussian surface inside the shell, the mass enclosed by this surface is zero since all the mass is on the shell's surface.
- Thus, by Gauss's law:
∮→E⋅d→A=−4πGMenclosed=0
- This implies that the gravitational field intensity E inside the shell is zero.
- Therefore, at a distance R2 from the center of the shell, the gravitational field intensity is:
Einside=0
The gravitational field intensity at a distance R2 from the center of the shell is 0.
Topper's Solved these Questions
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - C (PREVIOUS YEARS QUESTIONS)
51 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
15 Videos
- GRAVITATION BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - GRAVITATION EXERCISE - ASSIGNMENT SECTION - A (OBJECTIVE TYPE QUESTIONS)
41 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - ASSIGNMENT SECTION - D
9 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
Similar Questions
The electric field at a distance 3R/2 from the centre of a charge conducting spherical shell of radius R is E. The electric field at a distance R/2 from the centre of the sphere is
View SolutionThe electric field at a distance 3R/2 from the centre of a charge conducting spherical shell of radius R is E. The electric field at a distance R/2 from the centre of the sphere is
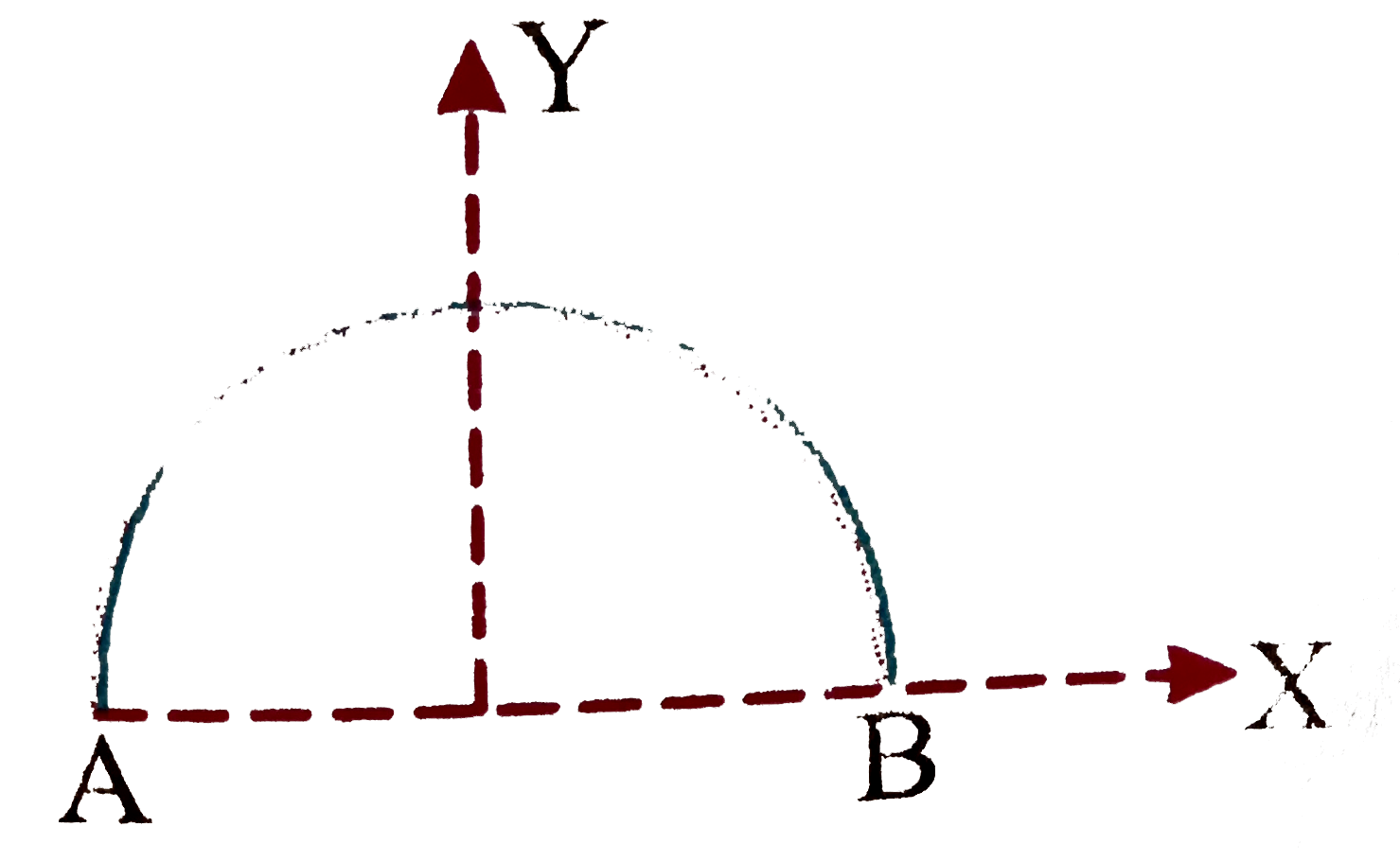
View SolutionGravitational field intensity at the centre of the semi circle formed by a thin wire AB of mass m and length L is
View SolutionIn a spherical region the densiy varies inversely with the distance from ther centre Gravitational field at a distance r from the centre is .
View SolutionThe electric field intensity at a distance 20cm from the centre of a uniformly charged non conducting solid sphere of radius 10cm is E.Then what is the electric field intensity at a distance 5cm from the centre it will be.....
View SolutionA positive point charge q is placed at a distance 2 R from the surface of a metallic shell of radius R. the electric field at centre of shell due to indouced charge has magnitude
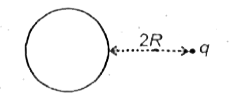
View SolutionThe distance between the centres of the Moon and the earth is D. The mass of the earth is 81 times the mass of the Moon. At what distance from the centre of the earth, the gravitational force will be zero?
View SolutionA uniform solid sphere of mass M and radius a is surrounded symmetrically by a uniform thin spherical shell of equal mass and radius 2a. Find the gravitational field at a distance a). 3/2 a from the centre , b). 5/2 as from the centre.
View SolutionCalculate the gravitational field intensity and potential at the centre of the base of a solid hemisphere of mass m, radius R
View SolutionA solid sphere of radius a and mass m is surrounded by cocentric spherical shell of thickness 2a and mass 2m the gravitational field at a distance 3a from their centres is
View Solution