In an LCR circuit, the resonating frequency is 500 kHz. If the value of L is increased two times and value of C is decreased times, then the new resonating frequency in kHz will be -
250
500
1000
2000
The correct Answer is:C
To find the new resonating frequency of the LCR circuit after changing the values of inductance (L) and capacitance (C), we can follow these steps:
1. Understand the formula for resonating frequency:
The resonating frequency f of an LCR circuit is given by the formula:
f=12π√LC
2. Identify the initial conditions:
We are given that the initial resonating frequency f1 is 500 kHz. We can express this in Hz for calculations:
f1=500×103 Hz
3. Express the initial values of L and C:
Let the initial values of inductance and capacitance be L1 and C1 respectively. Therefore, we can write:
f1=12π√L1C1
4. Change the values of L and C:
According to the problem:
- The new inductance L2=2L1 (L is doubled)
- The new capacitance C2=18C1 (C is decreased to one-eighth)
5. Substitute the new values into the frequency formula:
The new resonating frequency f2 can be expressed as:
f2=12π√L2C2=12π√(2L1)(18C1)
6. Simplify the expression:
f2=12π√2L1⋅18C1=12π√28L1C1=12π√14L1C1=12π⋅12√L1C1=21⋅12π√L1C1
Therefore,
f2=2f1
7. Calculate the new frequency:
Since f1=500 kHz:
f2=2×500 kHz=1000 kHz
Final Answer:
The new resonating frequency f2 is 1000 kHz.
---
The resonating frequency f of an LCR circuit is given by the formula:
f=12π√LC
We are given that the initial resonating frequency f1 is 500 kHz. We can express this in Hz for calculations:
f1=500×103 Hz
Let the initial values of inductance and capacitance be L1 and C1 respectively. Therefore, we can write:
f1=12π√L1C1
According to the problem:
- The new inductance L2=2L1 (L is doubled)
- The new capacitance C2=18C1 (C is decreased to one-eighth)
The new resonating frequency f2 can be expressed as:
f2=12π√L2C2=12π√(2L1)(18C1)
f2=12π√2L1⋅18C1=12π√28L1C1=12π√14L1C1=12π⋅12√L1C1=21⋅12π√L1C1
Therefore,
f2=2f1
Since f1=500 kHz:
f2=2×500 kHz=1000 kHz
The new resonating frequency f2 is 1000 kHz.
Similar Questions
Knowledge Check
In a series LCR circuit having L=30mH,R=8Ω and the resonant frequency is 50 Hz. The quality factor of the circuit is
A0.118B11.8C118D1.18
In a LCR series resonating circuit. Give the value of average power loss.
View SolutionIn LCR circuit current resonant frequency is 600Hz and half power points are at 650 and 550Hz. The quality factor is
View SolutionIn a series LCR circuit, obtain an expression for the resonant frequency.
View SolutionThe resonant frequency of a circuit is f. If the capacitance is made 4 times the initial values, then the resonant frequecy will become
View SolutionA resistor R, an inductor L, a capacitor C and voltmeters V1,V2 and V3 are connected to an oscillator in the circuit as shown in the adjoining diagram.When the frequency of the oscillator is increased, upto resonance frequency, the voltmeter reading (at resonance frequency) is zero in the case of:
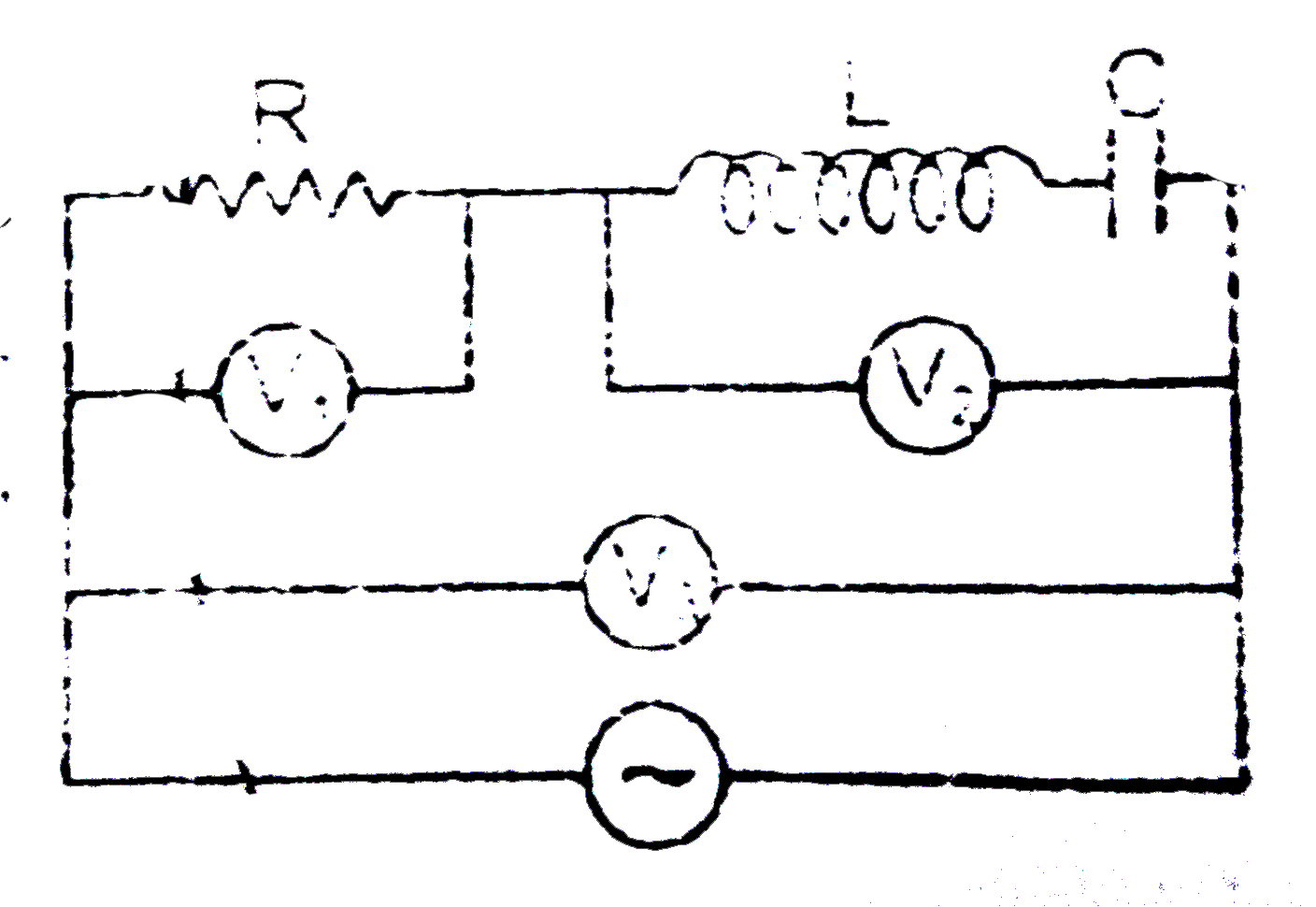
View SolutionTo reduce the resonant frequency in an LCR series circuit with a generator
View SolutionTo reduce the resonant frequency in an LCR series circuit with a generator
View Solution
