A direct current of 10 A is superimposed on an alternating current / = 40 cos ωt (A) flowing through a wire. The effective value of the resulting current will be
10√2 A
20√2 A
20√3 A
30 A
The correct Answer is:D
To find the effective value of the resulting current when a direct current (DC) of 10 A is superimposed on an alternating current (AC) given by I=40cos(ωt) A, we can follow these steps:
Step 1: Identify the Components of the Current
We have two components of current:
- Direct Current (DC): IDC=10A
- Alternating Current (AC): IAC=40cos(ωt)A
Step 2: Calculate the RMS Value of the AC Current
The RMS (Root Mean Square) value of the AC current can be calculated using the formula:
Irms=Imax√2
where Imax is the peak value of the AC current.
Here, the peak value Imax=40A, so:
Irms=40√2=401.414≈28.28A
Step 3: Calculate the Total Effective Current
The total effective current Itotal when DC and AC are superimposed is given by:
Itotal=IDC+IAC
Since the DC component does not change with time, we can combine the RMS values:
I2rms,total=I2DC+I2rms,AC
Step 4: Substitute the Values
Now we substitute the values we have:
I2rms,total=(10)2+(28.28)2
Calculating each term:
I2rms,total=100+28.282≈100+800≈900
Step 5: Calculate the Final RMS Value
Now take the square root to find Irms,total:
Irms,total=√900=30A
Conclusion
The effective value of the resulting current is:
30A
---
We have two components of current:
- Direct Current (DC): IDC=10A
- Alternating Current (AC): IAC=40cos(ωt)A
The RMS (Root Mean Square) value of the AC current can be calculated using the formula:
Irms=Imax√2
where Imax is the peak value of the AC current.
Irms=40√2=401.414≈28.28A
The total effective current Itotal when DC and AC are superimposed is given by:
Itotal=IDC+IAC
Since the DC component does not change with time, we can combine the RMS values:
I2rms,total=I2DC+I2rms,AC
Now we substitute the values we have:
I2rms,total=(10)2+(28.28)2
Calculating each term:
I2rms,total=100+28.282≈100+800≈900
Now take the square root to find Irms,total:
Irms,total=√900=30A
The effective value of the resulting current is:
30A
Similar Questions
A direct current of 5 amp is superimposed on an alternating current I=10sinωt flowing through a wire. The effective value of the resulting current will be:
View SolutionA direct current of 5 amp is superimposed on an alternating current I=10sinωt flowing through a wire. The effective value of the resulting current will be:
View SolutionIf a direct current of value a ampere is superimposed on an alternating current1=bsinωt flowing through a wire, what is the effective value of the resulting current in the circuit?
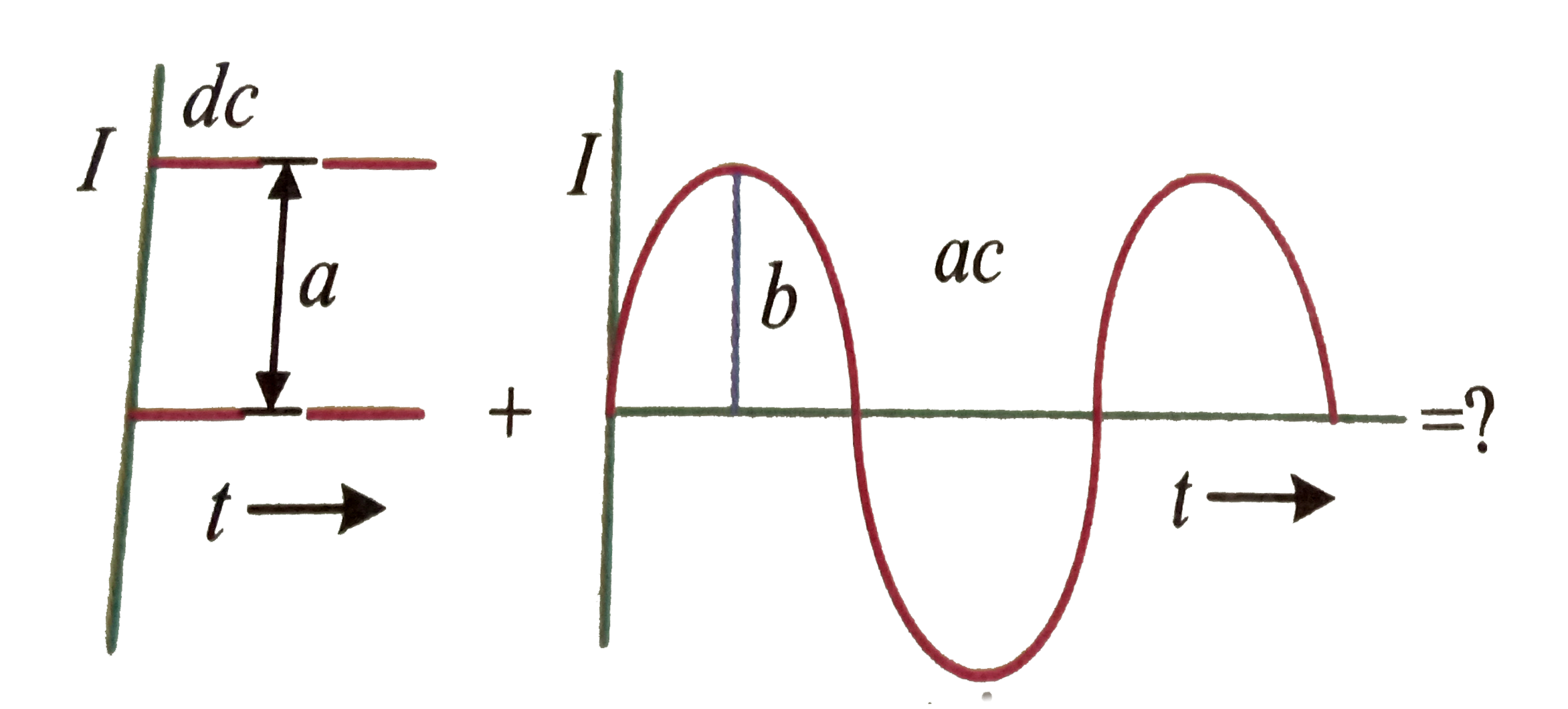
View SolutionIf a direct current of value a ampere is superimposed on an alternative current I=b sinωt flowing through a wire, what is the effective value of the resulting current in the circuit?
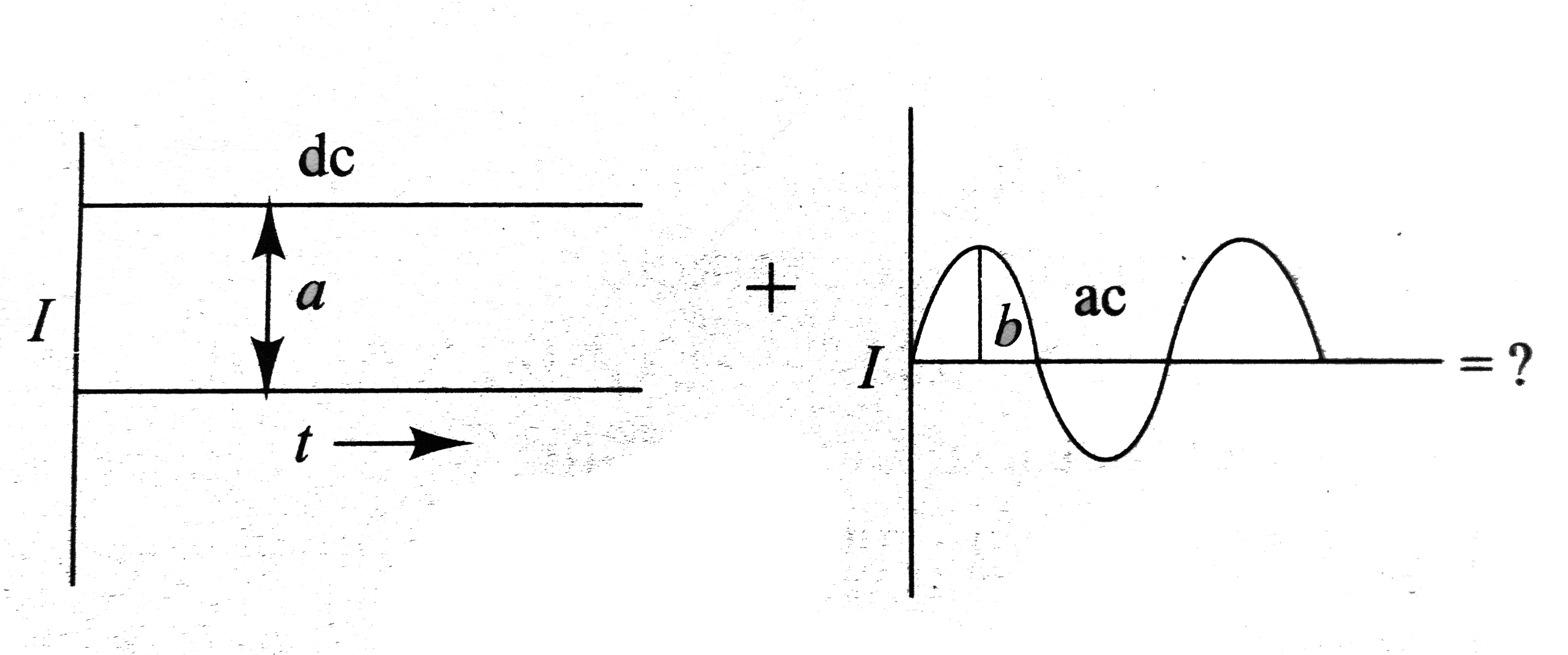
View SolutionThe rms value of an alternating current
View SolutionIf instantaneous current in a circuit is given by I=(2+3sinωt) A, then the effective value of resulting current in the circuit is
View SolutionA direct current of 2 A and an alternating current having a maximum value of 2 A flow through two identical resistances. The ratio of heat produced in the two resistances will be
View SolutionA direct current of 2 A and an alternating current having a maximum value of 2 A flow through two identical resistances. The ratio of heat produced in the two resistances will be
View SolutionA direct current of 2 A and an alternating current having a maximum value of 2 A flow through two identical resistances. The ratio of heat produced in the two resistances will be
View SolutionAn alternating current is given by
I=i1cosωt+i2sinωt.
The rms current is given by
View Solution
