The velocity of a body depends on time according to the equative v=20+0.1t2. The body is undergoing
Uniform retardation
Uniform acceleration
Non uniform acceleration
Zero acceleration
The correct Answer is:C
To solve the problem, we need to determine the nature of the acceleration of the body whose velocity is given by the equation v=20+0.1t2.
1. Identify the given equation for velocity:
v=20+0.1t2
Here, v is the velocity of the body, and t is the time.
2. Find the acceleration:
Acceleration a is defined as the rate of change of velocity with respect to time, which can be expressed mathematically as:
a=dvdt
To find a, we need to differentiate the velocity function v with respect to t.
3. Differentiate the velocity function:
- The derivative of a constant (20) is 0.
- The derivative of 0.1t2 is 0.1⋅2t=0.2t.
Therefore, the acceleration is:
a=dvdt=0+0.2t=0.2t
4. Analyze the acceleration:
The expression a=0.2t indicates that acceleration is a function of time t. This means that as time increases, the acceleration also increases.
5. Determine the nature of acceleration:
- Since the acceleration a=0.2t changes with time, it is not constant.
- A constant acceleration would mean that a does not depend on t, which is not the case here.
6. Conclusion:
The body is undergoing non-uniform acceleration because the acceleration depends on time and changes as time progresses.
v=20+0.1t2
Here, v is the velocity of the body, and t is the time.
Acceleration a is defined as the rate of change of velocity with respect to time, which can be expressed mathematically as:
a=dvdt
To find a, we need to differentiate the velocity function v with respect to t.
- The derivative of a constant (20) is 0.
- The derivative of 0.1t2 is 0.1⋅2t=0.2t.
a=dvdt=0+0.2t=0.2t
The expression a=0.2t indicates that acceleration is a function of time t. This means that as time increases, the acceleration also increases.
- Since the acceleration a=0.2t changes with time, it is not constant.
- A constant acceleration would mean that a does not depend on t, which is not the case here.
The body is undergoing non-uniform acceleration because the acceleration depends on time and changes as time progresses.
Topper's Solved these Questions
Similar Questions
The velocity of a body depends on time according to the equation v=t210+20. The body is undergoing
View SolutionThe velocity v of the a particle depends upen the time t according to the equation v=a+bt+cd+1 Write the dimension of a, b,c and d.
View SolutionA particle starts moving rectilinearly at time t=0 such that its velocity v changes with time t according to the equation v=t2−t, where t is in seconds and v in s−1. Find the time interval for which the particle retards.
View SolutionThe velocity v of a body moving along a straight line varies with time t as v=2t2e−t, where v is in m/s and t is in second. The acceleration of body is zero at t =
View SolutionThe velocity-time graph of a body is shown in .
The displacement of the body in 8s is.
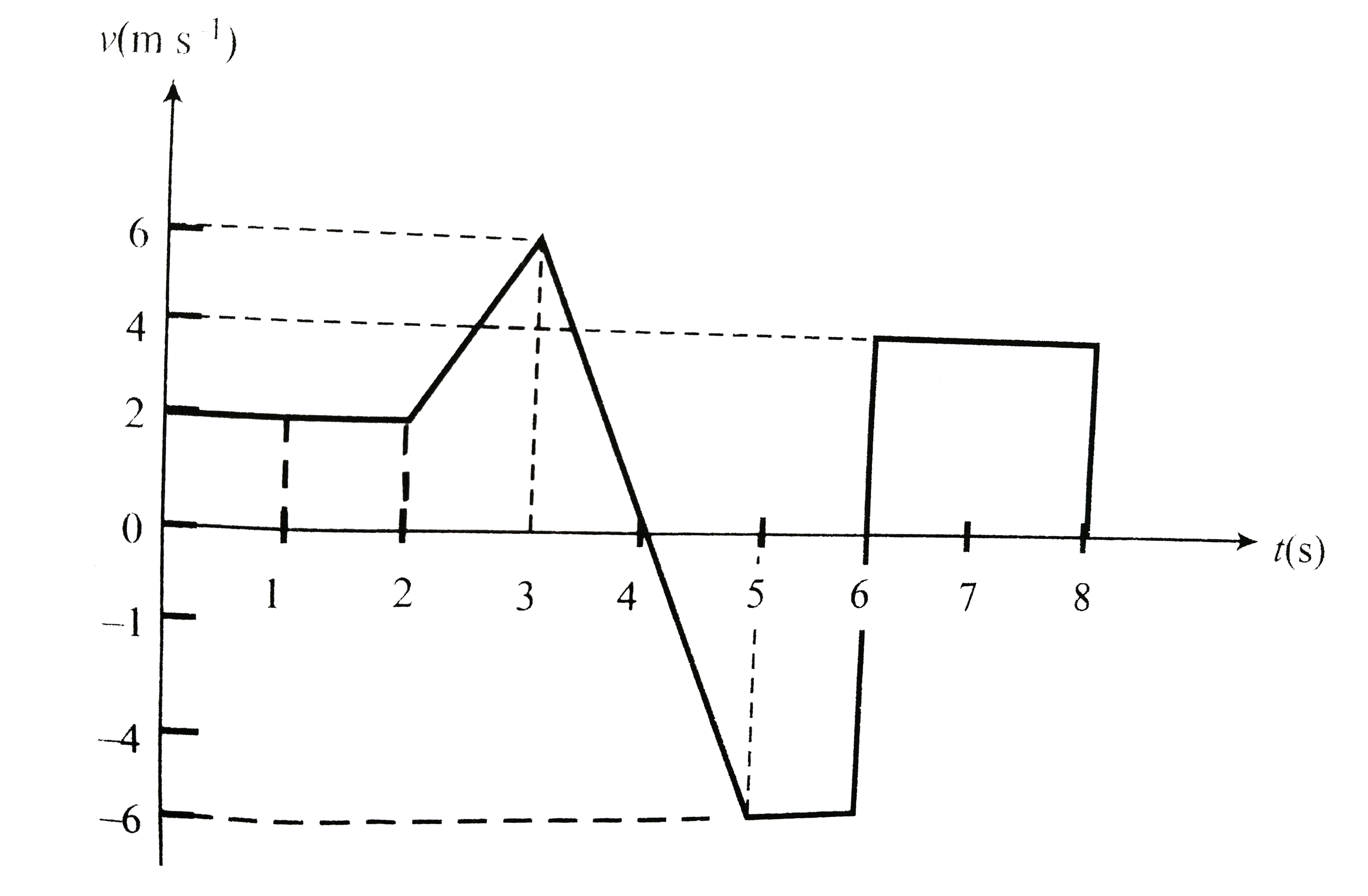 .
.
View SolutionThe displacement of a body along X-axis depends on time as √x=t+1. Then the velocity of body.
View SolutionA body is projected horizontally from a point above the ground. The motion of the body
is described by the equations x = 2 t and y=5t2 where x and y are the horizontal and vertical displacements (in m) respectively at time t. What is the magnitude of the velocity of the body 0.2 second after it is projected?
View SolutionSTATEMENT - 1 : Equation of motion (v=u+at) is applicable even if the acceleration is non-uniform.
STATEMENT - 2 : The initial velocity of a body is u and its acceleration is ft where f is a constant and t is time.
The displacement in time t is (ut+12ft2).
STATEMENT - 3 : The area enclosed by the a-t graph and time axis gives the change in velocity of the body.
View SolutionIf v is the velocity of a body moving along x-axis, then acceleration of body is
View SolutionThe position of a body moving along x-axis at time t is given by x=(t2−4t+6)m. The velocity of the body at time t = 3s is
View Solution
