The acceleration of a particle moving along a straight line at any time t is given by a = 4 - 2v, where v is the speed of particle at any time t The maximum velocity is
4 m/s
2 m/s
6 m/s
Infinity
The correct Answer is:B
To find the maximum velocity of a particle moving along a straight line with the given acceleration a=4−2v, we can follow these steps:
Step 1: Write the relationship between acceleration and velocity
We know that acceleration a can also be expressed as the derivative of velocity with respect to time:
a=dvdt
Thus, we can set up the equation:
dvdt=4−2v
Step 2: Rearrange the equation
To solve this differential equation, we can rearrange it:
dv4−2v=dt
Step 3: Integrate both sides
Now, we will integrate both sides. The left side requires a substitution:
∫dv4−2v=∫dt
The left side can be simplified by factoring out the constant:
∫12⋅12−vdv=∫dt
This gives us:
12ln|4−2v|=t+C
where C is the constant of integration.
Step 4: Solve for v
To solve for v, we can exponentiate both sides:
|4−2v|=e2(t+C)=e2t⋅e2C
Let K=e2C, then:
4−2v=Ke2t
Solving for v:
2v=4−Ke2t
v=2−K2e2t
Step 5: Determine the maximum velocity
To find the maximum velocity, we need to consider the limit as t approaches infinity. As t→∞, the term e2t grows very large, and thus K2e2t will dominate unless K is zero. For maximum velocity, we want the negative term to vanish:
vmax=2−0=2
Conclusion
Thus, the maximum velocity vmax is:
2 m/s
We know that acceleration a can also be expressed as the derivative of velocity with respect to time:
a=dvdt
Thus, we can set up the equation:
dvdt=4−2v
To solve this differential equation, we can rearrange it:
dv4−2v=dt
Now, we will integrate both sides. The left side requires a substitution:
∫dv4−2v=∫dt
The left side can be simplified by factoring out the constant:
∫12⋅12−vdv=∫dt
This gives us:
12ln|4−2v|=t+C
where C is the constant of integration.
To solve for v, we can exponentiate both sides:
|4−2v|=e2(t+C)=e2t⋅e2C
Let K=e2C, then:
4−2v=Ke2t
Solving for v:
2v=4−Ke2t
v=2−K2e2t
To find the maximum velocity, we need to consider the limit as t approaches infinity. As t→∞, the term e2t grows very large, and thus K2e2t will dominate unless K is zero. For maximum velocity, we want the negative term to vanish:
vmax=2−0=2
Thus, the maximum velocity vmax is:
2 m/s
Topper's Solved these Questions
Similar Questions
Velocity of a particle moving along a straight line at any time 't' is give by V=cos(π3t) . Then the distance travelled by the particle in the first two seconds is :
View SolutionThe acceleration-time graph of a particle moving along a straight line is as shown in. At what time the particle acquires its initial velocity?
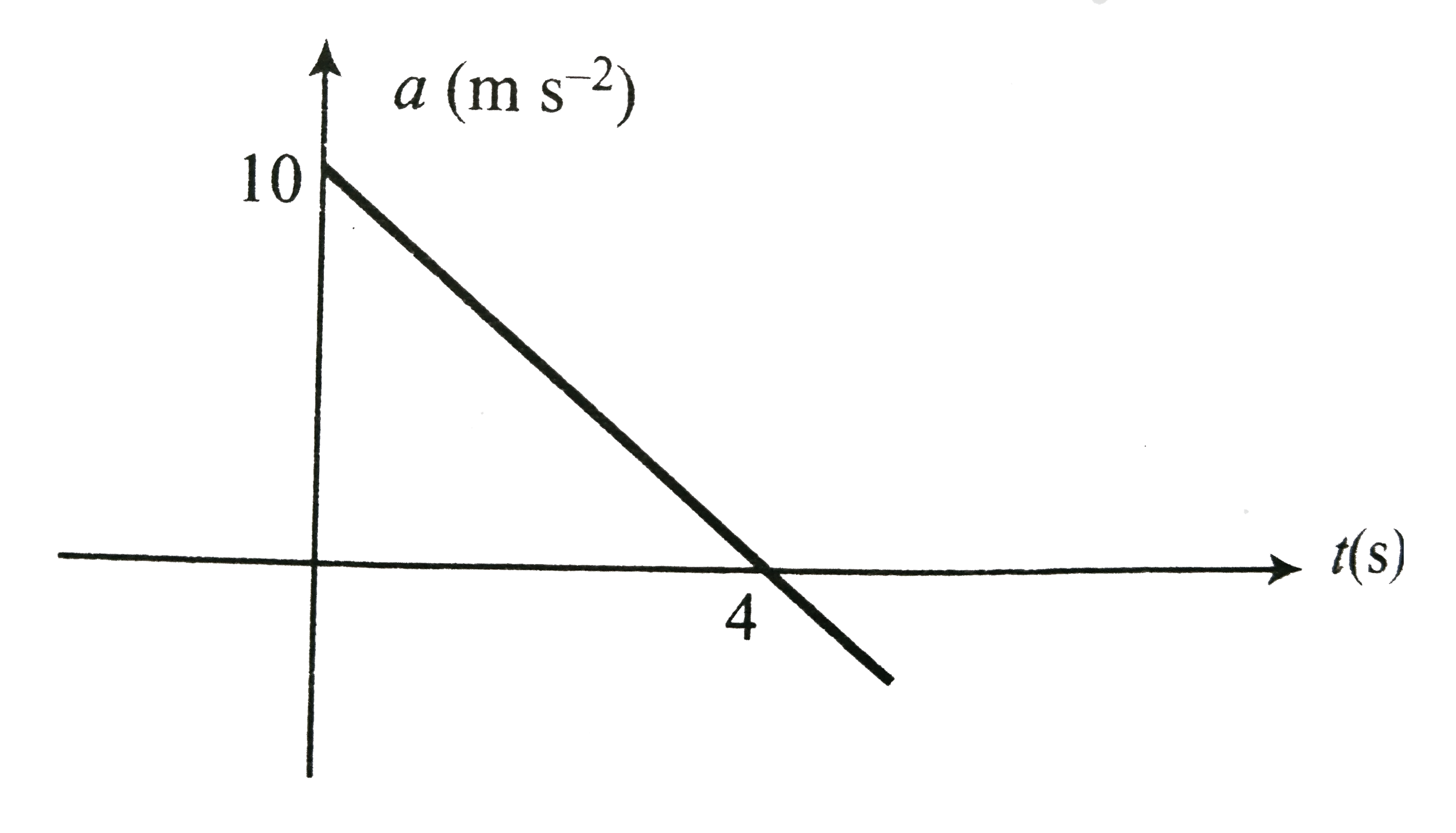 .
.
View SolutionThe acceleration-time graph of a particle moving along a straight line is as shown in. At what time the particle acquires its initial velocity?
 .
.
View SolutionKinetic energy of a particle moving in a straight line varies with time t as K=4t2. The force acting on the particle
View SolutionAt any instant, the velocity and acceleration of a particle moving along a straight line are v and a. The speed of the particle is increasing if
View SolutionThe displacement x of a particle moving along x-axis at time t is given by x2=2t2+6t. The velocity at any time t is
View SolutionAcceleration time graph of a particle moving along a straight line is given. Then average acceleration between t=0 & t=4 is :−
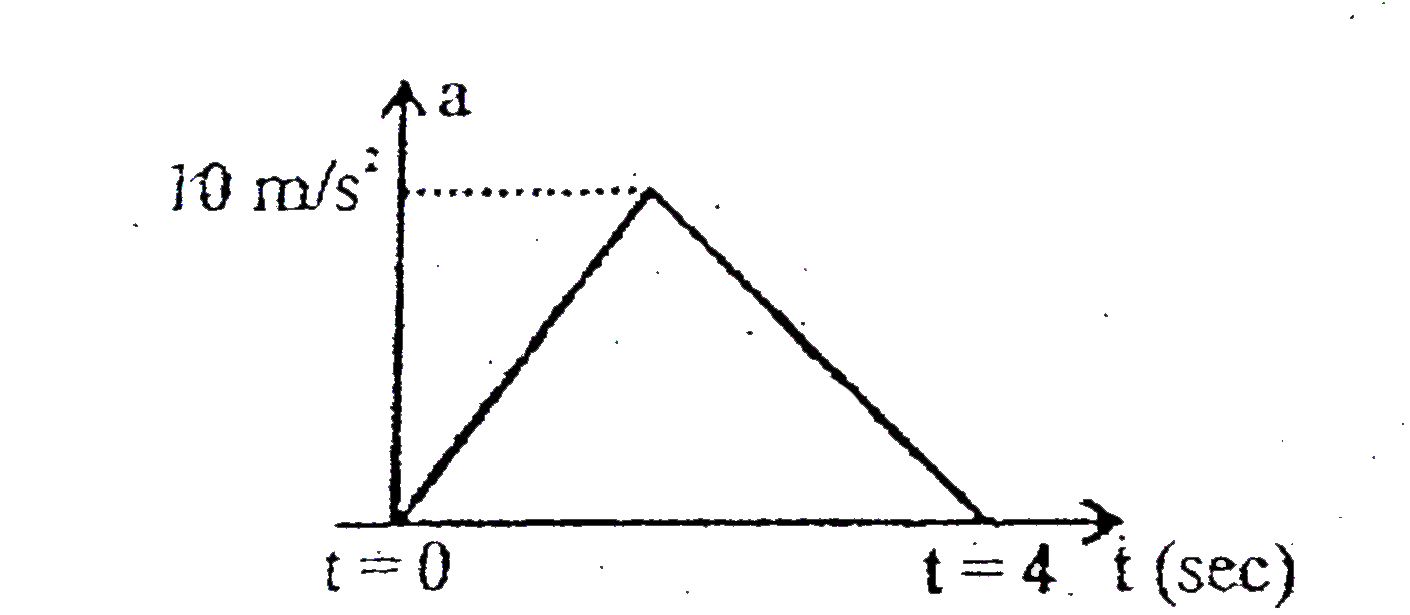
View SolutionThe speed v of a particle moving along a straight line is given by a+bv2=x2 where x is its distance from the origin. The acceleration of the particle is
View SolutionThe coordinates of a moving particle at any time t are given by x=αt3 and y=βt3. The speed of the particle at time t is given by
View SolutionThe speed(v) of a particle moving along a straight line is given by v=(t2+3t−4 where v is in m/s and t in seconds. Find time t at which the particle will momentarily come to rest.
View Solution