At the top of the trajectory of projectile the
Acceleration is minimum
Velocity is zero
Acceleration is maximum
Acceleration is g
The correct Answer is:D
To solve the question regarding the projectile motion at the top of its trajectory, we will analyze the components of velocity and acceleration at that point.
1. Understanding the Projectile Motion:
- A projectile is launched with an initial velocity u at an angle θ with the horizontal. The motion can be analyzed in two dimensions: horizontal (x-direction) and vertical (y-direction).
2. Components of Initial Velocity:
- The initial velocity can be broken down into two components:
- Horizontal component: ux=ucosθ
- Vertical component: uy=usinθ
3. At the Top of the Trajectory:
- At the highest point of the projectile's path (the top of the trajectory), the vertical component of the velocity becomes zero. This is because the projectile has reached its maximum height and is about to start descending.
4. Velocity at the Highest Point:
- While the vertical component of the velocity is zero at the highest point, the horizontal component remains unchanged throughout the motion (since there is no horizontal acceleration). Thus, the net velocity at the top is:
v=ucosθ
- Therefore, the velocity is not zero; it has a horizontal component.
5. Acceleration at the Highest Point:
- The only force acting on the projectile is gravity, which acts downward with an acceleration of g. This acceleration remains constant throughout the motion, including at the highest point.
- Hence, the acceleration at the top of the trajectory is:
a=−g
- This means the acceleration is equal to g (in magnitude) and directed downwards.
6. Conclusion:
- At the top of the trajectory:
- The vertical component of velocity is zero.
- The horizontal component of velocity is ucosθ.
- The acceleration is constant and equal to g (downward).
Final Answer:
- The acceleration at the top of the trajectory is g (downward), and the velocity is not zero; it is ucosθ (horizontal).
- A projectile is launched with an initial velocity u at an angle θ with the horizontal. The motion can be analyzed in two dimensions: horizontal (x-direction) and vertical (y-direction).
- The initial velocity can be broken down into two components:
- Horizontal component: ux=ucosθ
- Vertical component: uy=usinθ
- At the highest point of the projectile's path (the top of the trajectory), the vertical component of the velocity becomes zero. This is because the projectile has reached its maximum height and is about to start descending.
- While the vertical component of the velocity is zero at the highest point, the horizontal component remains unchanged throughout the motion (since there is no horizontal acceleration). Thus, the net velocity at the top is:
v=ucosθ
- Therefore, the velocity is not zero; it has a horizontal component.
- The only force acting on the projectile is gravity, which acts downward with an acceleration of g. This acceleration remains constant throughout the motion, including at the highest point.
- Hence, the acceleration at the top of the trajectory is:
a=−g
- This means the acceleration is equal to g (in magnitude) and directed downwards.
- At the top of the trajectory:
- The vertical component of velocity is zero.
- The horizontal component of velocity is ucosθ.
- The acceleration is constant and equal to g (downward).
- The acceleration at the top of the trajectory is g (downward), and the velocity is not zero; it is ucosθ (horizontal).
Topper's Solved these Questions
Similar Questions
Knowledge Check
At the top of the trajectory of a projectile, the directions of its velocity and acceleration are
Aperpendicular to each otherBparallel to each otherCinclined to each other at an angle of 45∘Danit-parallel to each other
A projectile has
View SolutionWhat is the angle made by the trajectory of a projectile with horizontal at maximum height ?
View SolutionA point P on the trajectory of a projectile projected at angle with θ with horizontal subtrends angles α and β at the point of landing them
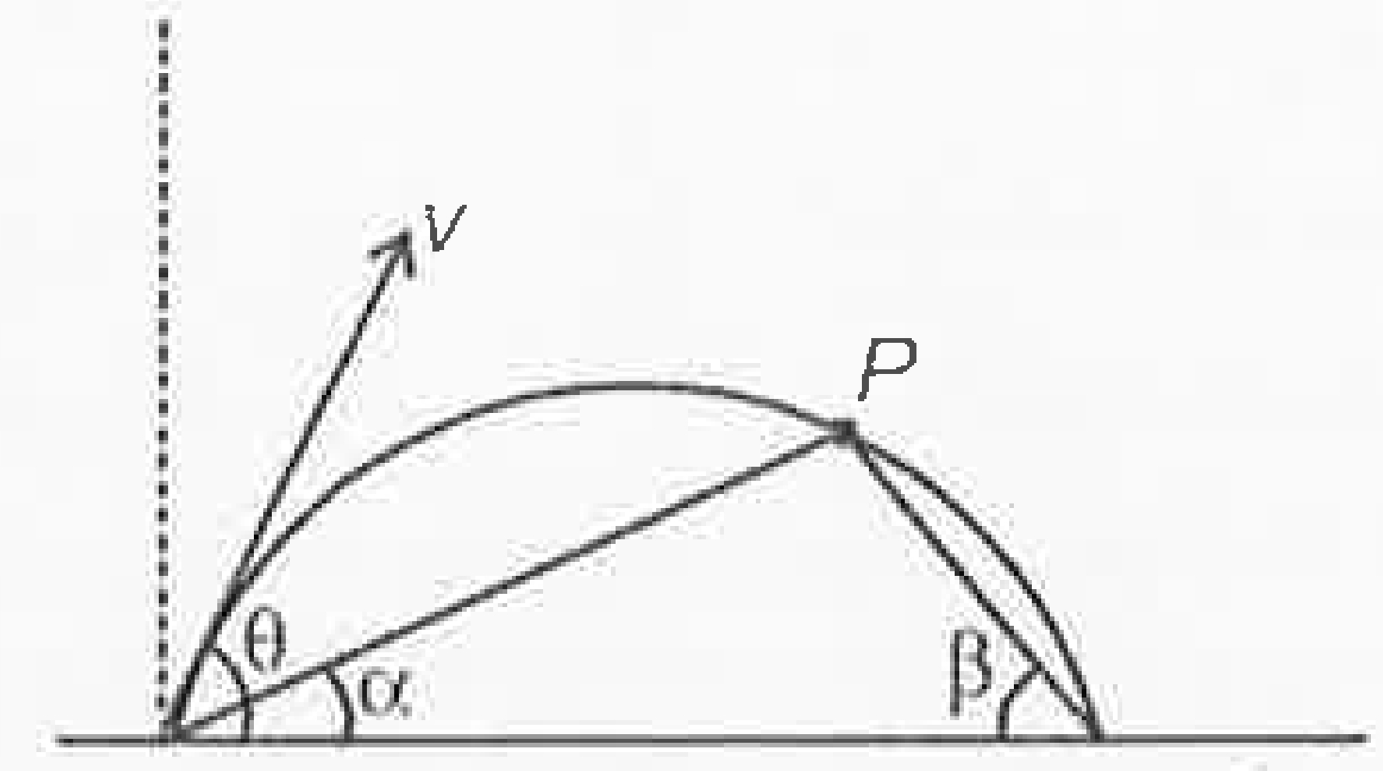
View SolutionA shell fired along a parabolic path explodes into two fragment of equal mass at the top of the trajectory. One of the fragment returns to the point of firing having retraced its original path. If v is the velocity of projectile at highest point, than
View SolutionThe equation of trajectory ofa projectile is y=10x−59x2 If we assume g=10ms−2, the range of projectile (in metre) is
View SolutionAssertion:− The trajectory of projectile in XY plane is quadratic in x and linear in y if x is independent of X−coordinate.
Reason :− y−coordinate of trajetory is independent of x−coordinate.
View SolutionA projectile is projected in air with initial velocity →v=(3ˆi+4ˆj) m/s from the origin. The equation of trajectory of the projectile is given as (g=−10ˆjms2) (neglect air resistance )
View Solution
