Potential energy of a particle at position x is given by U=(x2−4x)J. Which of the following is equilibrium position of the particle?
x=0
x=5m
x=2m
x=4m
The correct Answer is:C
To find the equilibrium position of a particle given its potential energy function U=x2−4x, we need to follow these steps:
Step 1: Understand the relationship between force and potential energy
The force F acting on a particle is related to the potential energy U by the equation:
F=−dUdx
At equilibrium, the net force acting on the particle is zero, which means:
F=0⟹−dUdx=0⟹dUdx=0
Step 2: Differentiate the potential energy function
Given the potential energy function:
U=x2−4x
we need to differentiate U with respect to x:
dUdx=ddx(x2)−ddx(4x)
Calculating the derivatives:
dUdx=2x−4
Step 3: Set the derivative equal to zero
To find the equilibrium position, we set the derivative equal to zero:
2x−4=0
Step 4: Solve for x
Now, we solve for x:
2x=4⟹x=42=2
Conclusion
The equilibrium position of the particle is at:
x=2 meters
The force F acting on a particle is related to the potential energy U by the equation:
F=−dUdx
At equilibrium, the net force acting on the particle is zero, which means:
F=0⟹−dUdx=0⟹dUdx=0
Given the potential energy function:
U=x2−4x
we need to differentiate U with respect to x:
dUdx=ddx(x2)−ddx(4x)
Calculating the derivatives:
dUdx=2x−4
To find the equilibrium position, we set the derivative equal to zero:
2x−4=0
Now, we solve for x:
2x=4⟹x=42=2
The equilibrium position of the particle is at:
x=2 meters
Topper's Solved these Questions
Similar Questions
Potential energy of a particle at position x is given by U=x2−5x. Which of the following is equilibrium position of the particle?
View SolutionThe potential energy of a particle under a conservative force is given by U(x)=(x2−3x) J. The equilibrium position of the particle is at
View SolutionThe potential energy U(x) of a particle moving along x - axis is given by U(x)=ax−bx2. Find the equilibrium position of particle.
View SolutionPotential energy of a particle is related to x coordinate by equation x2−2x . Particle will be in stable equilibrium at :
View SolutionIn the figure shown the potential energy U of a particle is plotted its position 'x' from origin. Then which of the following statement is correct A particle at :
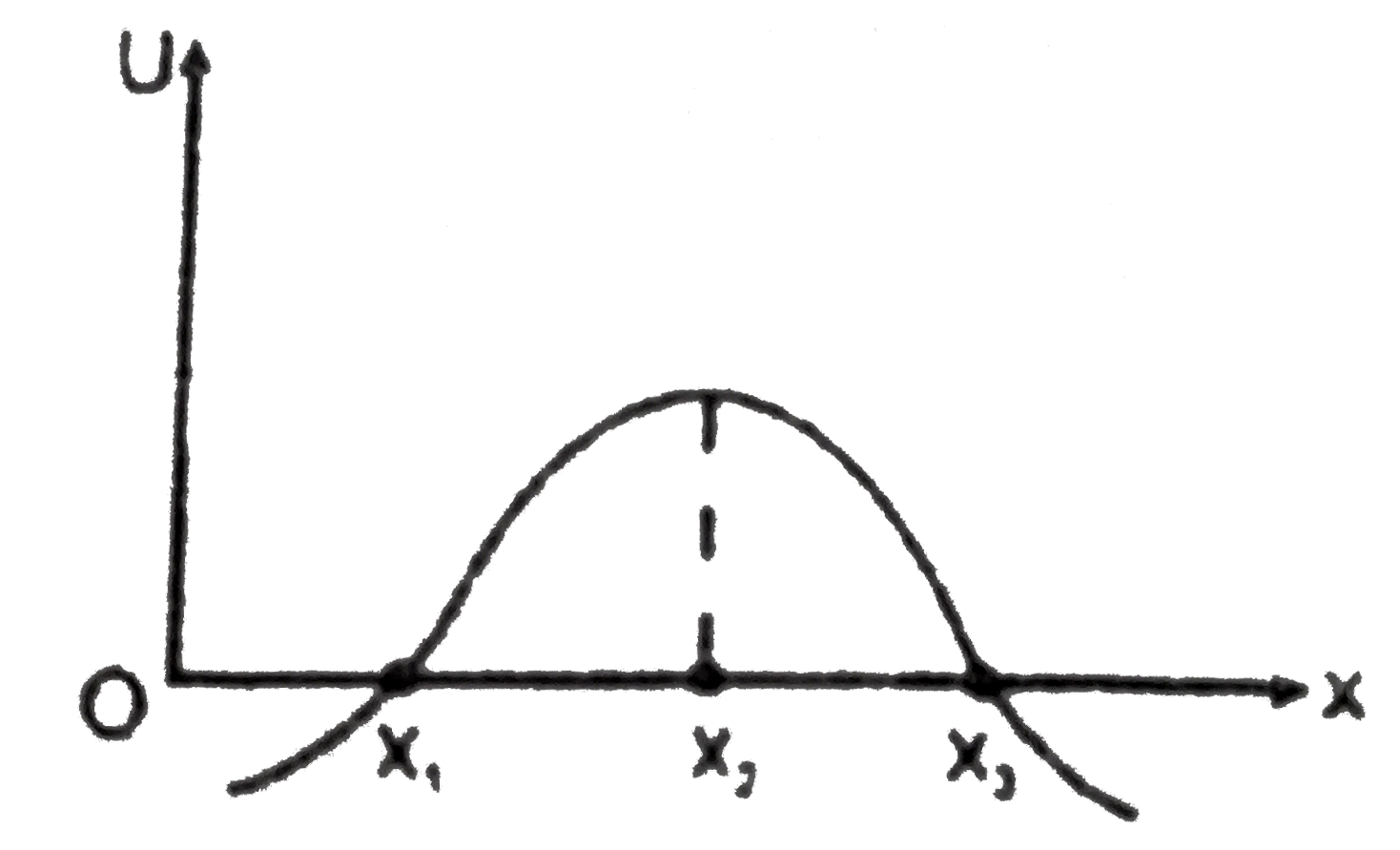
View SolutionPotential energy of a particle moving along x-axis is by
U=(x33−4x+6).
here, U is in joule and x in metre. Find position of stable and unstable equilibrium.
View SolutionIf for a particle position x∝t2 then :-
View SolutionThe potential energy of a particle in motion along X axis is given by U=U0−u0 cos ax. The time period of small oscillation is
View Solutionpotential enrgy of a particle along x-axis varies as, U=−20+(x−2)2, where U is in joule and x in meter. Find the equilibrium position and state whether it is stable or unstable equilibrium.
View SolutionThe potential energy of a particle of mass 5 kg moving in the x−y plane is given by U=(−7x+24y)J, where x and y are given in metre. If the particle starts from rest, from the origin, then the speed of the particle at t=2s is
View Solution
