The correct statement of the following,is
All free charged particles move from the region of lower electric potential to region of higher electric potential.
Electrostatic field inside a conductor is Zero
Surface of any conductor has uniform charge density in eletrostatic condition.
Both (1) and (3)
To solve the question regarding the correctness of the statements provided, let's analyze each statement step by step.
Step 1: Analyze Statement 1
Statement 1: "All free charged particles move from a region of lower electric potential to a region of higher electric potential."
- Analysis: This statement is incorrect. In electrostatics, positive charges move from higher to lower potential, while negative charges (which are free charged particles) move from lower to higher potential. Therefore, this statement does not hold true.
Hint: Remember the behavior of positive and negative charges in an electric field when considering electric potential.
Step 2: Analyze Statement 2
Statement 2: "Electrostatic field inside the conductor is zero."
- Analysis: This statement is correct. In electrostatic equilibrium, the electric field inside a conductor is always zero. This is because any electric field would cause the free charges to move, contradicting the condition of electrostatic equilibrium.
Hint: Recall that in electrostatic conditions, charges redistribute themselves until the electric field inside the conductor is neutralized.
Step 3: Analyze Statement 3
Statement 3: "Surface of any conductor has uniform charge density in electrostatic condition."
- Analysis: This statement is incorrect. While the surface charge density can be uniform in some cases, it is not true for all conductors, especially those with sharp points or irregular shapes. At sharp points, the surface charge density is higher due to the concentration of electric field lines.
Hint: Consider how charge distribution varies on conductors with different geometries.
Step 4: Analyze Statement 4
Statement 4: "Both statement 1 and 3 are correct."
- Analysis: Since both statement 1 and statement 3 have been identified as incorrect, this statement is also incorrect.
Conclusion
Based on the analysis:
- Statement 1: Incorrect
- Statement 2: Correct
- Statement 3: Incorrect
- Statement 4: Incorrect
Thus, the correct answer is that only Statement 2 is correct.
Final Answer
The correct statement is: Statement 2 is correct.
Statement 1: "All free charged particles move from a region of lower electric potential to a region of higher electric potential."
Statement 2: "Electrostatic field inside the conductor is zero."
Statement 3: "Surface of any conductor has uniform charge density in electrostatic condition."
Statement 4: "Both statement 1 and 3 are correct."
Based on the analysis:
- Statement 1: Incorrect
- Statement 2: Correct
- Statement 3: Incorrect
- Statement 4: Incorrect
The correct statement is: Statement 2 is correct.
Topper's Solved these Questions
- TEST 1BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE
21 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Try Yourself
63 Videos
- TEST 2BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 2EXERCISE - Exercise
308 Videos
Similar Questions
Knowledge Check
The correct statement out of the following is :
AThe boiling point of the solution falls on increasing the amount of soluteBThe boiling point of solution increases on diluting the solutionCThe freezing point of the solution is raised on adding more of soluteDThe freezing point of the solution decreases on increasing the amount of solute.
The correct statement among the following is:
View SolutionThe correct statement among the following is:
View SolutionThe correct statement among the following is/are
View SolutionThe correct statement from the following is
View SolutionSelect the correct statement form the following
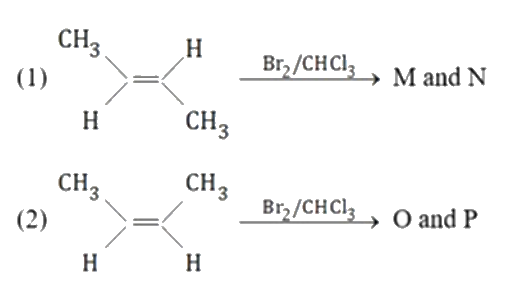
View SolutionThe correct statement for the following addition reaction is
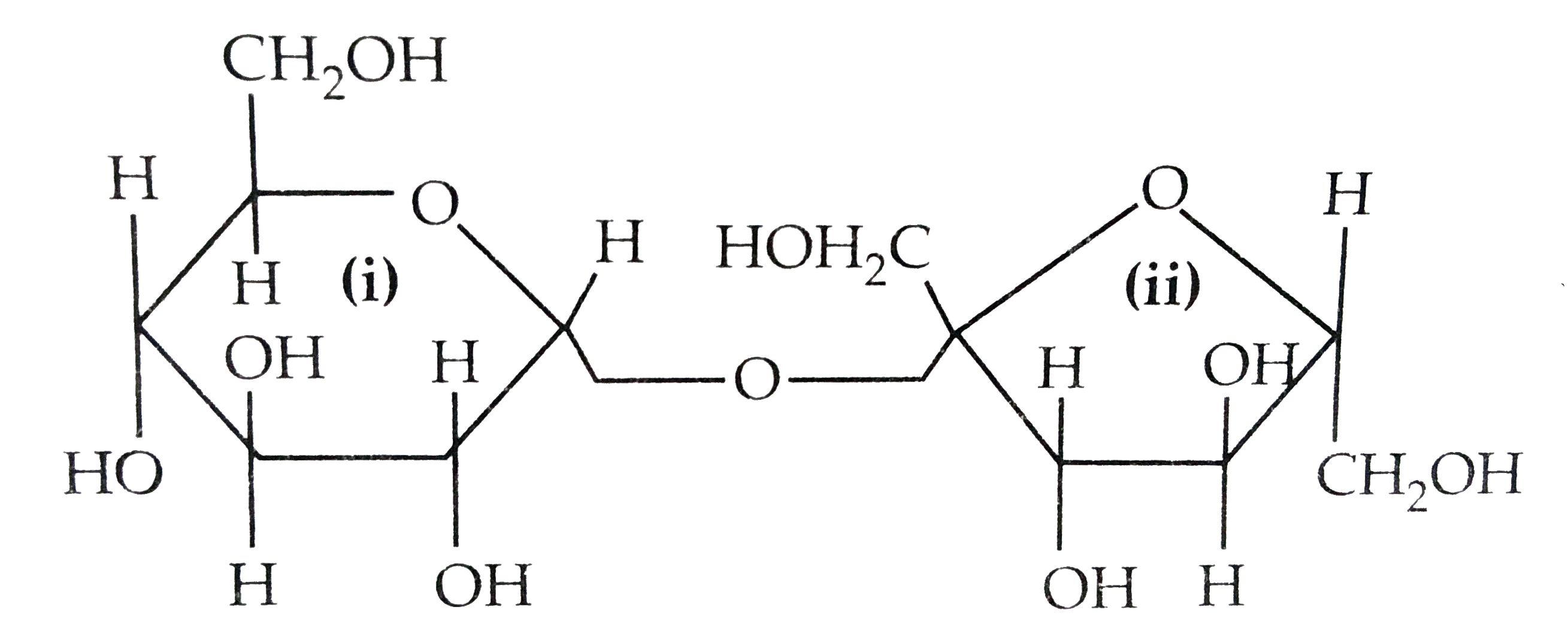
View SolutionThe correct statement about the following disaccharide
View Solution
