If a solid cylinder of area of cross-section A is moving with velocity V in medium of density p then power loss of cylinder is
To find the power loss of a solid cylinder of cross-sectional area A moving with velocity V in a medium of density ρ, we can follow these steps:
Step 1: Understand the concept of power
Power is defined as the rate at which work is done or energy is transferred. Mathematically, it can be expressed as:
P=Wt
where P is power, W is work done, and t is time.
Step 2: Determine the work done against the medium
When the cylinder moves through the medium, it experiences a drag force due to the medium. The work done against this drag force can be expressed as:
W=F⋅d
where F is the drag force and d is the distance moved.
Step 3: Calculate the drag force
The drag force F acting on a solid object moving through a fluid can be expressed as:
F=ρ⋅A⋅V2
where ρ is the density of the medium, A is the cross-sectional area of the cylinder, and V is the velocity of the cylinder.
Step 4: Relate distance and time
The distance d moved by the cylinder in time t can be expressed as:
d=V⋅t
Step 5: Substitute into the work equation
Substituting the expression for d into the work equation gives:
W=F⋅(V⋅t)=(ρ⋅A⋅V2)⋅(V⋅t)=ρ⋅A⋅V3⋅t
Step 6: Substitute work into the power equation
Now, substituting the expression for W into the power equation:
P=Wt=ρ⋅A⋅V3⋅tt=ρ⋅A⋅V3
Final Result
Thus, the power loss of the cylinder is given by:
P=ρ⋅A⋅V3
Power is defined as the rate at which work is done or energy is transferred. Mathematically, it can be expressed as:
P=Wt
where P is power, W is work done, and t is time.
When the cylinder moves through the medium, it experiences a drag force due to the medium. The work done against this drag force can be expressed as:
W=F⋅d
where F is the drag force and d is the distance moved.
The drag force F acting on a solid object moving through a fluid can be expressed as:
F=ρ⋅A⋅V2
where ρ is the density of the medium, A is the cross-sectional area of the cylinder, and V is the velocity of the cylinder.
The distance d moved by the cylinder in time t can be expressed as:
d=V⋅t
Substituting the expression for d into the work equation gives:
W=F⋅(V⋅t)=(ρ⋅A⋅V2)⋅(V⋅t)=ρ⋅A⋅V3⋅t
Now, substituting the expression for W into the power equation:
P=Wt=ρ⋅A⋅V3⋅tt=ρ⋅A⋅V3
Thus, the power loss of the cylinder is given by:
P=ρ⋅A⋅V3
Topper's Solved these Questions
Similar Questions
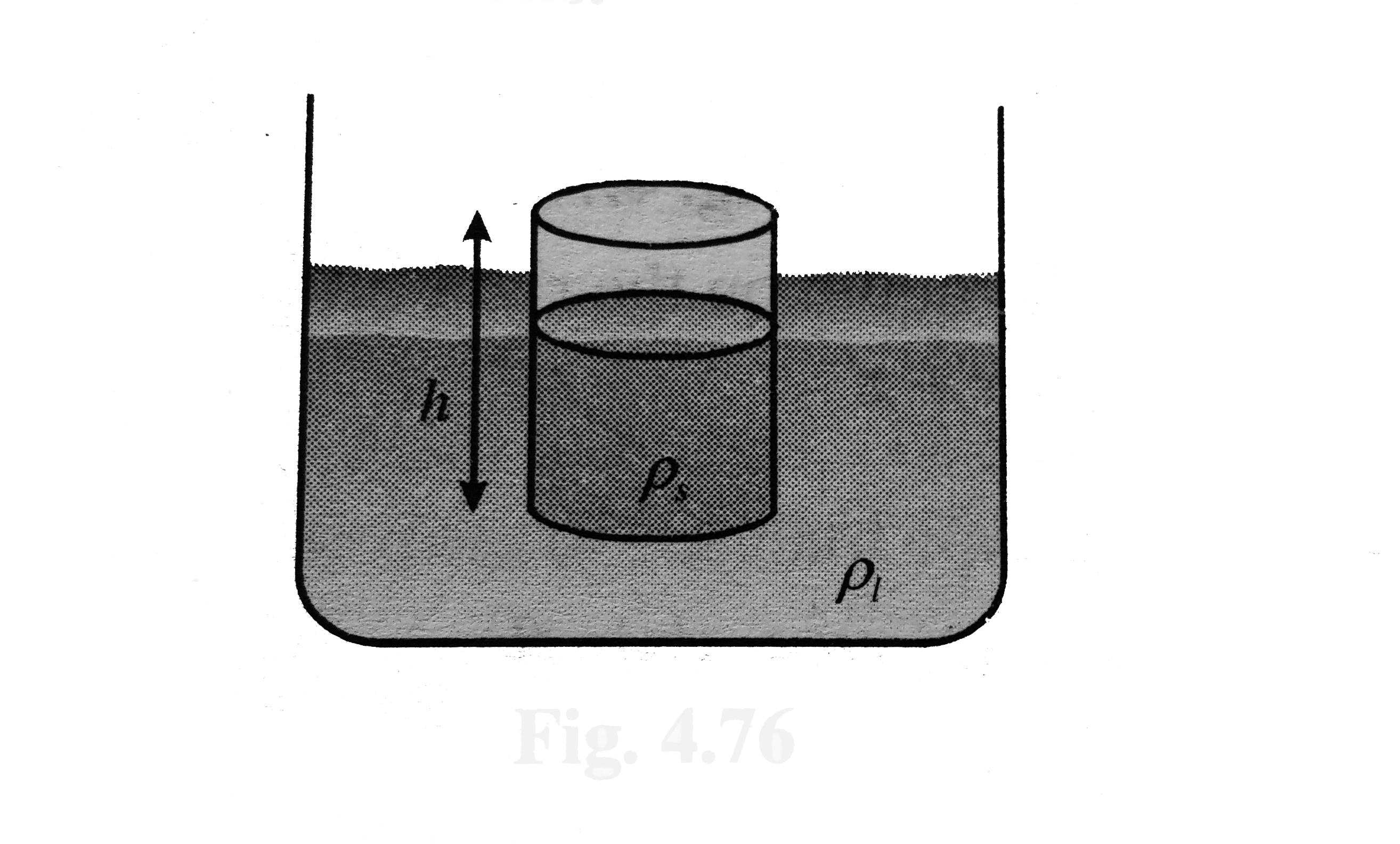
Consider a solid cylinder of the density ρs cross section area A and h ploating in a liquid of density ρl as shown in figure (ρl>ρs) . It is depressed sligtly and allowed to oscillation.
View SolutionA solid cylinder of mass 20kg rotates about its axis with angular velocity of 100 radian s−1. The radius of the cylinder is 0.25m. The magnitude of the angular momentum of the cylinder about its axis of rotation is
View Solution
Knowledge Check
A solid cylinder rolls up an inclined plane of inclination θ with an initial velocity v. How far does the cylinder go up the plane ?
A3v22gsinθBv24gsinθC3v2gsinθD3v24gsinθ
The length of a current-carrying cylindrical conductor is l, its area of cross-section is A, the number density of free electrons in it is n, and the drift velocity of electrons in it is vd . The number of electrons passing through a particular cross-section of the conductor per unit time is given by:
View SolutionVelocity of the centre of a small cylinder is v. There is no slipping anywhere. The velocity of the centre of the larger cylinder is

View SolutionA tank of height H and base area A is half filled with water and there is a small orifice at the bottom and there is a heavy solid cylinder having base area A3 and height of the cylinder is same as that of the tak. The water is flowing out of the orifice. Here cylinder is put into the tank to increase the speed of water flowing out.
After long time, when the height of water inside the tank again becomes equal to H2, the solid cylinder is taken out. then the velocity of liquid flowing out of the orifice (just after removing the cylinder) will be
View SolutionA solid cylinder of mass M and radius R rolls down an inclined plane of height h. The angular velocity of the cylinder when it reaches the bottom of the plane will be :
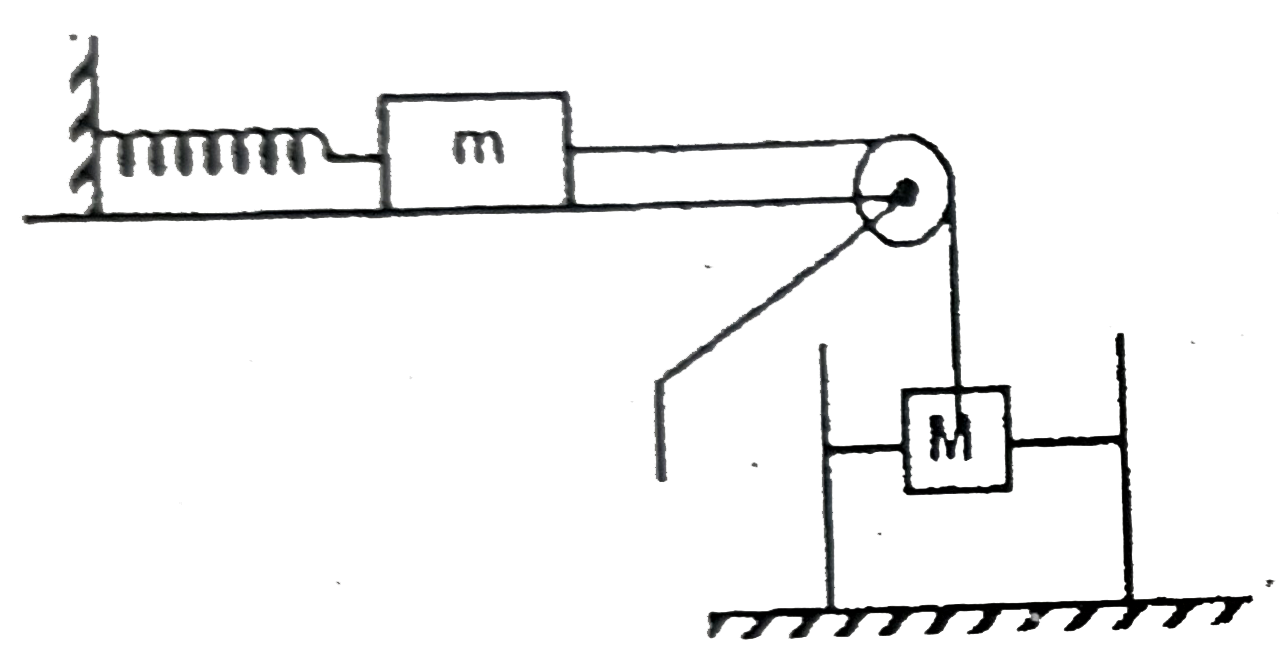
View SolutionFor the arrangement shown, a cylinder of mass m with cross-sectional area A, initially in equilibrium poisition, is displaced slightly inside the liquid of density p. Prove that the motion is simple harmonic and also find its time-period.
View SolutionA bullet of mass m moving with a velocity of u just grazes the top of a solid cylinder of mass M and radius R resting on a rough horizontal surface as shown and is embedded in the cylinder after impact. Assuming that the cylinder rolls without slipping, find the angular velocity of the cylinder and the final velocity of the bullet.

View SolutionA solid cylinder of denisty ρ0, cross-section area A and length l floats in a liquid ρ(>ρ0 with its axis vertical, . If it is slightly displaced downward and released, the time period will be :
View Solution
