Tensile stress is possible in
Liquids
Gases
Solids
Both (1) & (2)
To determine where tensile stress is possible, let's analyze the options provided step by step.
Step 1: Understand Tensile Stress
Tensile stress is defined as the stress that occurs when a material is subjected to a pulling force. It is associated with the change in length of the material when a force is applied. The formula for tensile stress (σ) is given by:
σ=FA
where:
- F is the applied force,
- A is the cross-sectional area of the material.
Step 2: Analyze the Options
1. Liquids: Liquids cannot sustain tensile stress because they do not have a fixed shape and will flow under tension. They can only support compressive stress.
2. Gases: Similar to liquids, gases cannot sustain tensile stress as they also do not have a fixed shape and will expand to fill their container. They can only support compressive stress.
3. Solids: Solids can sustain tensile stress because they have a definite shape and can resist deformation under tension. When a force is applied to a solid, it can elongate, which is the characteristic of tensile stress.
4. Both 1 and 2: This option suggests that both liquids and gases can sustain tensile stress, which is incorrect based on our analysis.
Step 3: Conclusion
Based on the analysis, tensile stress is only possible in solids. Therefore, the correct answer is:
Option 3: Solids
---
Tensile stress is defined as the stress that occurs when a material is subjected to a pulling force. It is associated with the change in length of the material when a force is applied. The formula for tensile stress (σ) is given by:
- F is the applied force,
- A is the cross-sectional area of the material.
1. Liquids: Liquids cannot sustain tensile stress because they do not have a fixed shape and will flow under tension. They can only support compressive stress.
2. Gases: Similar to liquids, gases cannot sustain tensile stress as they also do not have a fixed shape and will expand to fill their container. They can only support compressive stress.
Based on the analysis, tensile stress is only possible in solids. Therefore, the correct answer is:
Similar Questions
According to Hooke's law, within the elastic limit stress/strain = constant. This constant depends on the type of strain or the type of force acting. Tensile stress might result in compressional or elongative strain, however, a tangential stress can only cause a shearing strain. After crossing the elastic limit, the material undergoes elongation and beyond a stage beaks. All modulus of elasticity are basically constants for the materials under stress.
If stress/strain is x in elastic region and y in the region of yield, then
View Solution(i) State Hook's law.
(ii) Draw a labelled graph of tensile stress against tensile strain for a metal wire upto the breaking point. Shhow on your graph the region in which Hooke's law is obeyed.
What is the significance of the area between the graph and the strain axis withing the Hooke's law region?
View Solution
Knowledge Check
The ratio of tensile stress to the longitudinal strain is defined as
AÂ bulk modulusBYoung's modulusCshear modulusDcompressibility
A bar of cross section A is subjected to equal and opposite tensile forces F at its ends. Consider a plane through the bar making an angle θ with a plane at right angles to the bar as shown in figure.
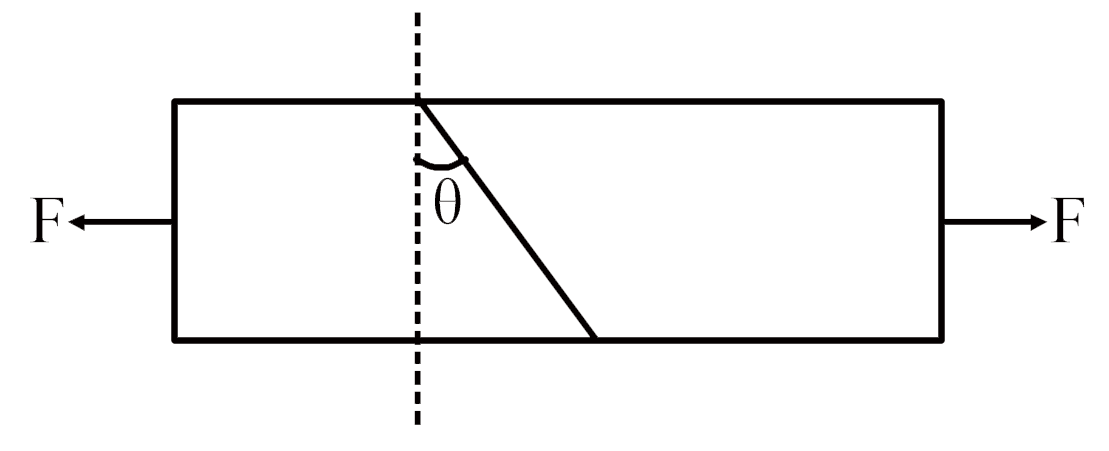
The tensile stress at this plane in terms of F, A and θ is
View SolutionWhat is the difference between tensile stress and volume stress?
View SolutionA massless uniform rod is subjected to froce F at its free end as shown in figure. The ratio of tensile stress at plane P1 to stress at P2 is
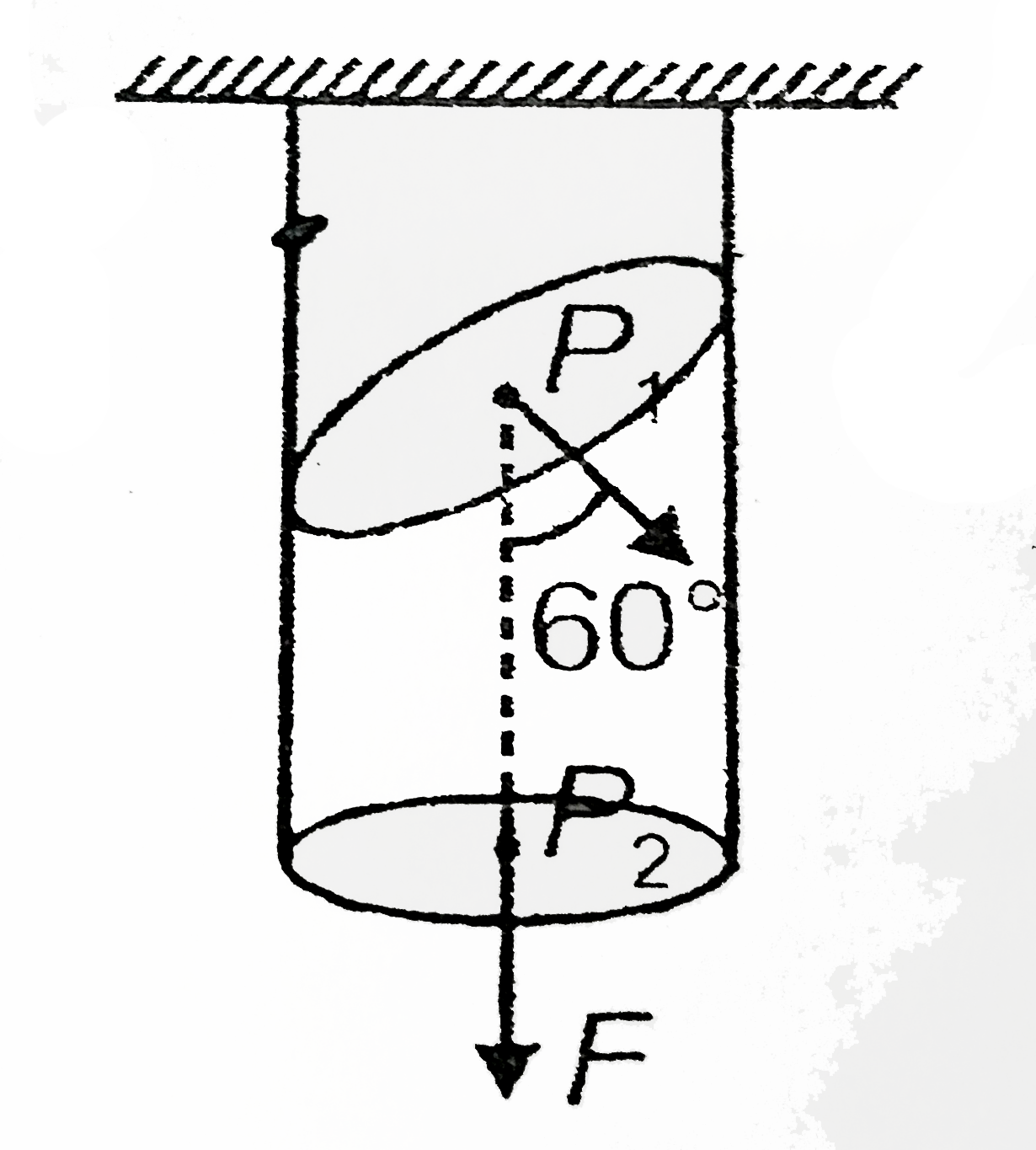
View SolutionA steel wire having a radius of 2.0mm, carrying a load of 4kg, is hanging from a celling. Given that g=3.1πms−2, what will be the tensile stress that would be developed in the wire?
View SolutionA load of 4.0 kg is suspended from a ceiling through a steel wire of radius 2.0 mm. Find the tensile stress developed in the wire when equilibrium is achieved. Take g=3.1πms−2
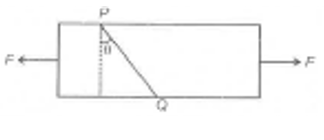
View SolutionConsider a long steel bar under a tensile stress due to forces F, acting at the edges along the length of the bar

Consider a plane making an angle θ with the length. What are the tensile and shering stresses on this plane ? (a) For what angle is the tensile stress a maximum ? (b) For what angle is the shearing stress a maximum ?
View SolutionA long rod of steel under a tensile stress due to the force acting on the edges along the length. The value of angle 0 for which shearing stress is maximum is
View Solution