Linear mass density of a rod AB(of≤n>h10m) varies with distance x from its end A as λ =λ0x3 (λ0 is positive constant) . Distance of centre of mass of the rod , from end B is
8m
2m
6m
4m
To find the distance of the center of mass of the rod from end B, we can follow these steps:
Step 1: Understanding the Problem
We have a rod AB of length 10 m, and the linear mass density λ varies with distance x from end A as λ=λ0x3, where λ0 is a positive constant. We need to find the distance of the center of mass from end B.
Step 2: Setting Up the Center of Mass Formula
The formula for the center of mass xcm of a continuous body is given by:
xcm=∫L0xdm∫L0dm
where L is the length of the rod.
Step 3: Expressing dm in Terms of dx
We know that:
dm=λdx
Substituting the expression for λ:
dm=λ0x3dx
Step 4: Substituting dm into the Center of Mass Formula
Now, substituting dm into the center of mass formula:
xcm=∫100x⋅(λ0x3)dx∫100(λ0x3)dx
Step 5: Simplifying the Integrals
The numerator becomes:
∫100x⋅(λ0x3)dx=λ0∫100x4dx
The denominator becomes:
∫100(λ0x3)dx=λ0∫100x3dx
Step 6: Calculating the Integrals
Calculating the integrals:
1. For the numerator:
∫100x4dx=(x55)100=1055=20000
2. For the denominator:
∫100x3dx=(x44)100=1044=2500
Step 7: Substituting Back into the Center of Mass Formula
Now substituting back into the center of mass formula:
xcm=λ0⋅20000λ0⋅2500=200002500=8
Step 8: Finding the Distance from End B
Since the length of the rod is 10 m, the distance of the center of mass from end B (which is at x=10) is:
Distance from B=10−xcm=10−8=2 m
Final Answer
The distance of the center of mass of the rod from end B is 2 m.
---
We have a rod AB of length 10 m, and the linear mass density λ varies with distance x from end A as λ=λ0x3, where λ0 is a positive constant. We need to find the distance of the center of mass from end B.
The formula for the center of mass xcm of a continuous body is given by:
xcm=∫L0xdm∫L0dm
where L is the length of the rod.
We know that:
dm=λdx
Substituting the expression for λ:
dm=λ0x3dx
Now, substituting dm into the center of mass formula:
xcm=∫100x⋅(λ0x3)dx∫100(λ0x3)dx
The numerator becomes:
∫100x⋅(λ0x3)dx=λ0∫100x4dx
The denominator becomes:
∫100(λ0x3)dx=λ0∫100x3dx
Calculating the integrals:
1. For the numerator:
∫100x4dx=(x55)100=1055=20000
2. For the denominator:
∫100x3dx=(x44)100=1044=2500
Now substituting back into the center of mass formula:
xcm=λ0⋅20000λ0⋅2500=200002500=8
Since the length of the rod is 10 m, the distance of the center of mass from end B (which is at x=10) is:
Distance from B=10−xcm=10−8=2 m
The distance of the center of mass of the rod from end B is 2 m.
Similar Questions
Linear mass density of a rod AB ( of length 10 m) varied with distance x from its end A as λ=λ0x3 (λ0 is poitive constant). Distance of centre of mass the rod, form end B is
View Solutionthe linear charge density of a thin metallic rod varies with the distance x from the end as λ=λ0x2(0≤x≤l) The total charge on the rod is
View SolutionA rod of length L is placed along the x-axis between x=0 and x=L. The linear mass density (mass/length) ρ of the rod varies with the distance x from the origin as ρ=a+bx. Here, a and b are constants. Find the position of centre of mass of this rod.
View SolutionThe linear mass density of a thin rod AB of length L varies from A to B as λ(x)=λ0(1+xL). Where x is the distance from A. If M is the mass of the rod then its moment of inertia about an axis passing through A and perpendicualr to the rod is :
View SolutionThe density of a thin rod of length l varies with the distance x from one end as ρ=ρ0x2l2. Find the position of centre of mass of rod.
View SolutionLinear mass density of a rod PQ of length l and mass m is varying with the distance x (from P), as λ=m2l(1+ax)
(i) Determine the value of a
(ii) Also determine the distance of c.m. from the end P.
View SolutionLinear mss density (mass/length) of a rod depends on the distanec from one end (say A) as λx=(αx+β) here α and β are constants, find the moment of inertia of this rod about an axis passing through A and perpendicular to the rod. Length of the rod is l.
View SolutionThe density of a rod of length L varies as ρ=A+Bx where x is the distance from the left end. Locate the centre of mass.

View SolutionA rod of length L is placed along the X-axis between x=0andx=L. The linear density (mass/length) ρ of the rod varies with the distance x from the origin as ρ=a+bx. a.) Find the SI units of a and b b.) Find the mass of the rod in terms of a,b, and L.
View Solution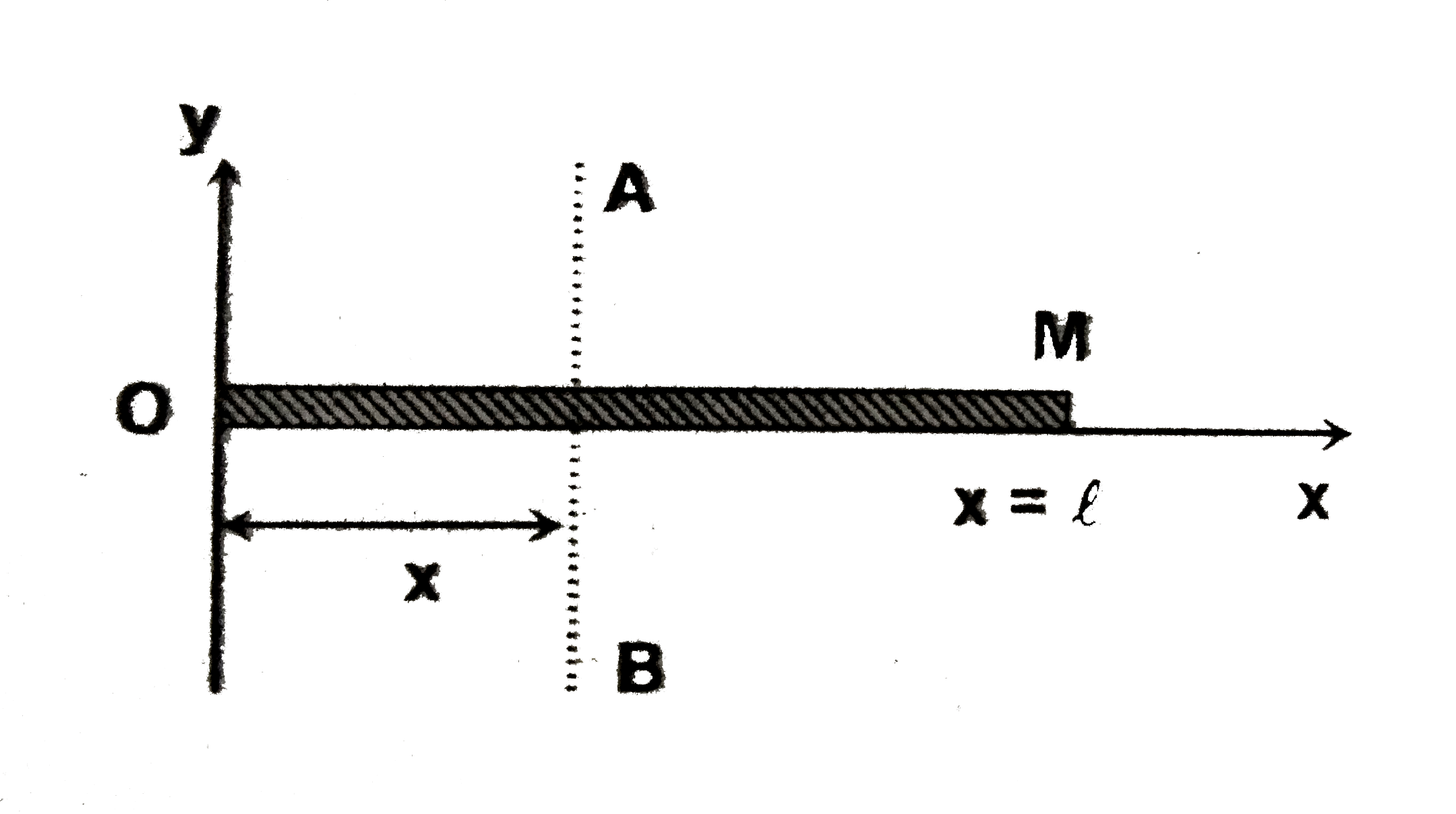
A non uniform rod OM (of length l m) is kept along x-axis and rotating about an axis AB, which is perpendicular to rod as shown in the figure. The rod has linear mass density that varies with the distance x from left end of the rod according to λ=λ0(x3L3)
Where unit of λ0 is kg/m. What is the value of x so that moment of inertia of rod about axis AB (IAB) is minimum?
View Solution
