If the ratio of diameter, lengths and Young's moduli of steel and brass wires shown in figure are 2 : 1, 2 : 1 and 2 : 1 respectively, then the corresponding ratio of increase in their lengths would be 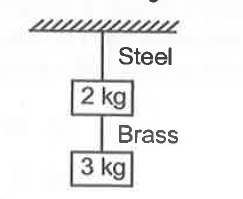
4/5
5/4
5/12
12/5
Step by step video & image solution for If the ratio of diameter, lengths and Young's moduli of steel and brass wires shown in figure are 2 : 1, 2 : 1 and 2 : 1 respectively, then the corresponding ratio of increase in their lengths would be by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
If the ratio of lengths, radii and Young's moduli of steel and brass wires in the figure are a,b and c respectively then the corresponding ratio of increase in their lengths is
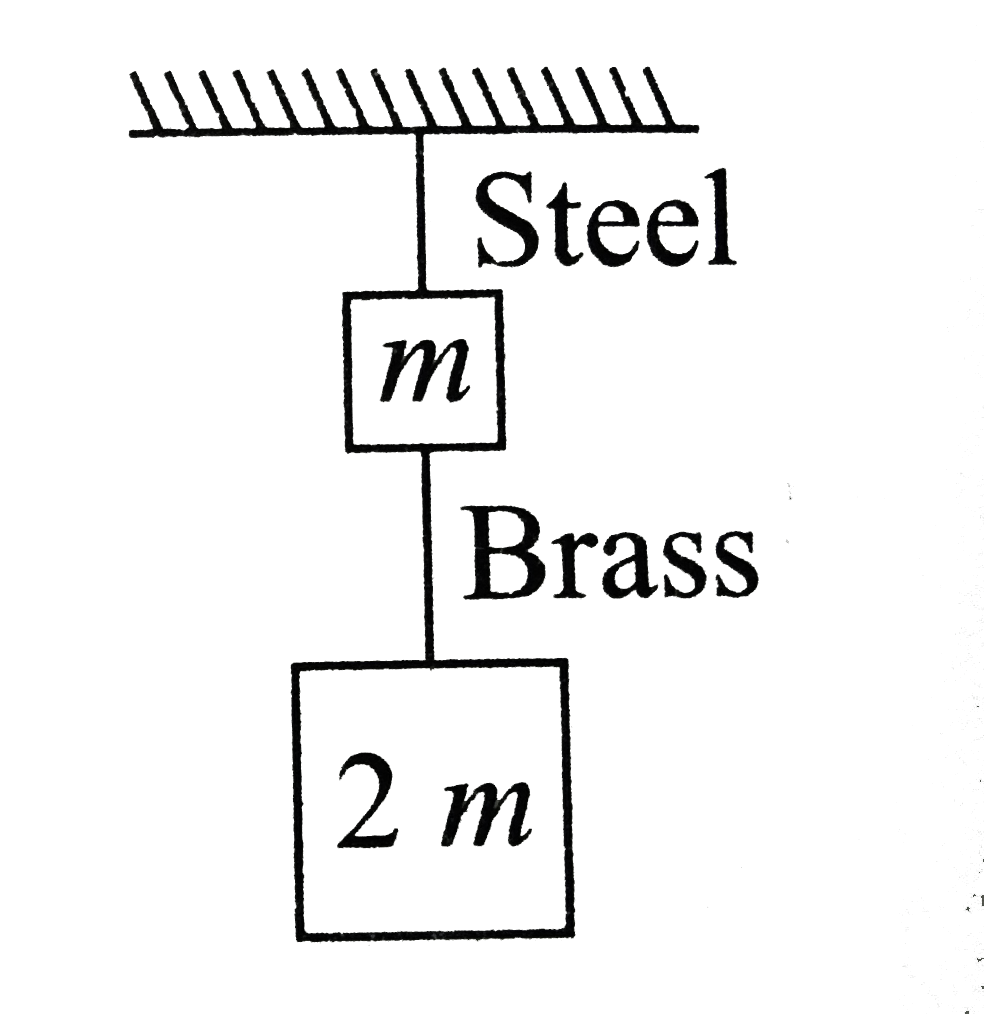
View SolutionIf the ratio of lengths, radii and Young's moduli of steel and brass wires in the figure are a,b and c respectively then the corresponding ratio of increase in their lengths is
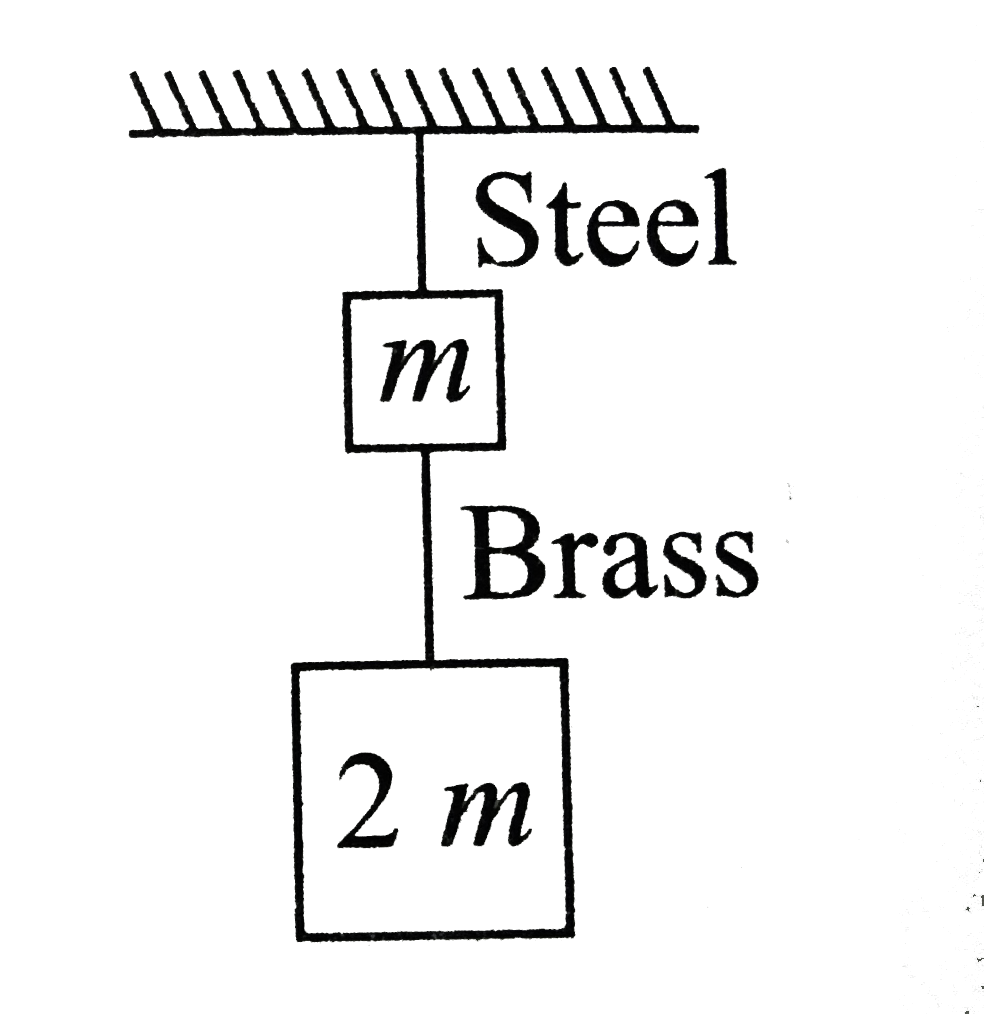
View Solution
Knowledge Check
If the ratio of diameters,lengths and Young's moduli of steel and brass wires shown in the figure are p, q and r respectively . Then the corresponding ratio of increase in their lenghts would be
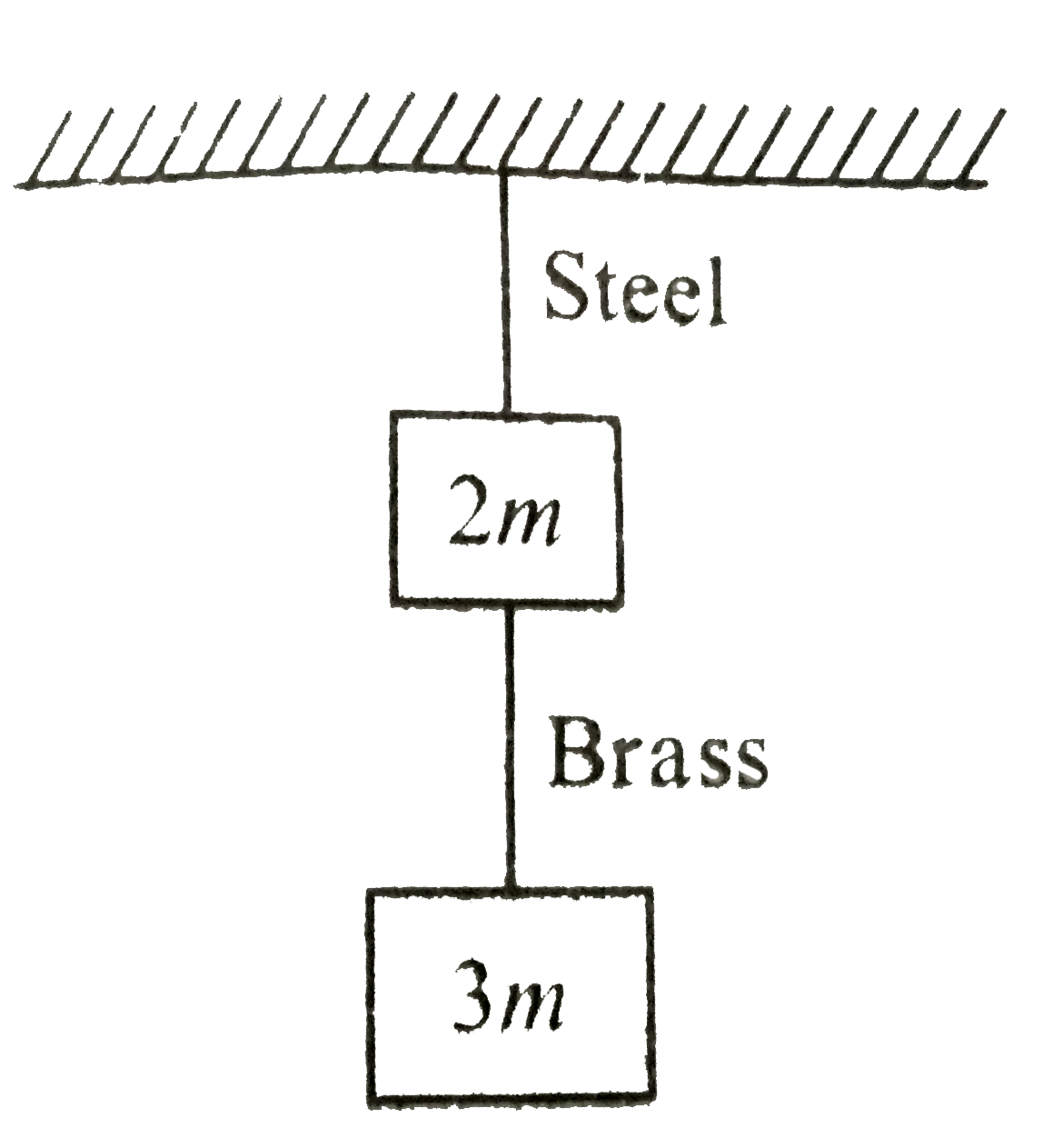 A3q5p2rB5q3p2rC3q5prD5q3pr
A3q5p2rB5q3p2rC3q5prD5q3prThe radii and Young's moduli of two uniform wires A and B are in the ratio 2:1 and 1:2 respectively. Both wires are subjected to the same longitudinal force. If the increase in length of the wire A is one percent, the percentage increase in length of the wire B is
A1.0B1.5C2.0D3.0Two wires A and B are of the same maeterial. Their lengths are in the ratio 1 : 2 and the diameters are in the ratio 2 : 1. IF they are pulled by the same force, their increases in length will be in the ratio
A2:1B1:4C1:8D8:1
If the ratio of lengths, radii and Young's moduli of steel and brass wires in the figure are a,b and c respectively then the corresponding ratio of increase in their lengths is
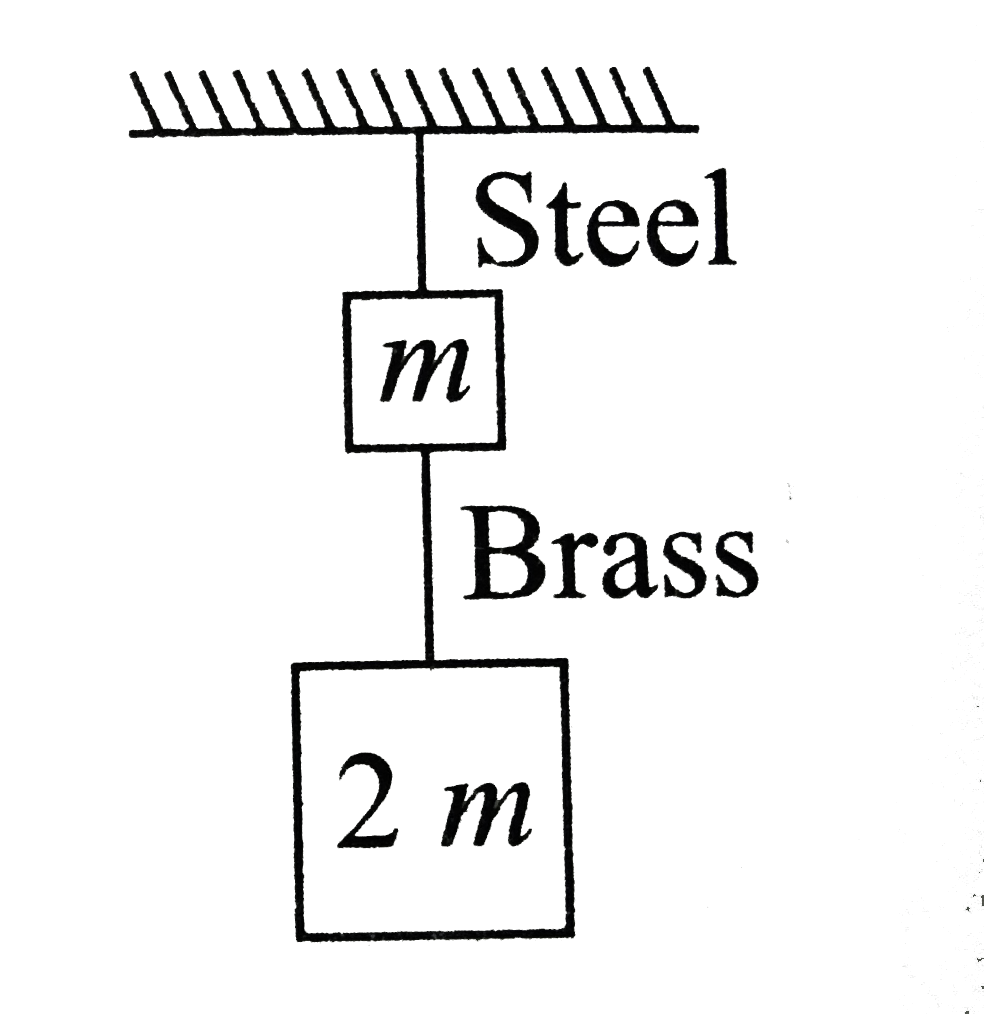
View SolutionThe time periods of two simple pendulums at a place are in the ratio 2:1. What will be the corresponding ratio of their lengths?
View SolutionTwo wires A and B are of same material. Their lengths are in the ratio 1:2 and diameters are in the ratio 2:1 when stretched by force FA and FB respectively they get equal increase in their lengths. Then the ratio FAFB should be
View SolutionTwo unifrom brass rod A and B of length l and 2l and radii 2r respectively are heated to the same temperature. The ratio of the increase in the volume of A to that of B is
View SolutionTwo wires of the same material have lengths in the ratio 1:2 and their radii are in the ratio 1:√2 If they are stretched by applying equal forces, the increase in their lengths will be in the ratio
View Solution
