ABCDE is a pentagon in which AB = AE, BC = ED and ∠ABC=∠AED.
Prove that
AC= AD
(ii) ∠BCD=∠EDC
To prove that AC=AD and ∠BCD=∠EDC in the pentagon ABCDE with the given conditions, we can follow these steps:
Step 1: Identify the Given Information
We are given:
- AB=AE
- BC=ED
- ∠ABC=∠AED
Step 2: Draw the Pentagon
Construct the pentagon ABCDE with the sides and angles as described. Label the points clearly.
Step 3: Analyze Triangles △ABC and △AED
We will compare triangles ABC and AED:
- From the given information, we have:
- AB=AE (given)
- BC=ED (given)
- ∠ABC=∠AED (given)
Step 4: Apply the Side-Angle-Side (SAS) Congruence Criterion
Since we have two sides and the included angle of triangle ABC equal to the corresponding parts of triangle AED, we can conclude:
△ABC≅△AED
Step 5: Conclude AC=AD
From the congruence of triangles ABC and AED, we can deduce:
AC=AD
Step 6: Analyze Angles ∠BCD and ∠EDC
Since △ABC≅△AED, corresponding angles are equal:
- ∠BCA=∠ADE
Step 7: Establish Relationship for ∠BCD and ∠EDC
Now, consider the angles:
- ∠BCD=∠BCA+∠ACD
- ∠EDC=∠ADE+∠ADC
Since ∠ACD=∠ADC (as AC=AD), we can write:
∠BCD=∠BCA+∠ACD
∠EDC=∠ADE+∠ADC
Step 8: Conclude ∠BCD=∠EDC
Thus, we can conclude:
∠BCD=∠EDC
Final Conclusion
We have proved that:
1. AC=AD
2. ∠BCD=∠EDC
We are given:
- AB=AE
- BC=ED
- ∠ABC=∠AED
Construct the pentagon ABCDE with the sides and angles as described. Label the points clearly.
We will compare triangles ABC and AED:
- From the given information, we have:
- AB=AE (given)
- BC=ED (given)
- ∠ABC=∠AED (given)
Since we have two sides and the included angle of triangle ABC equal to the corresponding parts of triangle AED, we can conclude:
△ABC≅△AED
From the congruence of triangles ABC and AED, we can deduce:
AC=AD
Since △ABC≅△AED, corresponding angles are equal:
- ∠BCA=∠ADE
Now, consider the angles:
- ∠BCD=∠BCA+∠ACD
- ∠EDC=∠ADE+∠ADC
∠BCD=∠BCA+∠ACD
∠EDC=∠ADE+∠ADC
Thus, we can conclude:
∠BCD=∠EDC
We have proved that:
1. AC=AD
2. ∠BCD=∠EDC
Topper's Solved these Questions
- CHAPTER REVISION (STAGE 2) BOOK - ICSECHAPTER - CHAPTER REVISION (STAGE 2) EXERCISE - CONSTRUCTIONS OF POLYGONS
19 Videos
- CHAPTER REVISION (STAGE 2) BOOK - ICSECHAPTER - CHAPTER REVISION (STAGE 2) EXERCISE - STATISTICS
2 Videos
- CHAPTER REVISION (STAGE 2) BOOK - ICSECHAPTER - CHAPTER REVISION (STAGE 2) EXERCISE - MID-POINT THEOREM
12 Videos
- AREA THEOREMSBOOK - ICSECHAPTER - AREA THEOREMSEXERCISE - Exercies 16(C )
22 Videos
- CHAPTERWISE REVISION (STAGE 1) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 1) EXERCISE - Graphical solution
10 Videos
Similar Questions
In the following figure AB = AD , AC = AE and ∠BAD=∠CAE,
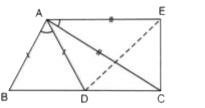
Prove that :BC = ED
View SolutionIn a quadrilateral ABCD, AB = CD and ∠B=∠C. Prove that
AC=AB
View SolutionIn the given figure, AC = AE, AB = AD and ∠BAD=∠EAC. Show that : BC = DE.

View SolutionIn a quadrilateral ABCD, AB = CD and ∠B=∠C. Prove that
AD is parallel to BC.
View SolutionWhich of the following pairs of triangles are congruent ? In each case, state the condition of congruency :
(a) In ΔABCandΔDEF, AB = DE, BC = EF and ∠B=∠E.
(b) In ΔABCandΔDEF,∠B=∠E=90∘, AC = DF and BC = EF.
(c) In ΔABCandΔQRP, AB = QR, ∠B=∠Rand∠C=∠P.
(d) In ΔABCandΔPQR, AB = PQ, AC = PR and BC = QR.
(e) In ΔABCandΔPQR, BC = QR, ∠A=90∘,∠C=∠R=40∘and∠Q=50∘.
View SolutionIn the following diagram, AD = AB and AE bisects angle A. Prove that :
(i) BE = DE (ii) ∠ABD>∠C
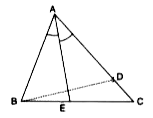
View SolutionABC is a right triangle with AB = AC.If bisector of ∠A meet BC at D then prove that BC =2 AD .
View SolutionIn parallelogram ABCD, E is the mid-point of side AB and CE bisect angle BCD. Prove that :
(i) AE=AD
(ii) DE bisect ∠ADC and
(iii) Angle DEC is a right angle.
View SolutionIn quadrilateral ABCD, AD = BC and BD = CA. Prove that :
(i) ∠ADB=∠BCA
(ii) ∠DAB=∠CBA
View SolutionIn a quadrilateral ABCD, AB= AD and CB= CD. Prove that:
AC bisects angle BAD
View Solution
