If the sides of a quadrilateral ABCD touch a circle prove that
AB+CD=BC+AD.
To prove that if the sides of a quadrilateral ABCD touch a circle, then AB+CD=BC+AD, we will use the properties of tangents drawn from external points to a circle.
1. Understanding the Setup:
Let the quadrilateral ABCD be such that a circle touches all its sides. We denote the points where the circle touches the sides as follows:
- Let the circle touch side AB at point P.
- Let the circle touch side BC at point Q.
- Let the circle touch side CD at point R.
- Let the circle touch side DA at point S.
2. Using the Tangent Property:
From the properties of tangents, we know that the lengths of tangents drawn from an external point to a circle are equal. Thus, we can write:
- AP=AS (tangents from point A)
- BP=BQ (tangents from point B)
- CR=CQ (tangents from point C)
- DR=DS (tangents from point D)
3. Expressing the Sides in Terms of Tangents:
Using the above equalities, we can express the lengths of the sides of the quadrilateral in terms of the tangent segments:
- The length of side AB can be expressed as:
AB=AP+BP
- The length of side CD can be expressed as:
CD=CR+DR
- The length of side BC can be expressed as:
BC=BQ+CQ
- The length of side AD can be expressed as:
AD=AS+DS
4. Adding the Lengths:
Now, we can add the expressions for AB and CD:
AB+CD=(AP+BP)+(CR+DR)
Similarly, we can add the expressions for BC and AD:
BC+AD=(BQ+CQ)+(AS+DS)
5. Substituting the Equalities:
Now, substituting the equalities we established earlier:
- From AP=AS and BP=BQ
- From CR=CQ and DR=DS
This gives us:
AB+CD=(AP+BQ)+(CR+DR)=(AS+BP)+(CQ+DS)
Thus, we have:
AB+CD=BC+AD
6. Conclusion:
Therefore, we conclude that:
AB+CD=BC+AD
Hence, the statement is proved.
Let the quadrilateral ABCD be such that a circle touches all its sides. We denote the points where the circle touches the sides as follows:
- Let the circle touch side AB at point P.
- Let the circle touch side BC at point Q.
- Let the circle touch side CD at point R.
- Let the circle touch side DA at point S.
From the properties of tangents, we know that the lengths of tangents drawn from an external point to a circle are equal. Thus, we can write:
- AP=AS (tangents from point A)
- BP=BQ (tangents from point B)
- CR=CQ (tangents from point C)
- DR=DS (tangents from point D)
Using the above equalities, we can express the lengths of the sides of the quadrilateral in terms of the tangent segments:
- The length of side AB can be expressed as:
AB=AP+BP
- The length of side CD can be expressed as:
CD=CR+DR
- The length of side BC can be expressed as:
BC=BQ+CQ
- The length of side AD can be expressed as:
AD=AS+DS
Now, we can add the expressions for AB and CD:
AB+CD=(AP+BP)+(CR+DR)
Similarly, we can add the expressions for BC and AD:
BC+AD=(BQ+CQ)+(AS+DS)
Now, substituting the equalities we established earlier:
- From AP=AS and BP=BQ
- From CR=CQ and DR=DS
AB+CD=(AP+BQ)+(CR+DR)=(AS+BP)+(CQ+DS)
Thus, we have:
AB+CD=BC+AD
Therefore, we conclude that:
AB+CD=BC+AD
Hence, the statement is proved.
Topper's Solved these Questions
- CIRCLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLESEXERCISE - Revision Exercise Very Short Answer Question
7 Videos
- CIRCLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLESEXERCISE - Revision Exercise Short Answer Questions
4 Videos
- CIRCLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLESEXERCISE - Problems From NCERT/exemplar
11 Videos
- ARITHMETIC PROGRESSIONBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Revision Exercise Long Answer Question
5 Videos
- CO-ORDINATE GEOMETRYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Revision Exercise Short Answer Questions
10 Videos
Similar Questions
If the sides of a quadrilateral touch a circle, prove that the sum of a pair of opposite sides is equal to the sum of the other pair.
View SolutionA quadrilateral ABCD is drawn to circumscribe a circle. Prove that AB+CD=AD+BC.
or
A circle touches all the four sides of a quadrilateral ABCD. Prove that AB+CD=BC+DA.
View SolutionIn Figure, a quadrilateral ABCD is drawn to circumscribe a circle .
Prove that
AB+CD=BC+AD

View SolutionIn Figure, a quadrilateral ABCD is drawn to circumscribe a circle .
Prove that
AB+CD=BC+AD

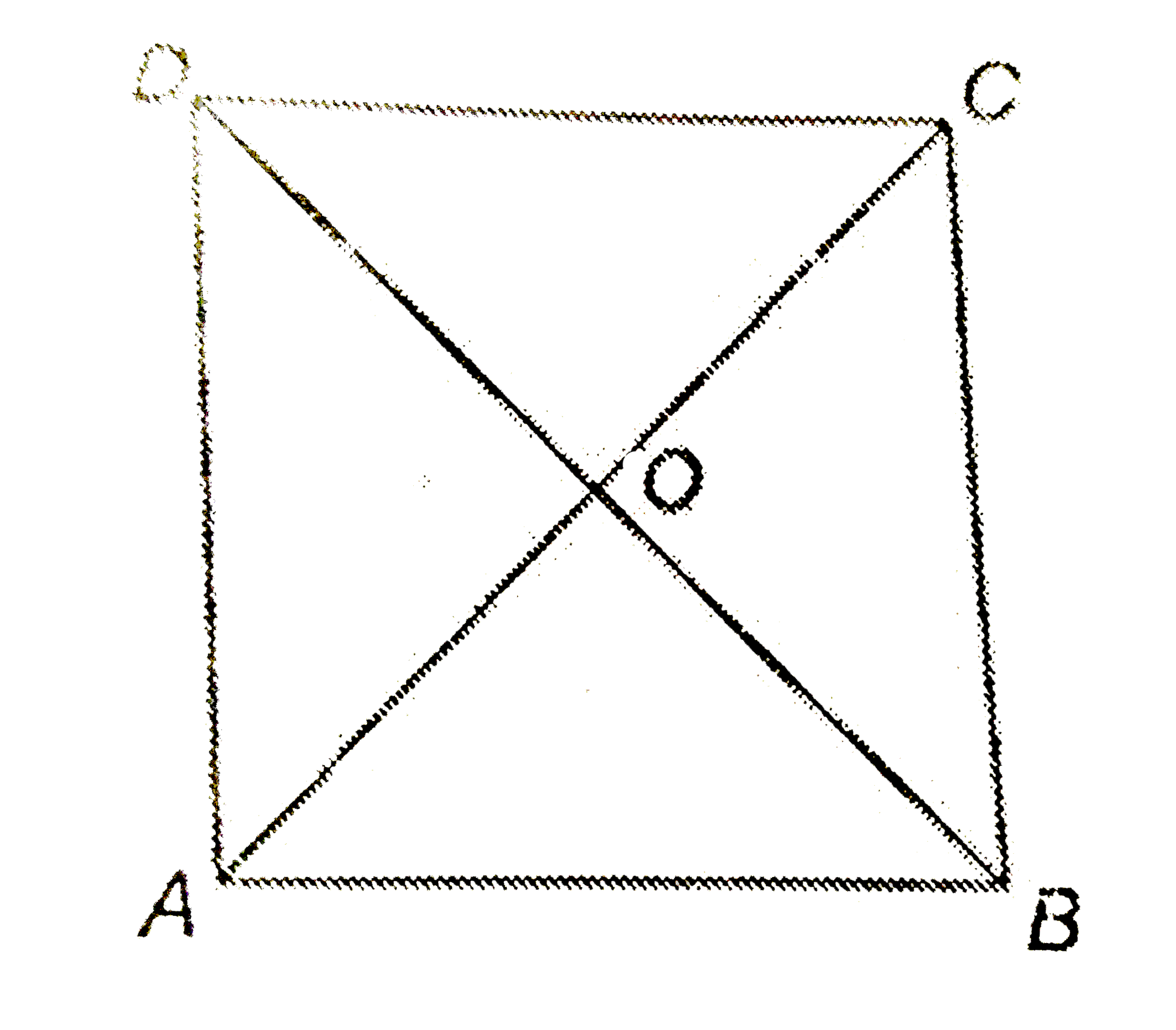
View SolutionIn the adjoining figure, the diagonals AC and BD of a quadrilateral ABCD intersect point O. Prove that :
AB+BC+CD+DA<2(AC+BD)
View SolutionIf ABCD is a cyclic quadrilateral, then prove that AC.BD=AB.CD+BC.AD
View SolutionIn Figure 3, a circle touches all the four sides of a quadrilateral ABCD whose sides are AB = 6 cm, BC = 9 cm and CD = 8 cm. Find the length of side AD.
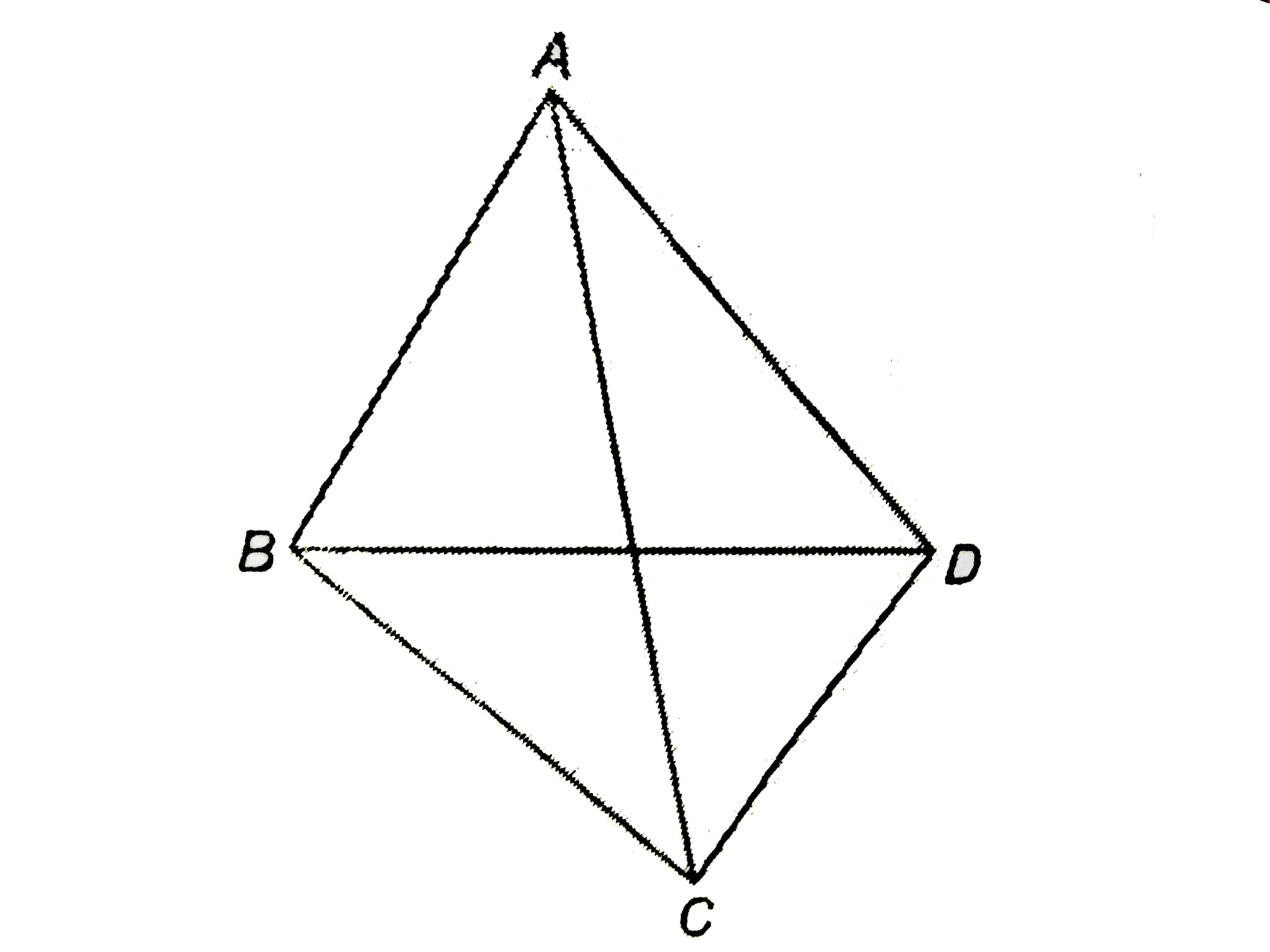
View SolutionThe given figure shows a quadrilateral ABCD. Prove that :
AB+BC+CD+DA>AC+BD
View SolutionABandCD are respectively the smallest and longest sides of a quadrilateral ABCD (see Fig. 7.50). Show that ∠A>∠C and ∠B>∠D.

View SolutionIn a quadrilateral ABCD, AB = CD and ∠B=∠C. Prove that
AC=AB
View Solution
