∆ABC ~ ∆DEF. If AB = 4 cm, BC = 3.5 cm, CA = 2.5 cm and DF = 7.5 cm, find the perimeter of ∆DEF
The correct Answer is:30 cm
To find the perimeter of triangle DEF given that triangle ABC is similar to triangle DEF, we can follow these steps:
Step 1: Calculate the perimeter of triangle ABC
The perimeter of a triangle is the sum of the lengths of its sides. For triangle ABC, we have:
- AB = 4 cm
- BC = 3.5 cm
- CA = 2.5 cm
So, the perimeter of triangle ABC (P_ABC) is:
PABC=AB+BC+CA=4+3.5+2.5
Step 2: Perform the addition
Now, let's add these values together:
PABC=4+3.5+2.5=10 cm
Step 3: Set up the proportion using the similarity of triangles
Since triangles ABC and DEF are similar, the ratios of their corresponding sides are equal. We can express this as:
PABCPDEF=CADF
Where:
- CA = 2.5 cm
- DF = 7.5 cm
Step 4: Substitute the known values into the proportion
Substituting the values we have:
10PDEF=2.57.5
Step 5: Simplify the ratio on the right side
The ratio 2.57.5 simplifies to:
2.57.5=13
Step 6: Set up the equation
Now we have:
10PDEF=13
Step 7: Cross-multiply to solve for PDEF
Cross-multiplying gives us:
10⋅3=1⋅PDEF
PDEF=30 cm
Conclusion
The perimeter of triangle DEF is 30 cm.
---
The perimeter of a triangle is the sum of the lengths of its sides. For triangle ABC, we have:
- AB = 4 cm
- BC = 3.5 cm
- CA = 2.5 cm
PABC=AB+BC+CA=4+3.5+2.5
Now, let's add these values together:
PABC=4+3.5+2.5=10 cm
Since triangles ABC and DEF are similar, the ratios of their corresponding sides are equal. We can express this as:
PABCPDEF=CADF
Where:
- CA = 2.5 cm
- DF = 7.5 cm
Substituting the values we have:
10PDEF=2.57.5
The ratio 2.57.5 simplifies to:
2.57.5=13
Now we have:
10PDEF=13
Cross-multiplying gives us:
10⋅3=1⋅PDEF
PDEF=30 cm
The perimeter of triangle DEF is 30 cm.
Topper's Solved these Questions
- MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)BOOK - ICSECHAPTER - MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)EXERCISE - EXERCISE 24 (A)
17 Videos
- MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)BOOK - ICSECHAPTER - MEASURES OF CENTRAL TENDENCY (MEAN, MEDIAN, QUARTILES AND MODE)EXERCISE - EXERCISE 24 (B)
10 Videos
- MATRICESBOOK - ICSECHAPTER - MATRICESEXERCISE - Exercise 9D
25 Videos
- MIXED PRACTICE BOOK - ICSECHAPTER - MIXED PRACTICE EXERCISE - SET B
52 Videos
Similar Questions
In parallelogram ABCD , AB = (3x- 4) cm, BC = ( y- 1) cm , CD = ( y+ 5) cm and AD = (2x+ 5) cm. find the ratio AB : BC.
Find the values of x and y.
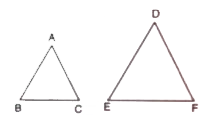
View SolutionIn the given figure, ΔABC is similar to ΔDEF,AB=(x−0.5) cm, AC=1.5xcm,DE=9cm,andDF=3xcm. Find the lengths of AB and DF.
View SolutionConstruct a ΔABC such
BC=6cm,CA=4cmandAB=3.5cm
View SolutionConstruct a ΔABC such
CA=4.8cm,CB=4.2cmandAB=4.5cm
View SolutionConstruct Δ ABC with AB = 7 cm, BC = 6.5 cm, and CA = 5.5 cm. Find the midpoint D of AB. Draw DE parallel to BC, meeting AC at E. Measure DE. Find AE : EC.
View SolutionIt is given that ΔABC~ΔEDF such that AB=5 cm, AC=7 cm, DF= 15 cm and DE = 12 cm. Find the lengths of the remaining sides of the triangles.
View SolutionConstruct a ΔABC such
AB=5cm,BC=4.6cmandAC=4.3cm
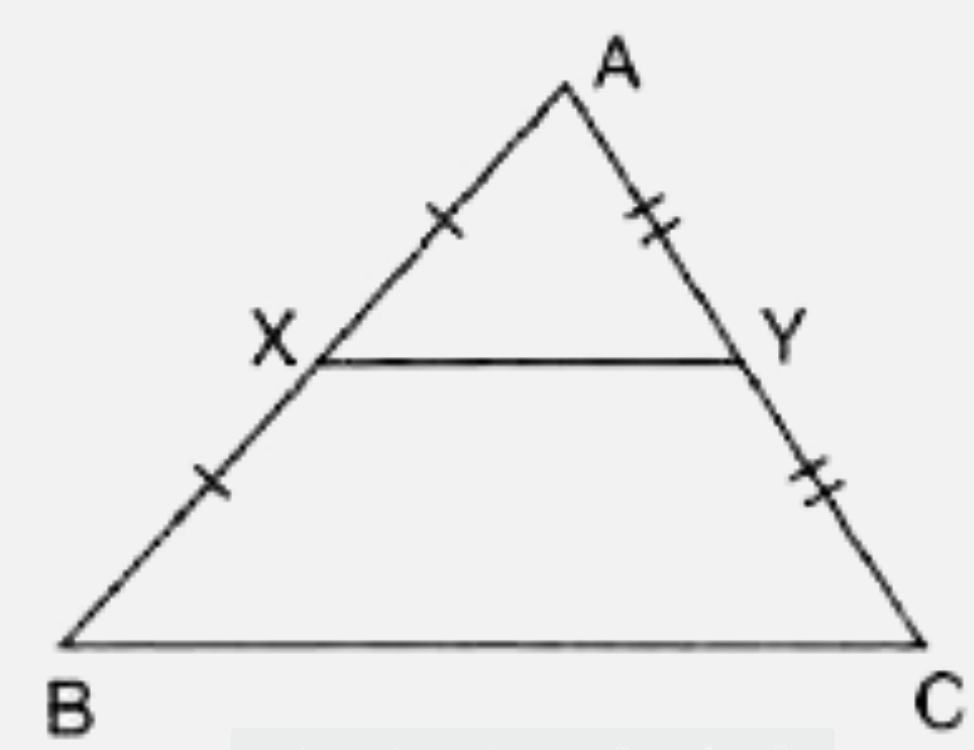
View SolutionIn the figure, given below X and Y are the mid-points of AB and AC respectively. Given that BC = 6 cm, AB = 5-4 cm and AC = 5-0 cm, calculate the perimeter of trapezium YXBC.
View SolutionConstruct Δ ABC with AB = 4.5 cm, BC = 7 cm and CA = 6 cm.
View SolutionConstruct a ΔABC in which AB = 4 cm, BC = 5.2 cm and CA = 4.5 cm.
View Solution
