A nonconducting ring of uniform mass m, radius b and uniform linear charge density is suspended as shown in figure in a gravity free space. There is uniform coaxial magnetic field , pointing up in a circular region of radius 'a' . Now if this field is switched off, then:-
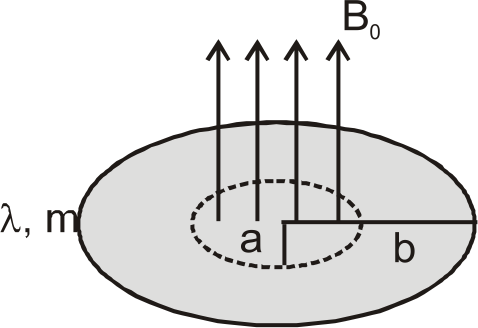
a. There will be induced electric field on periphery of ring, in anticlockwise sense when seen from above
b. Induced electric field imparts angular momentum of magnitude
c. Final angular velocity of ring will be more if time taken to switch of the field is small
d. Final angular velocity will always be independent of time taken to switch off the field
Changing magnetic field (at switching off to zero) induced electric field in such a way to restore the upwards flux, hence anticlockwise (E) as seen from above
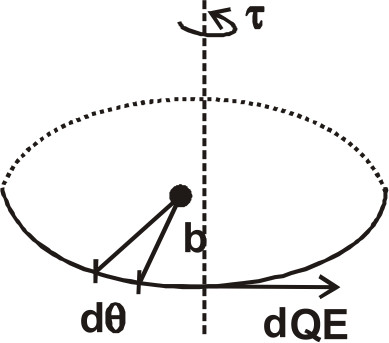
There is force on small element dQ of ring, tangentially Now this force produces torque about axis of ring to rotate in anticlockwise sense, so
So impulse or torque
(in magnitude)
It is indpendent of time taken
where I is moment of inertia
So,
Topper's Solved these Questions
Similar Questions
Figure shows a long wire having uniform charge density λ as shown in figure. Calculate electric field intensity at point P.
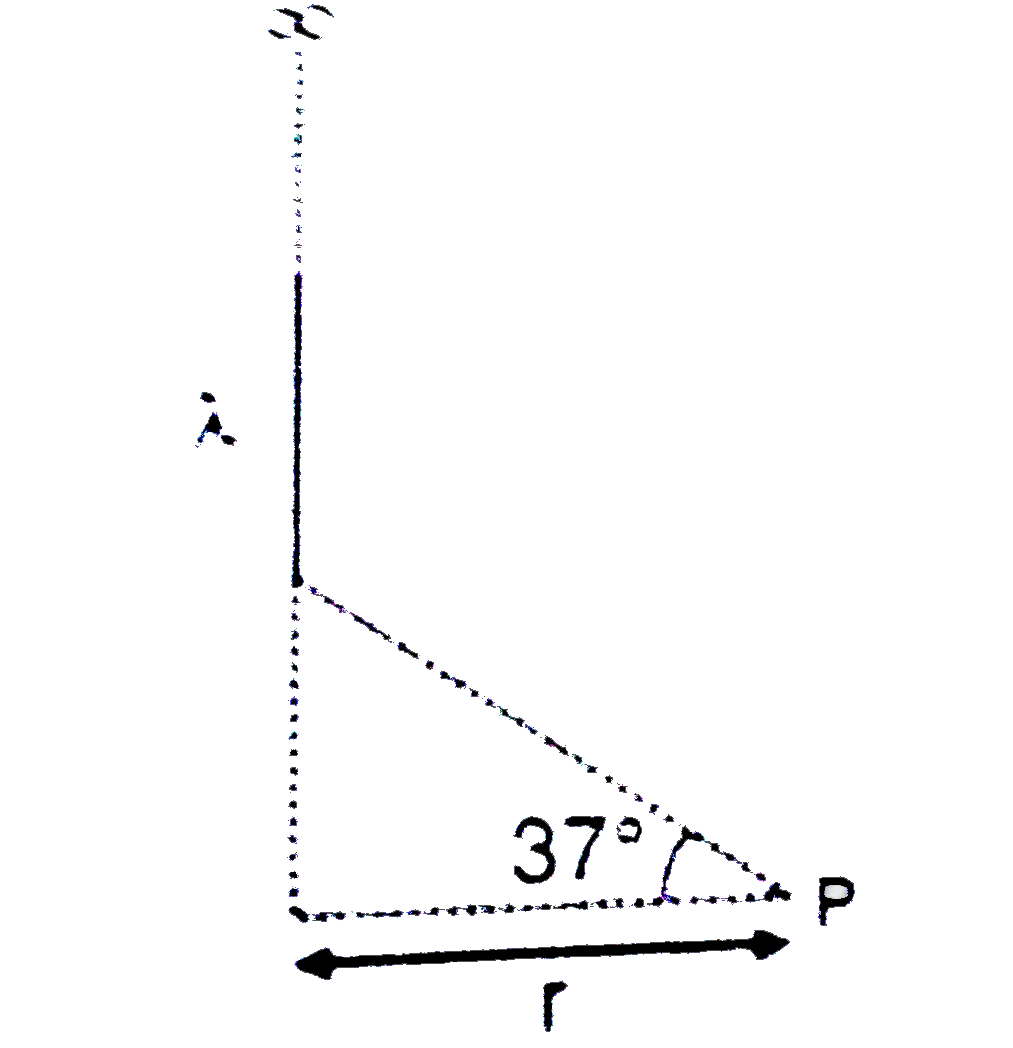 View Solution
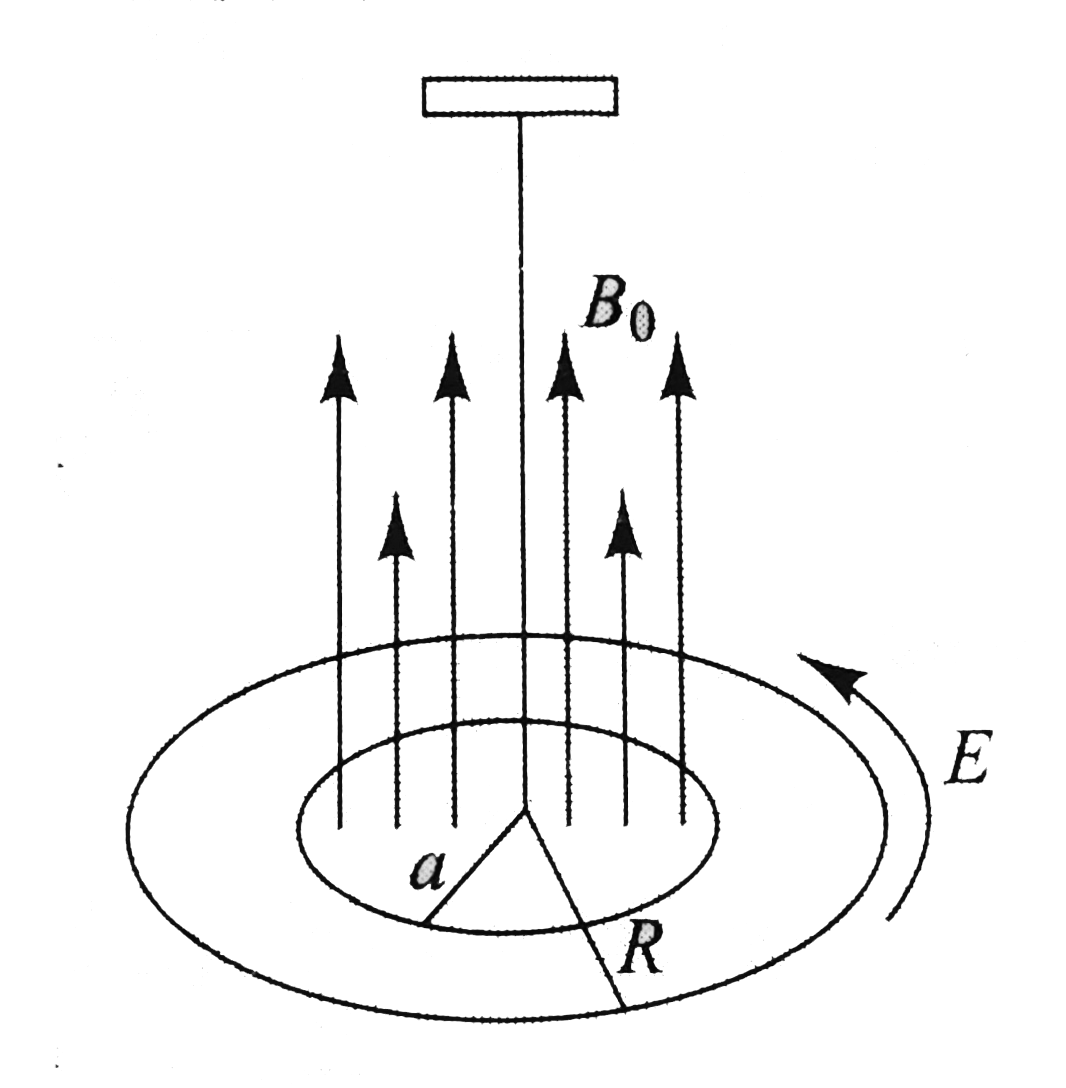
View SolutionA line charge with linear charge density λ is wound around an insulating disc of mass M and radius R, which is then suspended horizontally as shown in Fig. 3.90, so that it is free to rotate. In the central region, of radius a, there is a uniform magnetic field B0, pointing up. Now the magnetic field is switched off, which causes the disc to rotate.
Find the angular speed with which the disc starts rotating.
 View Solution
View SolutionA circular ring of radius R and uniform linear charge density +λC/m are kept in x - y plane with its centre at the origin. The electric field at a point (0,0,R√2) is
View SolutionA small conducting rod of length l, moves with a uniform velocity v in a uniform magnetic field B as shown in fig __
 View Solution
View SolutionA ring of radius R carries a non - uniform charge of linear density λ=λ0cosθ sec in the figure) Magnitude of the net dipolement of the ring is :
 View Solution
View SolutionThere is a ring of radius r having linear charge density λ and rotating with a uniform angular velocity ω. the magnetic field produced by this ring at its own centre would be
View SolutionA ring of radius R moves with a velocity v in a uniform static magnetic field B as shown in diagram. The emf between P and Q is
View SolutionA thin non-conducting ring or radius a has a linear charge density λ=λ0sinϕ. A uniform electric field E0ˆi+E0ˆj exist in the region . .Net torque acting on ring is given as :
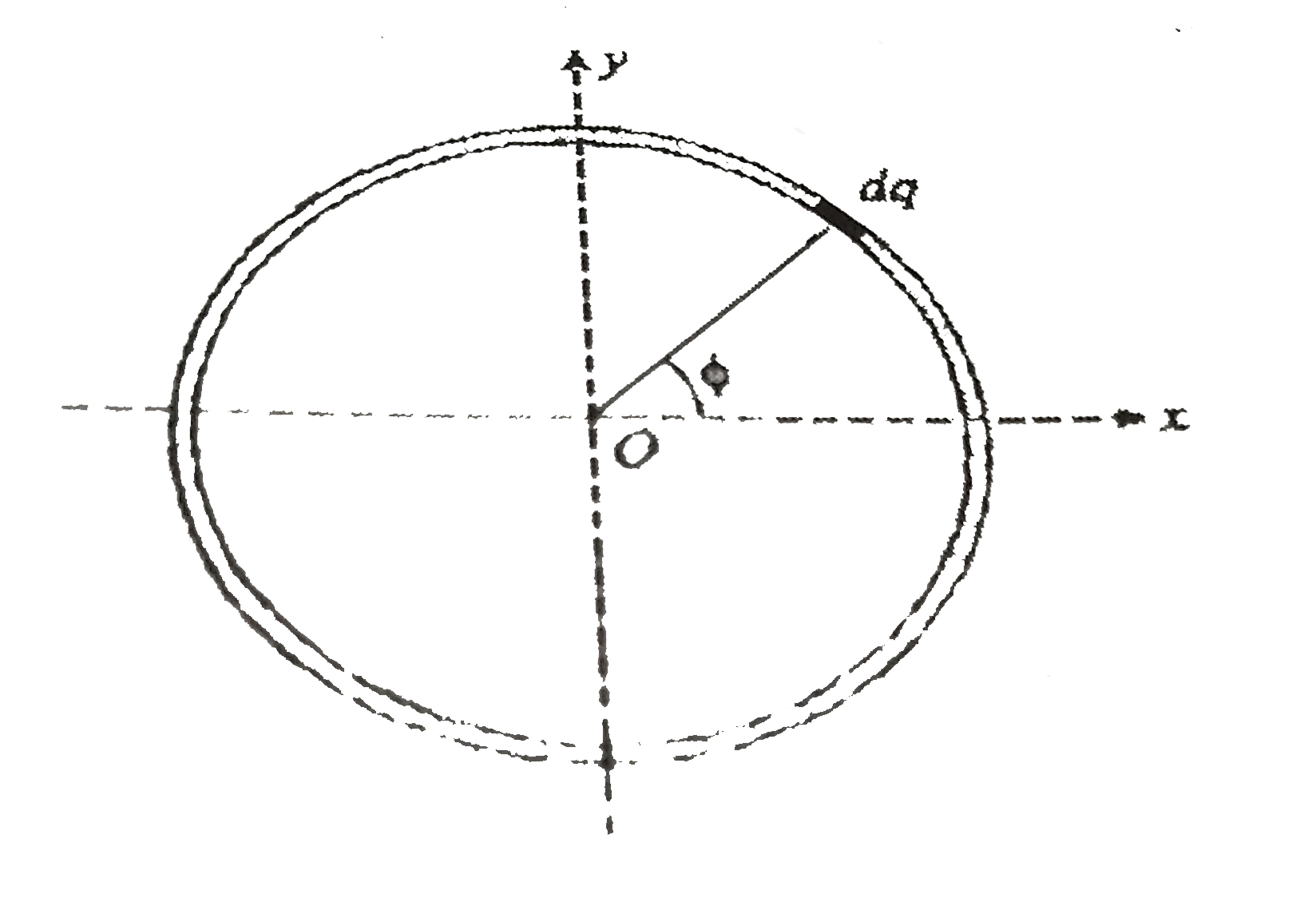 View Solution
View SolutionA thin non conducting ring of mass m, radius a carrying a charge q can rotate freely about its own axis which is vertical. At the initial moment, the ring was at rest in horizontal position and no magnetic field was present. At instant t=0, a uniform magnetic field is switched on which is vertically downward and increases with time according to the law B=B0t. Neglecting magnetism induced due to rotational motion of ring.
The magnitude of an electric field on the circumference of the ring isView SolutionA thin non conducting ring of mass m, radius a carrying a charge q can rotate freely about its own axis which is vertical. At the initial moment, the ring was at rest in horizontal position and no magnetic field was present. At instant t=0, a uniform magnetic field is switched on which is vertically downward and increases with time according to the law B=B0t. Neglecting magnetism induced due to rotational motion of ring.
Angular acceleration of ring isView Solution

