A car is moving on a straight road with a speed of 20m/s. The drive of the car put the breaks at some instant which retards the car uniformly at a rate of 2m/s2, until the car stops completely then find the time (t0) taken by the car and distance (d) travelled by car, from the instant the drive put the breaks till it stops:
t0=10 sec
d=50m
t0=5 sec
d=100m
The correct Answer is:A, D
To solve the problem, we will break it down into steps to find the time taken (t₀) and the distance (d) traveled by the car from the moment the brakes are applied until it stops.
Step 1: Identify the given values
- Initial velocity (u) = 20 m/s
- Final velocity (v) = 0 m/s (since the car stops)
- Retardation (a) = -2 m/s² (negative because it is a deceleration)
Step 2: Use the first equation of motion to find time (t₀)
The first equation of motion is:
v=u+at
Substituting the known values into the equation:
0=20+(−2)⋅t₀
This simplifies to:
0=20−2t₀
2t₀=20
t₀=202=10 seconds
Step 3: Use the second equation of motion to find distance (d)
The second equation of motion is:
s=ut+12at2
Substituting the known values:
d=20⋅10+12⋅(−2)⋅(10)2
Calculating each term:
1. 20⋅10=200
2. 12⋅(−2)⋅100=−100
Now, combine these results:
d=200−100=100 meters
Final Results
- Time taken (t₀) = 10 seconds
- Distance traveled (d) = 100 meters
- Initial velocity (u) = 20 m/s
- Final velocity (v) = 0 m/s (since the car stops)
- Retardation (a) = -2 m/s² (negative because it is a deceleration)
The first equation of motion is:
v=u+at
0=20+(−2)⋅t₀
0=20−2t₀
2t₀=20
t₀=202=10 seconds
The second equation of motion is:
s=ut+12at2
d=20⋅10+12⋅(−2)⋅(10)2
1. 20⋅10=200
2. 12⋅(−2)⋅100=−100
d=200−100=100 meters
- Time taken (t₀) = 10 seconds
- Distance traveled (d) = 100 meters
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS SEC - 220videos
TEST PAPERS
- BOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS106videos
TEST PAPERS
- BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems13videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - PHYSICS130videos
TEST SERIES
Similar Questions
Knowledge Check
- Question 1 - Select One
A car moving along a straight road with speed of 144kmh−1 is brought to a stop within a distance of 200 m. How long does it take for the car to stop ?
A5 sB10 sC15 sD20 s
A car is moving in a straight line with speed 18 km h−1 . It is stopped in 5 s by applying the brakes. Find the retardation
View SolutionA car is traveling in a straight line along a highway at a speed of 20m/s. The driver steps on the gas pedal, and 3 seconds later, the car's speed is 32m/s. Find its average acceleration.
View SolutionA car is moving along a straight horizontal road with a speed v0 . If the coefficient of friction between the tyres and the road is μ , the shortest distance in which the car can be stopped is
View SolutionA car moving along a straight highway with speed of 126kmh−1 is brought to a stop within a distance of 200m. What is the retardation of the car (assumed uniform) and how long does it take for the car to stop ?
View SolutionA car is moving in a straight line with speed 18 km h−1 . It is stopped in 5 s by applying the brakes. Find the speed of car in ms−1 .
View SolutionA car is moving on a straight road. The velocity of the car varies with time as shown in the figure. Initially (at t=0), the car was at x=0, where, x is the position of the car at any time 't'.
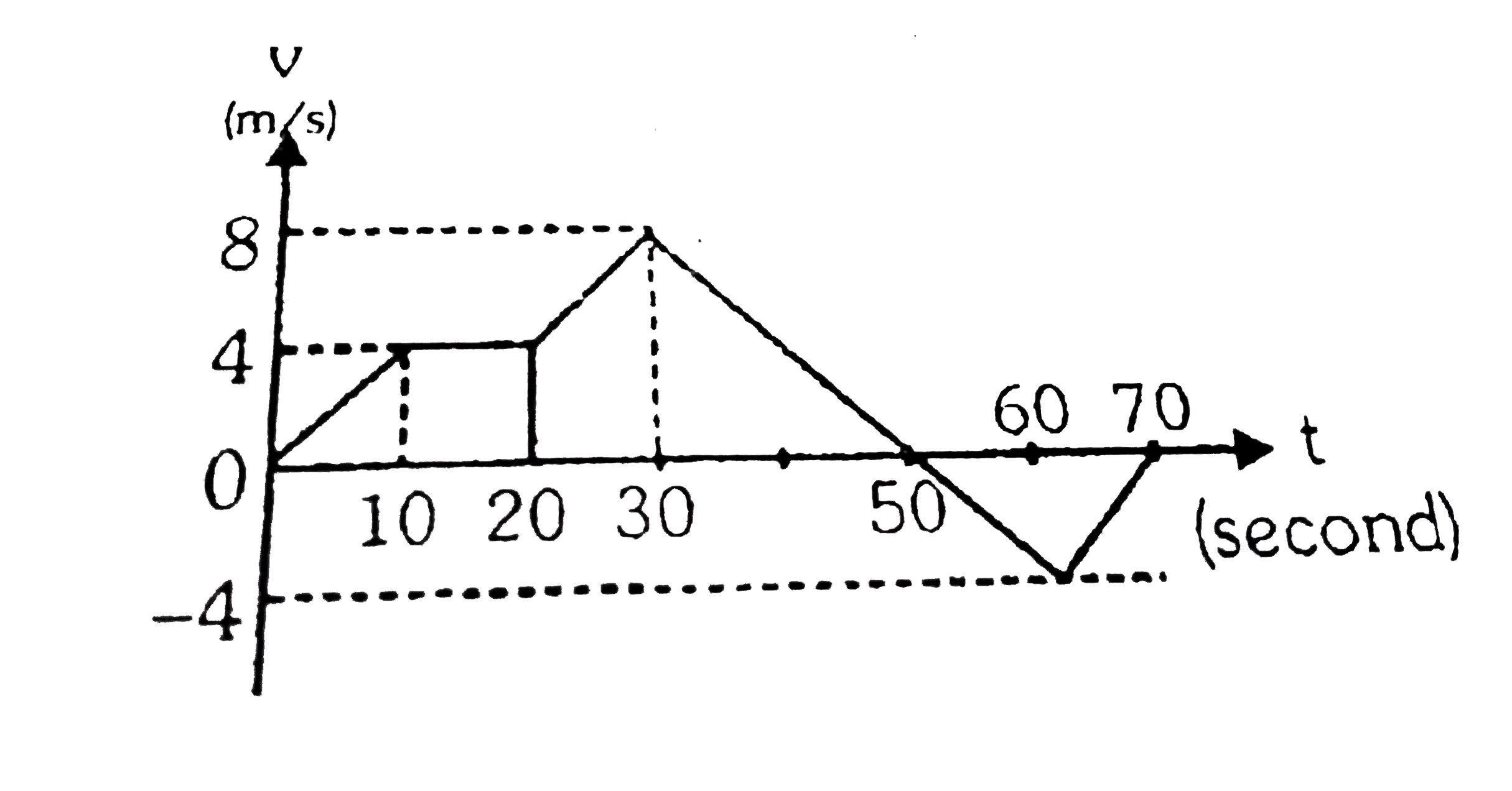
The time interval during which the car is retarding can be :-View SolutionA car is moving in a straight line with speed 18 km h−1 . It is stopped in 5 s by applying the brakes. Find the speed of car after 2 s of applying the brakes.
View Solution

