A uniform metal chain is placed on a rough table such that the one end of chain hangs down over the edge of the table, when one-third of its length hang over the edge, the chain starts sliding. Then the coefficient of static friction is
(a)3/4
(b)1/4
(c)2/3
(d)1/2
The correct Answer is:D
To solve the problem step by step, we will analyze the forces acting on the chain and apply the principles of static friction.
1. Define the Length of the Chain:
Let the total length of the chain be L. When one-third of the chain hangs over the edge of the table, the length of the chain hanging down is:
Lh=L3
The length of the chain on the table is:
Lt=L−Lh=L−L3=2L3
2. Calculate the Weight of the Hanging Portion:
The weight of the hanging portion of the chain can be calculated using the mass per unit length. Let m be the mass of the entire chain. The mass per unit length λ is:
λ=mL
Therefore, the weight of the hanging portion is:
Wh=λ⋅Lh=mL⋅L3=m3
3. Calculate the Normal Force:
The normal force N acting on the chain from the table is equal to the weight of the portion of the chain that is on the table:
Wt=λ⋅Lt=mL⋅2L3=2m3
Thus, the normal force N is:
N=Wt=2m3
4. Apply Static Friction Condition:
The maximum static friction force Fs that can act on the chain is given by:
Fs=μs⋅N=μs⋅2m3
where μs is the coefficient of static friction.
5. Set Up the Equation for Sliding:
The chain starts sliding when the weight of the hanging portion equals the maximum static friction force:
Wh=Fs
Substituting the values we have:
m3=μs⋅2m3
6. Solve for the Coefficient of Static Friction:
We can cancel m from both sides (assuming m≠0):
13=μs⋅23
Multiplying both sides by 3:
1=2μs
Dividing both sides by 2:
μs=12
Final Answer:
The coefficient of static friction μs is 12.
---
Let the total length of the chain be L. When one-third of the chain hangs over the edge of the table, the length of the chain hanging down is:
Lh=L3
The length of the chain on the table is:
Lt=L−Lh=L−L3=2L3
The weight of the hanging portion of the chain can be calculated using the mass per unit length. Let m be the mass of the entire chain. The mass per unit length λ is:
λ=mL
Therefore, the weight of the hanging portion is:
Wh=λ⋅Lh=mL⋅L3=m3
The normal force N acting on the chain from the table is equal to the weight of the portion of the chain that is on the table:
Wt=λ⋅Lt=mL⋅2L3=2m3
Thus, the normal force N is:
N=Wt=2m3
The maximum static friction force Fs that can act on the chain is given by:
Fs=μs⋅N=μs⋅2m3
where μs is the coefficient of static friction.
The chain starts sliding when the weight of the hanging portion equals the maximum static friction force:
Wh=Fs
Substituting the values we have:
m3=μs⋅2m3
We can cancel m from both sides (assuming m≠0):
13=μs⋅23
Multiplying both sides by 3:
1=2μs
Dividing both sides by 2:
μs=12
The coefficient of static friction μs is 12.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - DYNAMICS OF A PARTICLEEXERCISE - LEVEL 249videos
DYNAMICS OF A PARTICLE
- BOOK - VMC MODULES ENGLISHCHAPTER - DYNAMICS OF A PARTICLEEXERCISE - LEVEL 2 ( NUMERICAL TYPE FOR JEE MAIN )16videos
DYNAMICS OF A PARTICLE
- BOOK - VMC MODULES ENGLISHCHAPTER - DYNAMICS OF A PARTICLEEXERCISE - LEVEL 0 (LONG ANSWER TYPE)4videos
DYNAMICS OF A PARTICLE
- BOOK - VMC MODULES ENGLISHCHAPTER - DC CIRCUITEXERCISE - JEE ADVANCED ARCHIVE68videos
DC CIRCUIT
- BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - IN-CHAPTER EXERCISE-G10videos
ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENT
Similar Questions
A uniform chain of length L lies on a smooth horizontal table with its length perpendicular to the edge of the table and a small portion of the chain is hanging over the edge. The chain starts sliding due to the weight of the hanging part
View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

If the chain is limiting equlibrium, what is the coefficient of friction for the contact between table and chain?View Solution
Knowledge Check
- Question 1 - Select One
A uniform chain of length 2m is kept on a table such that a length of 60cm hangs freely from the edge of the table . The total mass of the chain is 4kg What is the work done in pulling the entire the chain the on the table ?
A12.9JB6.3 JC3.6 JD2.0 J
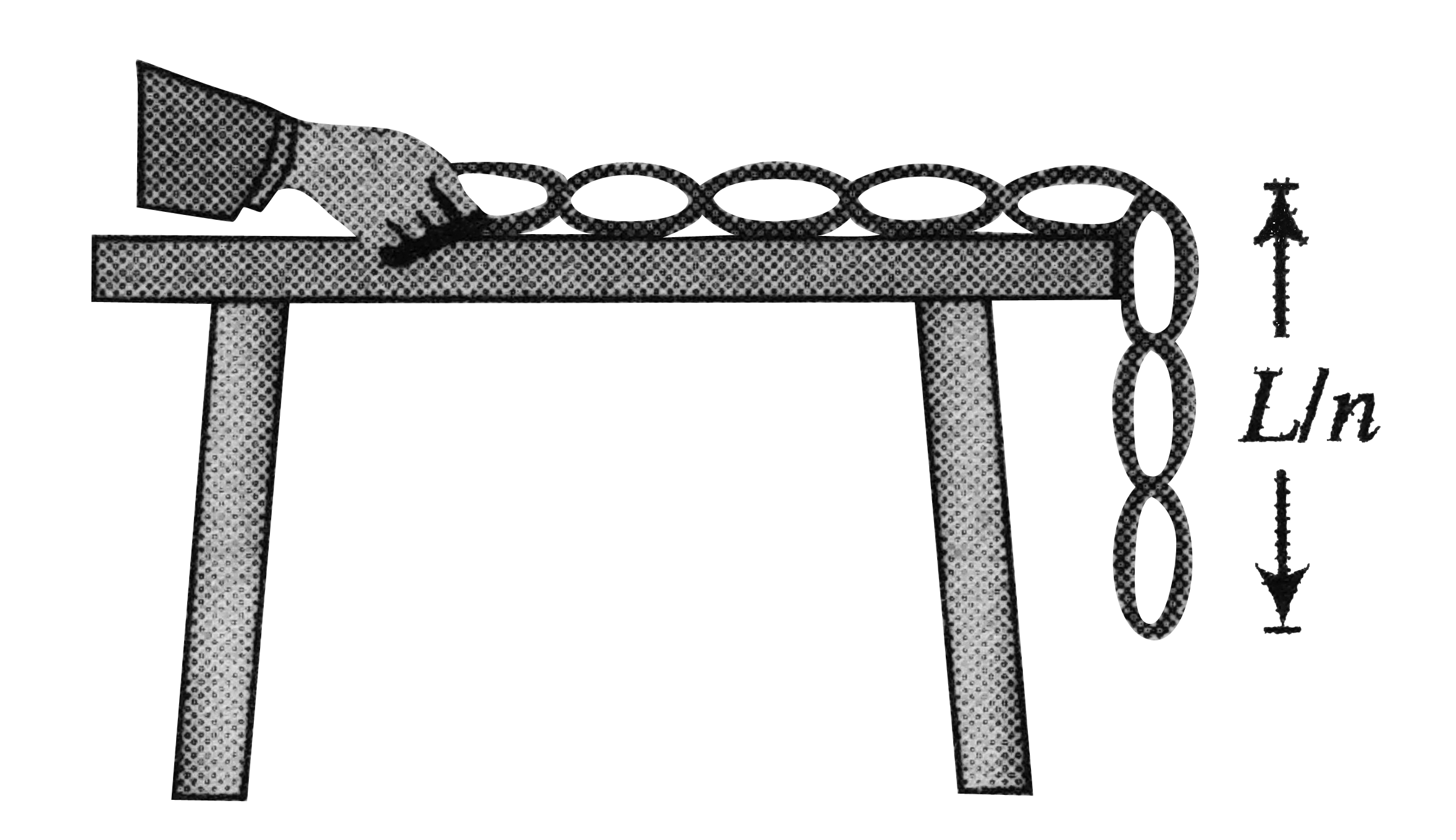
A chain is lying on a rough table with a fraction 1/n of its length hanging down from the edge of the table. if it is just on the point of sliding down from the table, then the coefficient of friction between the table and the chain is -
View SolutionA uniform chain of length l is placed on rough table with length l/n (where n>1) , hanging over the edge. If the chain just begins to slide off the table by itself from this position, the coefficient of friction between the chain and the table is
View SolutionA uniform rope of length l and mass m lies on a smooth horizontal table with its length perpendicular to the edge of the table and a small part of the rope having over the edge. The rope starts sliding from rest under the weight of the over hanging end. The velocity of the rope when the length of the hanging part is . x
View SolutionA rope lies on a table such that a part of it hangs down the table, when the length of hanging part is 1/3 of entire length the rope just begins to slide. The coefficient of friction between the rope and the table is:-
View SolutionA chain of length L and mass M is held on a frictionless table with (1/n)th of its length hanging over the edge. When the chain is released, find the velocity of chain while leaving the table.
 View Solution
View SolutionA uniform chain of length 4m is kept on a table such that a length of 120 cm hangs freely from the edge of the table. The total mass of the chain is 4 kg What is the work done in pulling the entire chain on the table ?
View SolutionA uniform chain of mass 4 kg and length 2 m is kept on table such that 3/10th of the chain hanges freely from the edge of the table. How much work has to be done in pulling the entire chain on the table?
View Solution

