Two identical long, solid cylinders are used to conduct heat from temp T1 to Temp T2. Originally the cylinders are connected in series and the rate of heat transfer is H. If the cylinders are connected in parallel then the rate of heat transer would be:
H/4
2H
4H
8H
The correct Answer is:C
To solve the problem, we need to analyze the heat transfer through the two identical long solid cylinders when they are connected in series and then in parallel.
1. Understanding Heat Transfer in Series:
- When the two cylinders are connected in series, the total thermal resistance Rseries is the sum of the individual resistances:
Rseries=R1+R2=R+R=2R
- The rate of heat transfer H through the cylinders can be expressed using the formula:
H=T1−T2Rseries
- Substituting Rseries:
H=T1−T22R
2. Understanding Heat Transfer in Parallel:
- When the two cylinders are connected in parallel, the total thermal resistance Rparallel can be calculated using the formula for resistances in parallel:
1Rparallel=1R1+1R2=1R+1R=2R
- Therefore, the equivalent resistance in parallel is:
Rparallel=R2
- The rate of heat transfer H′ through the cylinders in parallel is given by:
H′=T1−T2Rparallel
- Substituting Rparallel:
H′=T1−T2R2=2(T1−T2)R
3. Relating H′ to H:
- From our earlier expression for H:
H=T1−T22R
- We can express H′ in terms of H:
H′=4H
Conclusion:
Thus, when the two identical long solid cylinders are connected in parallel, the rate of heat transfer increases to 4H.
- When the two cylinders are connected in series, the total thermal resistance Rseries is the sum of the individual resistances:
Rseries=R1+R2=R+R=2R
- The rate of heat transfer H through the cylinders can be expressed using the formula:
H=T1−T2Rseries
- Substituting Rseries:
H=T1−T22R
- When the two cylinders are connected in parallel, the total thermal resistance Rparallel can be calculated using the formula for resistances in parallel:
1Rparallel=1R1+1R2=1R+1R=2R
- Therefore, the equivalent resistance in parallel is:
Rparallel=R2
- The rate of heat transfer H′ through the cylinders in parallel is given by:
H′=T1−T2Rparallel
- Substituting Rparallel:
H′=T1−T2R2=2(T1−T2)R
- From our earlier expression for H:
H=T1−T22R
- We can express H′ in terms of H:
H′=4H
Thus, when the two identical long solid cylinders are connected in parallel, the rate of heat transfer increases to 4H.
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.62
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.63
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.60
8 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
When two identical batteries of internal resistance 1Ω each are connected in series across a resistor R, the rate of heat produced in R is J1. When the same batteries are connected in parallel across R, the rate is J2=2.25J1 then the value of R in Ω is
View SolutionTwo identical rods are connected between two containers. One of them is at 100∘C containing water and another is at 0∘C containing ice. If rods are connected in parallel then the rate of melting of ice is q1g/s . If they are connected in series then teh rate is q2g/s . The ratio q2/q1 is
View Solution
Knowledge Check
A monatomic gas on n molecules is heated from temperature T1 to T2 under two different conditions (i) constant volume, (ii) constant pressure. The change of internal energy of the gas
Amore of (i)Bmore of (ii)Csame in both casesDindependent of number of moles
A long resistance wire is divided into 2n parts. Then n parts are connected in series and the other n parts in parallel separately. Both combinations are connected to identical supplies. Then the ratio of heat produced in series to parallel combinations will be -
View SolutionTwo conducting wires of the same material and of equal length and equal diameters are first connected in series and then in parallel in an electric circuit. The ratio of the heat produced in series and parallel combinations would be :
View SolutionA cylindrical rod having temperature T1 and T2 at its ends. The rate of flow of heat is Q1cal/sec. If all the linear dimensions are doubled keeping temperature constant, then rate of flow of heat Q2 will be
View SolutionTwo vertical cylinders are connected by a small tube at the bottom. It contains a gas at constant temperature . Initially the pistons are located at the same height. The diameters of the two cylinders are different . Outside the cylinder the space is vaccum . Gravitational acceleration is h,h0=20cm,m1=2kgandm2=1kg. The pistons are initially in equilibrium. If the masses of the piston are interchanged find the separation between the two pistons when they are again in equilibrium. Assume constant temperature .
_E01_299_Q01.png)
View SolutionTwo long ,thin,solid cylinders are identical in size, by they are made of different substances with two different thermal conductivities. The two cylinders are connected in series between a reservoir at temperature Thot and a reservoir at temperature Tcold.The temperature at the boundary between the two cylinders is Tb. One can conclude that:-
View SolutionA thermometer uses density of water as thermometric property. Actual reading in thermometer is height of water (h) which inversely proportional to the density of water (d). In certain temp range is symmetrical ablout maximum.
Two identical bodies (of same mass and specific heat) at different temp T1 and T2 show the same reading of height h1=h2 in the thermometer. The bodies are brought in contact and allowed to reach the equilibrium. The thermometric reading height of water for final equilibrium state hf satisfies
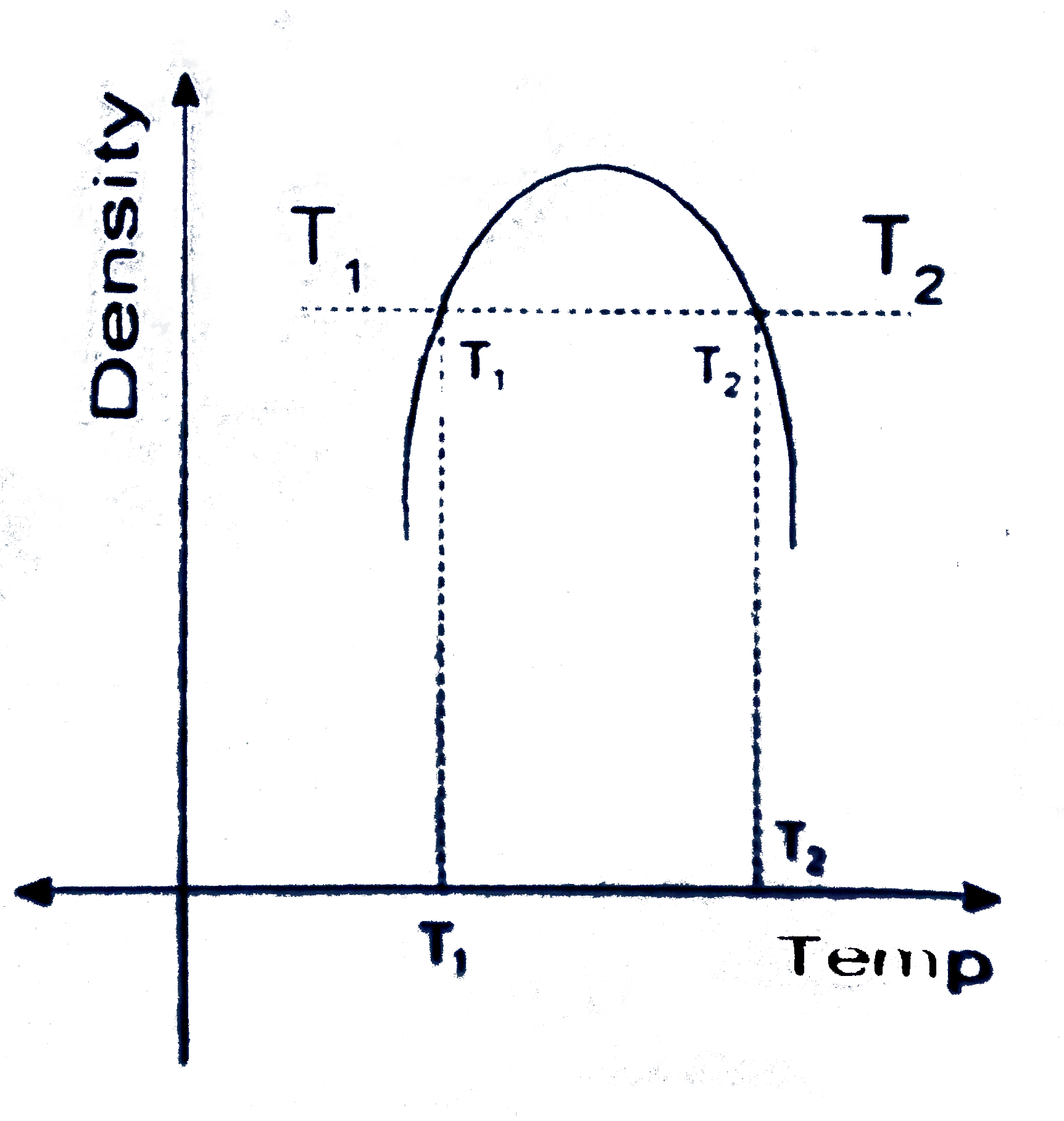
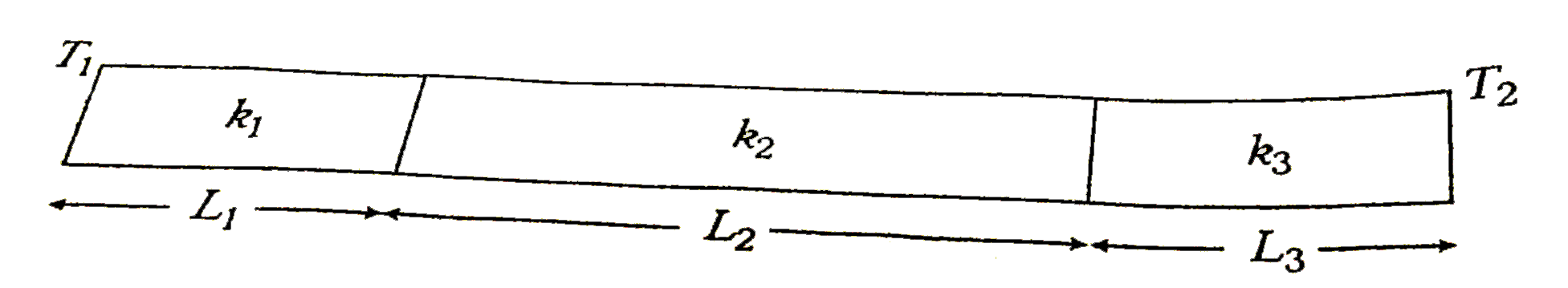
View SolutionThree rods of same cross-section but different length and conductivity are joined in series . If the temperature of the two extreme ends are T1andT2(T1>T2) find the rate of heat transfer H.
View Solution