Doubtnut Promotions Sticky

If the net electric field flux passing through a closed surface is zero, then the electric field at the surface will be

A

0

B

same at all places

C

depends upon the location of points

D

infinite

Text Solution
Verified by Experts

The correct Answer is:C

depends upon the location of points

Doubtnut Promotions Banner
|
Updated on:21/07/2023
Class 11PHYSICSELECTROSTATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    If the net electric flux through a closed surface is zero, then we can infer

    Ano net charge is enclosed by the surface
    Buniform electric field exists within the surface
    Celectric potential varies from point to point inside the surface
    Dcharge is present inside the surface
  • Question 2 - Select One

    If the flux of the electric field through a closed surface is zero ,
    (i) the electric field must be zero everywhere on the surface
    (ii) the electric field may be zero everywhere on the surface
    (iii) the charge inside the surface must be zero
    (iv) the charge in the vicinity of the surface must be zero

    A(i),(ii)
    B(ii),(iii)
    C(ii),(iv)
    D(i),(iii)
  • Question 3 - Select One

    Three point charges are placed as shown in the figure. S is a gaussian surface ϕ is the net flux passing through surface A and E be the electric field at point P on the surface, then
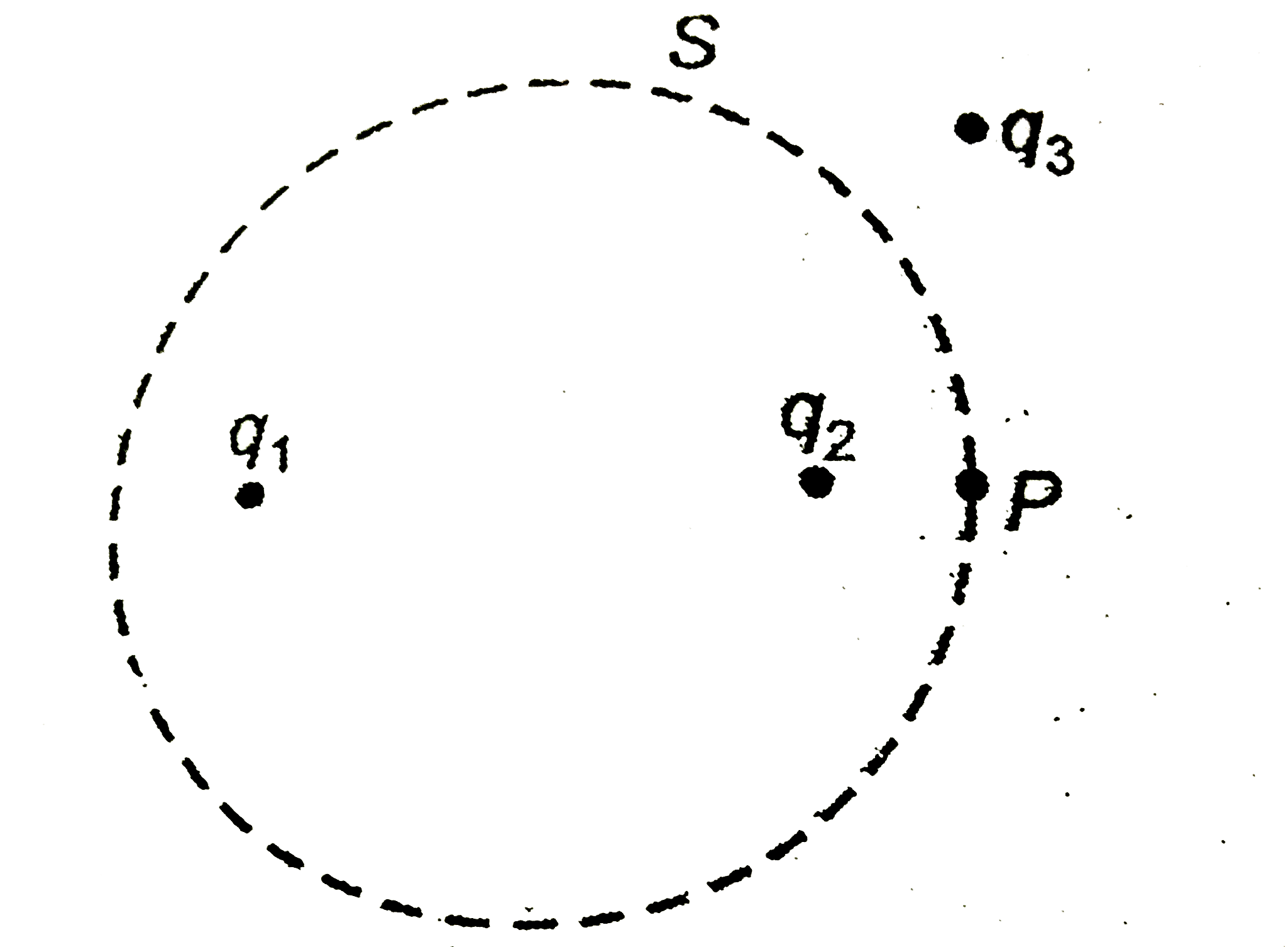    ocr_image

    AFlux ϕ is due to q1 and q3 only
    BFlux ϕ is due to q1 and q2
    CElectric field at point P is due to q1 and q2 only
    DFlux ϕ is due to q3 only