Two rods of same length and areas of cross section A1 and A2 have their ends at same temperature. If K1 and K2 are their thermal conductivities, C1 and C2 their specific heats and ρ1 and ρ2 are their densities, then the condition that rate of flow of heat is same in both the rods is
A1/A2=K1/K2
A1/A2=K1C1ρ1/K2C2ρ2
A1/A2=K2C1ρ1/K1C2ρ2
A1/A2=K2/K1
The correct Answer is:D
To solve the problem, we need to establish the condition under which the rate of heat flow through two rods of different cross-sectional areas and thermal conductivities is the same.
1. Understanding Heat Flow:
The rate of heat flow (dq/dt) through a rod is given by the formula:
dqdt=K⋅A⋅dθdx
where K is the thermal conductivity, A is the area of cross-section, and dθdx is the temperature gradient.
2. Applying the Formula to Both Rods:
For the first rod with thermal conductivity K1 and cross-sectional area A1:
dqdt=K1⋅A1⋅dθdx
For the second rod with thermal conductivity K2 and cross-sectional area A2:
dqdt=K2⋅A2⋅dθdx
3. Setting the Heat Flow Equal:
Since the rate of heat flow is the same in both rods, we can equate the two expressions:
K1⋅A1⋅dθdx=K2⋅A2⋅dθdx
4. Canceling the Common Terms:
Since both rods are at the same temperature difference and have the same length, dθdx is the same for both rods. We can cancel dθdx from both sides:
K1⋅A1=K2⋅A2
5. Rearranging the Equation:
Rearranging gives us:
A1A2=K2K1
Conclusion:
The condition that the rate of flow of heat is the same in both rods is:
A1A2=K2K1
The rate of heat flow (dq/dt) through a rod is given by the formula:
dqdt=K⋅A⋅dθdx
where K is the thermal conductivity, A is the area of cross-section, and dθdx is the temperature gradient.
For the first rod with thermal conductivity K1 and cross-sectional area A1:
dqdt=K1⋅A1⋅dθdx
For the second rod with thermal conductivity K2 and cross-sectional area A2:
dqdt=K2⋅A2⋅dθdx
Since the rate of heat flow is the same in both rods, we can equate the two expressions:
K1⋅A1⋅dθdx=K2⋅A2⋅dθdx
Since both rods are at the same temperature difference and have the same length, dθdx is the same for both rods. We can cancel dθdx from both sides:
K1⋅A1=K2⋅A2
Rearranging gives us:
A1A2=K2K1
The condition that the rate of flow of heat is the same in both rods is:
A1A2=K2K1
Topper's Solved these Questions
Similar Questions
Two metal rods 1 and 2 of the same length have same temperature difference between their ends. Their thermal conductivities are K1andK2 and cross-section areas A1andA2 respectively. What is the required condition for the same rate of heat conduction in them?
View SolutionTwo metal rods 1 and 2 of same lengths have same temperature difference between their ends. Their thermal conductivities are K1 and K2 and cross sectional areas A1 and A2 respectively. If the rate of heat conduction in 1 is four times that in 2, then
View SolutionThe ratio of the areas of cross section of two rods of different materials is 1:2 and the ratio of ther themal conductivities of their mateirals is 4: 3. On keeping equal temperature difference between the ends of theserods, the rate of conduction of heat are equal. The ratio of the lengths of the rods is
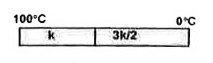
View SolutionTwo rods of similar area of cross section and length are joined as shown in the figure. The ratio of their thermal conductivity is 2:3. The free ends are maintained at 100∘C and 0∘C respectivley. THE temperature at junction in steady state is
View SolutionConsider two rods of same length and different specific heats (S1 and S2), conductivities K1 and K2 and area of cross section (A1 and A2) and both having temperature T1 and T2 at their ends. If the rate of heat loss due to conduction is equal then
View SolutionTwo rods P and Q of equal lengths have thermal conductivities K1andK2 and cross-sectional areas A1andA2 respectively. If the temperature difference across the ends of each rod is the same, then the condition for which the rate of flow of heat through each of them will be equal, is
View SolutionTwo rods A and B are of equal lengths. Their ends of kept between the same temperature and their area of cross-section are A1 and A2 and thermal conductivities K1 and K2. The rate of heat transmission in the two rods will be equal, if
View SolutionIf two liquids of same volume but different densities ρ1 and ρ2 are mixed, then the density of the mixture is:
View SolutionTwo ends of rods of length L and radius R of the same material of kept at the same temperature. Which of the following rods conducts the maximum heat?
View SolutionTwo metal rods of the same length and area of cross-section are fixed end to end between rigid supports. The materials of the rods have Young moduli Y1 and Y2 , and coefficient of linear expansion α1 and α2 . The junction between the rod does not shift and the rods are cooled
View Solution
